Article contents
CONSEQUENCES OF THE EXISTENCE OF AMPLE GENERICS AND AUTOMORPHISM GROUPS OF HOMOGENEOUS METRIC STRUCTURES
Published online by Cambridge University Press: 14 September 2016
Abstract
We define a simple criterion for a homogeneous, complete metric structure X that implies that the automorphism group Aut(X) satisfies all the main consequences of the existence of ample generics: it has the automatic continuity property, the small index property, and uncountable cofinality for nonopen subgroups. Then we verify it for the Urysohn space 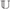 $$, the Lebesgue probability measure algebra MALG, and the Hilbert space
$$, the Lebesgue probability measure algebra MALG, and the Hilbert space 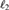 $\ell _2 $, thus proving that Iso(
$\ell _2 $, thus proving that Iso(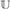 $$), Aut(MALG),
$$), Aut(MALG),  $U\left( {\ell _2 } \right)$, and
$U\left( {\ell _2 } \right)$, and 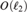 $O\left( {\ell _2 } \right)$ share these properties. We also formulate a condition for X which implies that every homomorphism of Aut(X) into a separable group K with a left-invariant, complete metric, is trivial, and we verify it for
$O\left( {\ell _2 } \right)$ share these properties. We also formulate a condition for X which implies that every homomorphism of Aut(X) into a separable group K with a left-invariant, complete metric, is trivial, and we verify it for 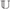 $$, and
$$, and 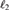 $\ell _2 $.
$\ell _2 $.
Keywords
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2016
References
REFERENCES
- 2
- Cited by


