Article contents
Completeness of global intuitionistic set theory
Published online by Cambridge University Press: 12 March 2014
Extract
Gentzen's sequential system LJ of intuitionistic logic has two symbols of implication. One is the logical symbol → and the other is the metalogical symbol ⇒ in sequents
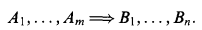
Considering the logical system LJ as a mathematical object, we understand that the logical symbols ∧, ∨, →, ¬, ∀, ∃ are operators on formulas, and ⇒ is a relation. That is, φ ⇒ Ψ is a metalogical sentence which is true or false, on the understanding that our metalogic is a classical logic. In other words, we discuss the logical system LJ in the classical set theory ZFC, in which φ ⇒ Ψ is a sentence.
The aim of this paper is to formulate an intuitionistic set theory together with its metatheory. In Takeuti and Titani [6], we formulated an intuitionistic set theory together with its metatheory based on intuitionistic logic. In this paper we postulate that the metatheory is based on classical logic.
Let Ω be a cHa. Ω can be a truth value set of a model of LJ. Then the logical symbols ∧, ∨, →, ¬, ∀x, ∃x are interpreted as operators on Ω, and the sentence φ ⇒ Ψ is interpreted as 1 (true) or 0 (false). This means that the metalogical symbol ⇒ also can be expressed as a logical operators such that φ ⇒ Ψ is interpreted as 1 or 0.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1997
References
REFERENCES
- 8
- Cited by


