Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T22:15:53.326Z Has data issue: false hasContentIssue false
  • English
  • Français

COMPLETELY SEPARABLE MAD FAMILIES AND THE MODAL LOGIC OF βω

Part of: Set theory General logic Fairly general properties

Published online by Cambridge University Press:  15 June 2020

TOMÁŠ LÁVIČKA  and
JONATHAN L. VERNER
TOMÁŠ LÁVIČKA
Affiliation:
THE CZECH ACADEMY OF SCIENCES INSTITUTE OF INFORMATION THEORY AND AUTOMATION POD VODÁRENSKOU VĚŽÍ 4, 182 07 PRAHA, CZECH REPUBLICE-mail:[email protected]
JONATHAN L. VERNER
Affiliation:
DEPARTMENT OF LOGIC FACULTY OF ARTS CHARLES UNIVERSITY PALACHOVO NÁM. 2, 116 38 PRAHA 1, CZECH REPUBLICE-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

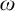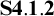We show in ZFC that the existence of completely separable maximal almost disjoint families of subsets of $\omega $ implies that the modal logic $\mathbf {S4.1.2}$ is complete with respect to the Čech–Stone compactification of the natural numbers, the space 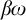$\beta \omega $ . In the same fashion we prove that the modal logic $\mathbf {S4}$ is complete with respect to the space 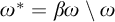$\omega ^*=\beta \omega \setminus \omega $ . This improves the results of G. Bezhanishvili and J. Harding in [4], where the authors prove these theorems under stronger assumptions ( $\mathfrak {a=c}$ ). Our proof is also somewhat simpler.

Keywords

modal logicČech-Stone compactification of natural numberscompletely separable mad families

MSC classification

Primary: 03B45: Modal logic (including the logic of norms)
Secondary: 03E17: Cardinal characteristics of the continuum 54D80: Special constructions of spaces (spaces of ultrafilters, etc.)
Type
Article
Information
The Journal of Symbolic Logic , Volume 87 , Issue 2 , June 2022 , pp. 498 - 507
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aiello, M., van Benthem, J., and Bezhanishvili, G., Reasoning about space: The modal way . Journal of Logic and Computation, vol. 13 (2003), pp. 889–920.CrossRefGoogle Scholar
Balcar, B. and Vojtáš, P., Almost disjoint refinement of families of subsets of $N$ . Proceedings of the American Mathematical Society, vol. 79 (1980), no. 3, pp. 465–470.Google Scholar
Bezhanishvili, G., Bezhanishvili, N., Lucero-Bryan, J., and van Mill, J., S4.3 and hereditarily extremally disconnected spaces . Georgian Mathematical Journal, vol. 22 (2015), no. 4, pp. 469–475.CrossRefGoogle Scholar
Bezhanishvili, G. and Harding, J., The modal logic of $\unicode{x3b2} \mathbb{N}$ . Archive for Mathematical Logic, vol. 48 (2009), no. 3, pp. 231–242.10.1007/s00153-009-0123-9CrossRefGoogle Scholar
Bezhanishvili, G. and Harding, J., Modal logics of stone spaces . Order, vol. 29 (2012), pp. 271–292.10.1007/s11083-011-9224-2CrossRefGoogle Scholar
Bezhanishvili, G., Mines, R., and Morandi, P. J., Scattered, Hausdorff-reducible, and hereditarily irresolvable spaces . Topology and its Applications, vol. 132 (2003), pp. 291–306.CrossRefGoogle Scholar
Gabelaia, D., Modal definability in topology , Master’s thesis, Institute of Logic, Language and Computation, University of Amsterdam, 2001.Google Scholar
Goldblatt, R., Diodorean modality in Minkowski spacetime . Studia Logica, vol. 39 (1980), no. 2–3, pp. 219–236.10.1007/BF00370321CrossRefGoogle Scholar
Hechler, S. H., Classifying almost-disjoint families with applications to $\beta$ N-N . Israel Journal of Mathematics, vol. 10 (1971), no. 4, pp. 413–432.CrossRefGoogle Scholar
Hewitt, E., A problem of set-theoretic topology . Duke Mathematical Journal, vol. 10 (1943), pp. 309–333.CrossRefGoogle Scholar
Hrušák, M., Almost Disjoint Families and Topology, Atlantis Press, Paris, 2014, pp. 601–638.Google Scholar
Hrušák, M. and Simon, P., Completely separable MAD families , Open Problems in Topology. II, Elsevier, Amsterdam, 2007, pp. 179–184.10.1016/B978-044452208-5/50019-3CrossRefGoogle Scholar
Rasiowa, H. and Sikorski, R., The Mathematics of Metamathematics, Monografie matematyczne, Vol. 41, Państwowe Wydawnictwo Naukowe, Poland, 1963.Google Scholar
Shelah, S., MAD saturated families and SANE player . Canadian Journal of Mathematics, vol. 63 (2011), no. 6, pp. 1416–1435.10.4153/CJM-2011-057-1CrossRefGoogle Scholar
Tarski, A., Der Aussagenkalkül und die Topologie . Fundamenta Mathematicae, vol. 31 (1938), pp. 103–134.10.4064/fm-31-1-103-134CrossRefGoogle Scholar
Tarski, A. and McKinsey, J. C. C., The algebra of topology . Annals of Mathematics, vol. 45 (1944), pp. 141—191.Google Scholar
van Benthem, J. and Bezhanishvili, G., Modal logics of space , Handbook of Spatial Logics (Aiello, M., Pratt-Hartmann, I., and Van Benthem, J., editors), Springer Netherlands, Dordrecht, 2007, pp. 217–298.10.1007/978-1-4020-5587-4_5CrossRefGoogle Scholar
van Douwen, E. K., An anti-Hausdorff Fréchet space in which convergent sequences have unique limits . Topology and its Applications, vol. 51 (1993), no. 2, pp. 147–158.10.1016/0166-8641(93)90147-6CrossRefGoogle Scholar
van Mill, J., Bezhanishvili, N., Lucero-Bryan, J., and Bezhanishvili, G., Tychonoff HED-spaces and Zemanian extensions of S4.3 . The Review of Symbolic Logic, vol. 11 (2018), pp. 115–132.Google Scholar