Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T03:33:23.716Z Has data issue: false hasContentIssue false
  • English
  • Français

Categoricity and U-rank in excellent classes

Published online by Cambridge University Press:  12 March 2014

Olivier Lessmann
Olivier Lessmann*
Affiliation:
Mathematical Institute, Oxford University, Oxford, OX1 3LB, England, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 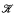be the class of atomic models of a countable first order theory. We prove that if 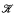is excellent and categorical in some uncountable cardinal, then each model is prime and minimal over the basis of a definable pregeometry given by a quasiminimal set. This implies that 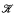is categorical in all uncountable cardinals. We also introduce a U-rank to measure the complexity of complete types over models. We prove that the U-rank has the usual additivity properties, that quasiminimal types have U-rank 1, and that the U-rank of any type is finite in the uncountably categorical, excellent case. However, in contrast to the first order case, the supremum of the U-rank over all types may be ω (and is not achieved). We illustrate the theory with the example of free groups, and Zilber's pseudo analytic structures.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 68 , Issue 4 , December 2003 , pp. 1317 - 1336
Copyright
Copyright © Association for Symbolic Logic 2003

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[BaLa] Baldwin, J. T. and Lachlan, A. H., On strongly minimal sets, this Journal, vol. 36 (1971), pp. 79–96.Google Scholar
[BCM] Baur, Walter, Cherlin, Gregory, and Macintyre, Angus, Totally categorical groups and rings, Journal of Algebra, vol. 57 (1979), pp. 407–440.CrossRefGoogle Scholar
[BuLe] Buechler, Steve and Lessmann, Olivier, Simple homogeneous models, Journal of the American Mathematical Society, vol. 16 (2003), no. 1, pp. 91–121.Google Scholar
[Gr1] Grossberg, Rami, A course on abstract elementary classes, Class notes from a course given at Carnegie Mellon University in 1997.Google Scholar
[Gr2] Grossberg, Rami, Classification theory for abstract elementary classes, Logic and algebra, Contemporary Mathematics, vol. 302, American Mathematical Society, Providence, RI, 2002, pp. 165–204.Google Scholar
[GrHa] Grossberg, Rami and Hart, Bradd, The classification of excellent classes, this Journal, vol. 54 (1989), pp. 1359–1381.Google Scholar
[GrLe] Grossberg, Rami and Lessmann, Olivier, Shelah's stability spectrum and homogeneity spectrum in finite diagrams, Archive for Mathematical Logic, vol. 41 (2002), no. 1, pp. 1–31.Google Scholar
[Hy] Hyttinen, Tapani, Generalizing Morley's theorem, Mathematical Logic Quarterly, vol.44 (1998), pp. 176–184.Google Scholar
[HyLe] Hyttinen, Tapani and Lessmann, Olivier, A rank for the class of elementary submodels of a superstable homogeneous model, this Journal, vol. 67 (2002), no. 4, pp. 1469–1482.Google Scholar
[HyShl] Hyttinen, Tapani and Shelah, Saharon, Strong splitting in stable homogeneous models. Annuls of Pure and Applied Logic, vol. 103 (2000), pp. 201–228.Google Scholar
[HySh2] Hyttinen, Tapani, Main gap for locally saturated elementary submodels of a homogeneous structure, this Journal, vol. 66 (2001), no. 3, pp. 1286–1302.Google Scholar
[Ke] Keisler, H. Jerome, Model theory for infinitary logic, North-Holland Publishing Co., Amsterdam, 1971.Google Scholar
[Ko] Kolesnikov, Alexei, Dependence relations in nonelementary classes . Preprint.Google Scholar
[Le1] Lessmann, Olivier, Ranks and pregeometries in finite diagrams. Annals of Pure and Applied Logic, vol. 106 (2000), pp. 49–83.Google Scholar
[Le2] Lessmann, Olivier, Homogeneous model theory: existence and categoricity, Logic and algebra (Zhang, Yi, editor), Contemporary Mathematics, vol. 302, American Mathematical Society, Providence, RI, 2002, pp. 149–164.Google Scholar
[Ma] Marcus, Leo, A minimal prime model with an infinite set of indiscernibles, Israel Journal of Mathematics, vol. 11 (1972), pp. 180–183.CrossRefGoogle Scholar
[Mo] Morley, Michael, Categoricity in power, Transactions of the American Mathematical Society, vol. 114 (1965), pp. 514–538.Google Scholar
[Sh600] Shelah, Saharon, Preprint.Google Scholar
[Sh576] Shelah, Saharon, Categoricity of an abstract elementary class in two successive cardinals, 115 pages, Preprint.Google Scholar
[Sh3] Shelah, Saharon, Finite diagrams stable in power, Annals of Mathematical Logic, vol. 2 (1970), pp. 69–118.Google Scholar
[Sh70] Shelah, Saharon, Solution to Łoš conjecture for uncountable languages, Notices of the American Mathematical Society, vol. 17 (1970), 968.Google Scholar
[Sh48] Shelah, Saharon, Categoricity in ℵ1 of sentences in L ω1ω (Q), Israel Journal of Mathematics, vol. 20 (1975), pp. 127–148.Google Scholar
[Sh54] Shelah, Saharon, The lazy model-theorist's guide to stability, Proceedings of a symposium in Louvain, March 1975 (Henrand, P., editor), vol. 18, Logique et Analyse, no. 71-72, 1975, pp. 241–308.Google Scholar
[Sh87a] Shelah, Saharon,Classification theory for nonelementary classes. I. The number of uncountable models of ψ ∈ L ω1ω , Part A, Israel Journal of Mathematics, vol. 46 (1983), pp. 212–240.CrossRefGoogle Scholar
[Sh87b] Shelah, Saharon, Classification theory for nonelementary classes, I. The number of uncountable models of ψ ∈ L ω1ω Part B, Classification theory (chicago, il, 1985),Lecture Notes in Mathematics, vol. 1292, Springer, Berlin, 1987, pp. 264–418, Israel Journal of Mathematics , vol. 46 (1983), pp. 241-273, Proceedings of the USA–Israel Conference on Classification Theory, Chicago (J. T. Baldwin, editor), December 1985.Google Scholar
[Zi2] Zilber, Boris, Analytic and pseudo-analytic structures , Preprint.Google Scholar
[Zi1] Zilber, Boris, Covers of the multiplicative group of an algebraically closed field of characteristic 0, Preprint.Google Scholar
[Zi3] Zilber, Boris, Covers of the multiplicative group under ℵ0-categoricity assumption . Preprint.Google Scholar