Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T13:37:22.675Z Has data issue: false hasContentIssue false
  • English
  • Français

BROUWER’S FAN THEOREM AND CONVEXITY

Published online by Cambridge University Press:  21 December 2018

JOSEF BERGER  and
GREGOR SVINDLAND
JOSEF BERGER
Affiliation:
MATHEMATISCHES INSTITUT LUDWIG-MAXIMILIANS-UNIVERSITÄT MÜNCHEN THERESIENSTRASSE 39 80333 MÜNCHEN, GERMANYE-mail:[email protected]
GREGOR SVINDLAND
Affiliation:
MATHEMATISCHES INSTITUT LUDWIG-MAXIMILIANS-UNIVERSITÄT MÜNCHEN THERESIENSTRASSE 39 80333 MÜNCHEN, GERMANYE-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In the framework of Bishop’s constructive mathematics we introduce co-convexity as a property of subsets B of 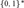${\left\{ {0,1} \right\}^{\rm{*}}}$, the set of finite binary sequences, and prove that co-convex bars are uniform. Moreover, we establish a canonical correspondence between detachable subsets B of 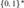${\left\{ {0,1} \right\}^{\rm{*}}}$ and uniformly continuous functions f defined on the unit interval such that B is a bar if and only if the corresponding function f is positive-valued, B is a uniform bar if and only if f has positive infimum, and B is co-convex if and only if f satisfies a weak convexity condition.

Keywords

03F6026E4052A41constructive mathematicsfan theoremconvex functions
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 4 , December 2018 , pp. 1363 - 1375
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Berger, J. and Bridges, D., A fan-theoretic equivalent of the antithesis of Specker’s theorem. Mathematicae, vol. 18 (2007), pp. 195–202.Google Scholar
Berger, J. and Ishihara, H., Brouwer’s fan theorem and unique existence in constructive analysis. Mathematical Logic Quarterly, vol. 51 (2005), pp. 360–364.CrossRefGoogle Scholar
Berger, J. and Svindland, G., Convexity and constructive infima. Archive for Mathematical Logic, vol. 55 (2016), pp. 873–881.CrossRefGoogle Scholar
Bishop, E. and Bridges, D., Constructive Analysis, Springer-Verlag, Heidelberg, 1985.CrossRefGoogle Scholar
Bridges, D. and Richman, F., Varieties of Constructive Mathematics, London Mathematical Society Lecture Notes, vol. 97, Cambridge University Press, New York, 1987.CrossRefGoogle Scholar
Bridges, D. and Vita, L. S., Techniques of Constructive Analysis, Universitext, Springer-Verlag, New York, 2006.Google Scholar
Julian, W. and Richman, F., A uniformly continuous function on $[0,1]$ that is everywhere different from its infimum. Pacific Journal of Mathematics, vol. 111 (1984), pp. 333–340.CrossRefGoogle Scholar