Article contents
Addition in nonstandard models of arithmetic
Published online by Cambridge University Press: 12 March 2014
Extract
In [3] Kemeny made the following conjecture: Suppose *Z is a nonstandard model of the ring of integers Z. Let

and let F be the subgroup of those cosets ā which contain an element of infinite height in *Z. Kemeny then asked if the ring R = {a: ā ∈ F} is also a nonstandard model of Z. If so then Goldbach's conjecture is false because Kemeny also shows in [3] that Goldbach's conjecture fails in R.
The papers [1] and [5] by Gandy and Mendelson show that R is not a nonstandard model of Z but we give here a simpler proof based on Mendelson's paper. Suppose R is a nonstandard model of Z. Then each positive number in R is a sum of four squares. Choose a in R so that a is a positive element of R of infinite height in *Z. Then since a is infinite in *Z, a − 1 is positive. Thus 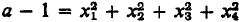 , xi ∈ R for i = 1, …, 4. Now each xi must be of the form ai + ni, where ai has infinite height in *Z and ni, ∈ Z.
, xi ∈ R for i = 1, …, 4. Now each xi must be of the form ai + ni, where ai has infinite height in *Z and ni, ∈ Z.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1972
References
BIBLIOGRAPHY
- 2
- Cited by


