Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-26T13:22:25.205Z Has data issue: false hasContentIssue false
  • English
  • Français

ACCEPTABLE COLORINGS OF INDEXED HYPERSPACES

Published online by Cambridge University Press:  21 December 2018

JAMES H. SCHMERL
JAMES H. SCHMERL*
Affiliation:
DEPARTMENT OF MATHEMATICS UNIVERSITY OF CONNECTICUT STORRS, CT 06269-3009, USAE-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Previous results about n-grids with acceptable colorings are extended here to n-indexed hyperspaces, which are structures 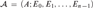${\cal A} = \left( {A;{E_0},{E_1}, \ldots ,{E_{n - 1}}} \right)$, where each ${E_i}$ is an equivalence relation on A.

Keywords

03E5052C99indexed hyperspacegridacceptable coloring
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 4 , December 2018 , pp. 1644 - 1666
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Fundamenta Mathematicae, vol. 38 (1951).Google Scholar
Sierpiński, W., Sur quelques propositions concernant la puissance du continu, in [FM], pp. 1–13.Google Scholar
Kuratowski, C., Sur une charactérisation des alephs, in [FM], pp. 14–17.Google Scholar
Sikorski, R., A characterization of alephs, in [FM], pp. 18–22. [FM]Google Scholar
van den Dries, L., Algebraic theories with definable Skolem functions, this Journal, vol. 49 (1984), pp. 625–629.Google Scholar
Erdős, P., Jackson, S., and Mauldin, R. D., On partitions of lines and space. Fundamenta Mathematicae, vol. 145 (1994), pp. 101–119.Google Scholar
Erdős, P. and Rado, R., A combinatorial theorem. Journal of the London Mathematical Society, vol. 25 (1950), pp. 249–255.CrossRefGoogle Scholar
Graham, R. L., Rothschild, B. L., and Spencer, J. H., Ramsey Theory, Wiley, New York, 1980.Google Scholar
Schmerl, J. H., How many clouds cover the plane? Fundamenta Mathematicae, vol. 177 (2003), pp. 209–211.CrossRefGoogle Scholar
Schmerl, J. H., A generalization of Sierpiński’s paradoxical decompositions: Coloring semialgebraic grids, this Journal, vol. 77 (2012), pp. 1165–1183.Google Scholar
Schmerl, J. H., Deciding the chromatic numbers of algebraic hypergraphs, this Journal, vol. 83 (2018), pp. 128–145.Google Scholar
Sierpiński, W., Sur une propriété des ensembles plans équivalente l’hypothèse du continu. Bulletin de la Société Royale des Sciences de Liège, vol. 20 (1951), pp. 297–299.Google Scholar
Sierpiński, W., Une proposition de la géométrie élémentaire équivalente à l’hypothèse du continu. Comptes rendus de l’Académie des Sciences Paris, vol. 232 (1951), pp. 1046–1047.Google Scholar
Sierpiński, W., Sur une propriété paradoxale de l’espace à trois dimensions équivalente à l’hypothèse du continu. Rendiconti del Circolo Matematico di Palermo, vol. 1 (1952), no. 2, pp. 7–10.CrossRefGoogle Scholar
Simms, J. C., Another characterization of alephs: Decompositions of hyperspace. Notre Dame Journal of Formal Logic, vol. 38 (1997), pp. 19–36.Google Scholar
Simms, J. C., Sierpiński’s theorem. Simon Stevin, vol. 65 (1991), pp. 69–163.Google Scholar
de la Vega, R., Decompositions of the plane and the size of the continuum. Fundamenta Mathematicae, vol. 203 (2009), pp. 65–74.CrossRefGoogle Scholar
de la Vega, R., Coloring grids. Fundamenta Mathematicae, vol. 228 (2015), pp. 283–289.CrossRefGoogle Scholar