Article contents
Wildness implies undecidability for lattices over group rings
Published online by Cambridge University Press: 12 March 2014
Extract
Let G be a finite group. A Z [G]-lattice is a finitely generated Z-torsionfree module over the group ring Z [G]. There is a general conjecture concerning classes of modules over sufficiently recursive rings, and linking wildness and undecidability. Given a finite group G, Z [G] is sufficiently recursive, and our aim here is just to investigate this conjecture for Z [G]-lattices. In this setting, the conjecture says that
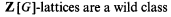
if and only if

In particular, we wish to deal here with the direction from the left to the right, so the one assuring that wildness implies undecidability. Of course, before beginning the analysis of this problem, one should agree upon a sharp definition of wildness for lattices. But, for our purposes, one might alternatively accept as a starting point a general classification of wild Z [G]-lattices when G is a finite p-group for some prime p, based on the isomorphism type of G. This is due to several authors and can be found, for instance, in [3]. It says that, when p is a prime and G is a finite p-group, then

if and only if
 .
.
More precisely, the representation type of Z [G]-lattices is finite when G is cyclic of order ≤ p2, tame domestic when G is the Klein group [1], tame non-domestic when G is cyclic of order 8 [11].
So our claim might be stated as follows.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1997
References
REFERENCES
- 2
- Cited by


