Article contents
Weakly atomic-compact relational structures
Published online by Cambridge University Press: 12 March 2014
Extract
A relational structure 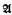 is called weakly atomic-compact if and only if every set Σ of atomic formulas (taken from the first-order language of the similarity type of
is called weakly atomic-compact if and only if every set Σ of atomic formulas (taken from the first-order language of the similarity type of 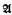 augmented by a possibly uncountable set of additional variables as “unknowns”) is satisfiable in
augmented by a possibly uncountable set of additional variables as “unknowns”) is satisfiable in 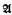 whenever every finite subset of Σ is so satisfiable. This notion (as well as some related ones which will be mentioned in §4) was introduced by J. Mycielski as a generalization to model theory of I. Kaplansky's notion of an algebraically compact Abelian group (cf. [5], [7], [1], [8]).
whenever every finite subset of Σ is so satisfiable. This notion (as well as some related ones which will be mentioned in §4) was introduced by J. Mycielski as a generalization to model theory of I. Kaplansky's notion of an algebraically compact Abelian group (cf. [5], [7], [1], [8]).
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1971
References
- 4
- Cited by


