Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-20T10:39:16.376Z Has data issue: false hasContentIssue false
  • English
  • Français

UNCOUNTABLE TREES AND COHEN 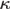$\kappa$-REALS

Published online by Cambridge University Press:  02 July 2019

GIORGIO LAGUZZI
GIORGIO LAGUZZI*
Affiliation:
ALBERT-LUDWIG-UNIVERSITAET-FREIBURG MATHEMATISCHES INSTITUTE ERNST-ZERMELO STR. 1, OFFICE311 79104FREIBURG IM BREISGAU, GERMANY E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate some versions of amoeba for tree-forcings in the generalized Cantor and Baire spaces. This answers [10, Question 3.20] and generalizes a line of research that in the standard case has been studied in [11], [13], and [7]. Moreover, we also answer questions posed in [3] by Friedman, Khomskii, and Kulikov, about the relationships between regularity properties at uncountable cardinals. We show 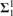${\bf{\Sigma }}_1^1$-counterexamples to some regularity properties related to trees without club splitting. In particular we prove a strong relationship between the Ramsey and the Baire properties, in slight contrast with the standard case.

Keywords

03Exxforcingdescriptive set theory
Type
Articles
Information
The Journal of Symbolic Logic , Volume 84 , Issue 3 , September 2019 , pp. 877 - 894
Copyright
Copyright © The Association for Symbolic Logic 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Brendle, J., How small can the set of generic be? Logic Colloquium ’98 (Buss, S. R., Hájek, P., and Pudlák, P., editors), Lecture Notes in Logic, vol. 13, Cambridge University Press, Cambridge, 2000, pp. 109–126.Google Scholar
Friedman, S. D. and Zdomskyy, L., Measurable cardinals and the cofinality of the symmetric group. Fundamenta Mathematicae, vol. 207 (2010), pp. 101–122.Google Scholar
Friedman, S. D., Khomskii, Y., and Kulikov, V., Regularity properties on the generalized reals. Annals of Pure and Applied Logic, vol. 167 (2016), pp. 408–430.Google Scholar
Halko, A. and Shelah, S., On strong measure zero set in ${2^\kappa }$. Fundamenta Mathematicae, vol. 170 (2001), no. 3, pp. 219–229.Google Scholar
Judah, H. and Roslanowsky, A., On Shelah’s amalgamation. Israel Mathematical Conference Proceedings, vol. 6 (1993), pp. 385–414.Google Scholar
Kanamori, A., Perfect set forcing for uncountable cardinals. Annals of Mathematical Logic, vol. 19 (1980), pp. 97–114.Google Scholar
Laguzzi, G., Some considerations on amoeba forcing notions. Archive for Mathematical Logic, vol. 53 (2014), no. 5–6, pp. 487–502.Google Scholar
Laguzzi, G., On the separation of regularity properties of the reals. Archive for Mathematical Logic, vol. 53 (2014), no. 7–8, pp. 731–747.Google Scholar
Laguzzi, G., Generalized silver and miller measurability. Mathematical Logic Quarterly, vol. 61 (2015), no. 1–2, pp. 91–102.Google Scholar
Khomskii, Y., Laguzzi, G., Löwe, B., and Sharankou, I., Questions on generalized Baire spaces. Mathematical Logic Quarterly, vol. 62 (2016), no. 4–5, pp. 439–456.Google Scholar
Louveau, A., Shelah, S., and Velickovic, B., Borel partitions of infinite subtrees of a perfect tree. Annals of Pure and Applied Logic, vol. 63 (1993), pp. 271–281.Google Scholar
Shelah, S., Can you take Solovay’s inaccessible away? Israel Journal of Mathematics, vol. 48 (1985), pp. 1–47.Google Scholar
Spinas, O., Generic trees, this Journal, vol. 60 (1995), no. 3, pp. 705–726.Google Scholar
Spinas, O., Silver trees and Cohen reals. Israel Journal of Mathematics, vol. 211 (2016), pp. 473–480.Google Scholar