No CrossRef data available.
Article contents
A Spector-Gandy theorem for cPCd(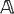 ) classes
) classes
Published online by Cambridge University Press: 12 March 2014
Abstract
Let  be an admissible structure. A cPCd(
be an admissible structure. A cPCd( ) class is the class of all models of a sentence of the form
) class is the class of all models of a sentence of the form 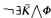 , where
, where  is an
is an  -r.e. set of relation symbols and Φ is an
-r.e. set of relation symbols and Φ is an  -r.e. set of formulas of ℒ∞,ω that are in
-r.e. set of formulas of ℒ∞,ω that are in  . The main theorem is a generalization of the following: Let
. The main theorem is a generalization of the following: Let  be a pure countable resolvable admissible structure such that
be a pure countable resolvable admissible structure such that  is not Σ-elementarily embedded in HYP(
is not Σ-elementarily embedded in HYP( ). Then a class K of countable structures whose universes are sets of urelements is a cPCd(
). Then a class K of countable structures whose universes are sets of urelements is a cPCd( ) class if and only if for some Σ formula σ (with parameters from
) class if and only if for some Σ formula σ (with parameters from  ),
), 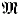 is in K if and only if
is in K if and only if 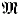 is a countable structure with universe a set of urelements and
is a countable structure with universe a set of urelements and 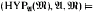 σ, where
σ, where 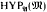 , the smallest admissible set above
, the smallest admissible set above 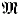 relative to
relative to  , is a generalization of HYP to structures with similarity type Σ over
, is a generalization of HYP to structures with similarity type Σ over  that is defined in this article. Here we just note that when Lα is admissible, HYPLα(
that is defined in this article. Here we just note that when Lα is admissible, HYPLα(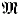 ) is Lβ(
) is Lβ(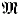 ) for the least β ≥ α such that Lβ(
) for the least β ≥ α such that Lβ(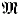 ) is admissible, and so, in particular, that
) is admissible, and so, in particular, that 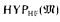 is just HYP(
is just HYP(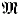 ) in the usual sense when
) in the usual sense when 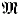 has a finite similarity type.
has a finite similarity type.
The definition of 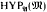 is most naturally formulated using Adamson's notion of a +-admissible structure (1978). We prove a generalization from admissible to +-admissible structures of the well-known truncation lemma. That generalization is a key theorem applied in the proof of the generalized Spector-Gandy theorem.
is most naturally formulated using Adamson's notion of a +-admissible structure (1978). We prove a generalization from admissible to +-admissible structures of the well-known truncation lemma. That generalization is a key theorem applied in the proof of the generalized Spector-Gandy theorem.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1992


