Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-28T18:54:15.491Z Has data issue: false hasContentIssue false
  • English
  • Français

Simplicity, and stability in there

Published online by Cambridge University Press:  12 March 2014

Byunghan Kim
Byunghan Kim*
Affiliation:
Department of Mathematics, 2-171, Massachusetts Institute of Technology, Cambridge, Massachusetts 02139., USA, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

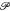Firstly, in this paper, we prove that the equivalence of simplicity and the symmetry of forking. Secondly, we attempt to recover definability part of stability theory to simplicity theory. In particular, using elimination of hyperimaginaries we prove that for any supersimple T. canonical base of an amalgamation class is the union of names of ψ-definitions of 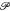, ψ ranging over stationary L-formulas in 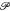. Also, we prove that the same is true with stable formulas for an 1-based theory having elimination of hyperimaginaries. For such a theory, the stable forking property holds, too.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 66 , Issue 2 , June 2001 , pp. 822 - 836
Copyright
Copyright © Association for Symbolic Logic 2001

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Buechler, S., Lascar strong types in some simple theories, this Journal, vol. 64 (1999), pp. 817–824.Google Scholar
[2]Buechler, S., Pillay, A., and Wagner, F. O., Supersimple theories, Journal of the American Mathematical Society, vol. 14 (2000), pp. 109–124.CrossRefGoogle Scholar
[3]Hart, B., Kim, B., and Pillay, A., Coordinatization and canonical bases in simple theories, this Journal, vol. 65 (2000), pp. 293–309.Google Scholar
[4]Kim, B., Simple first order theories, Ph.D. thesis, University of Notre Dame, 1996.Google Scholar
[5]Kim, B., Forking in simple unstable theories, The Journal of the London Mathematical Society, vol. 57 (1998), no. 2, pp. 257–267.CrossRefGoogle Scholar
[6]Kim, B., A note on Lascar strong types in simple theories, this Journal, vol. 63 (1998), pp. 926–936.Google Scholar
[7]Kim, B. and Pillay, A., From stability to simplicity, The Bulletin of Symbolic Logic, vol. 1 (1998), no. 4, pp. 17–36.Google Scholar
[8]Kim, B. and Pillay, A., Simple theories, Annals of Pure and Applied Logic, vol. 88 (1997), pp. 149–164.CrossRefGoogle Scholar
[9]Pillay, A., Definability and definable groups in simple theories, this Journal, vol, 63 (1998), pp. 788–796.Google Scholar
[10]Pillay, A. and Lascar, D., Hyperimaginaries and automorphism groups, this Journal, to appear.Google Scholar
[11]Shami, Z., Definability in low simple theories, this Journal, vol. 65 (2000), pp. 1481–1490.Google Scholar
[12]Shelah, S., Simple unstable theories, Annals of Mathematical Logic, vol. 19 (1980), pp. 177–203.CrossRefGoogle Scholar