No CrossRef data available.
Article contents
Prikry forcing at κ + and beyond
Published online by Cambridge University Press: 12 March 2014
Extract
If U is a normal measure on κ then we can add indiscernibles for U either by Prikry forcing [P] or by taking an iterated ultrapower 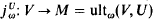 which will add a sequence
which will add a sequence 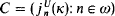 of indiscernibles for
of indiscernibles for 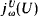 over M. These constructions are equivalent: the set C of indiscernibles for
over M. These constructions are equivalent: the set C of indiscernibles for 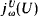 added by the iterated ultrapower is Prikry generic for
added by the iterated ultrapower is Prikry generic for 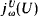 [Mat]. Prikry forcing has been extended for sequences of measures of length
[Mat]. Prikry forcing has been extended for sequences of measures of length  by Magidor [Mag], and his method readily extends to
by Magidor [Mag], and his method readily extends to 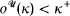 . In this case the measure U is replaced by a sequence
. In this case the measure U is replaced by a sequence 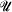 of measures and the set C of indiscernibles is replaced by a system
of measures and the set C of indiscernibles is replaced by a system 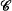 of indiscernibles for
of indiscernibles for 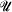 :
: 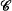 is a function such that
is a function such that 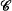 (κ, β) is a set of indiscernibles for
(κ, β) is a set of indiscernibles for 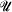 (κ, β) for each
(κ, β) for each 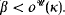 . The equivalence between forcing and iterated ultra-powers still holds true for such sequences: there is an interated ultrapower j: V → M (which is defined in detail later in this paper) such that the system
. The equivalence between forcing and iterated ultra-powers still holds true for such sequences: there is an interated ultrapower j: V → M (which is defined in detail later in this paper) such that the system 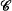 of indiscernibles for j(
of indiscernibles for j(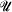 ) constructed by j is Magidor generic over M.
) constructed by j is Magidor generic over M.
The construction of the system 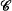 of indiscernibles works equally well for o(κ) ≧ κ +. Radin has defined a variant of Prikry forcing which also works for o(κ) > κ + ([R]; see also [Mi82] where Radin forcing is applied specifically to sequences of measures, rather than to hypermeasures as in Radin's paper), but Radin's forcing is weaker than Magidor's extension of Prikry forcing in the sense that the system
of indiscernibles works equally well for o(κ) ≧ κ +. Radin has defined a variant of Prikry forcing which also works for o(κ) > κ + ([R]; see also [Mi82] where Radin forcing is applied specifically to sequences of measures, rather than to hypermeasures as in Radin's paper), but Radin's forcing is weaker than Magidor's extension of Prikry forcing in the sense that the system 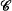 of indiscernibles generated by the interated ultrapower is not Radin generic for j(
of indiscernibles generated by the interated ultrapower is not Radin generic for j(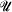 ), but only the set
), but only the set 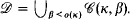 . That is, an indiscernible does not belong to a specific measure, but only to the whole sequence of measures on the cardinal κ.
. That is, an indiscernible does not belong to a specific measure, but only to the whole sequence of measures on the cardinal κ.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1987


