No CrossRef data available.
Published online by Cambridge University Press: 18 July 2022
Recall that B is PA relative to A if B computes a member of every nonempty 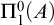 $\Pi ^0_1(A)$ class. This two-place relation is invariant under Turing equivalence and so can be thought of as a binary relation on Turing degrees. Miller and Soskova [23] introduced the notion of a
$\Pi ^0_1(A)$ class. This two-place relation is invariant under Turing equivalence and so can be thought of as a binary relation on Turing degrees. Miller and Soskova [23] introduced the notion of a 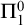 $\Pi ^0_1$ class relative to an enumeration oracle A, which they called a
$\Pi ^0_1$ class relative to an enumeration oracle A, which they called a  $\Pi ^0_1{\left \langle {A}\right \rangle }$ class. We study the induced extension of the relation B is PA relative to A to enumeration oracles and hence enumeration degrees. We isolate several classes of enumeration degrees based on their behavior with respect to this relation: the PA bounded degrees, the degrees that have a universal class, the low for PA degrees, and the
$\Pi ^0_1{\left \langle {A}\right \rangle }$ class. We study the induced extension of the relation B is PA relative to A to enumeration oracles and hence enumeration degrees. We isolate several classes of enumeration degrees based on their behavior with respect to this relation: the PA bounded degrees, the degrees that have a universal class, the low for PA degrees, and the 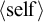 ${\left \langle {\text {self}\kern1pt}\right \rangle }$-PA degrees. We study the relationship between these classes and other known classes of enumeration degrees. We also investigate a group of classes of enumeration degrees that were introduced by Kalimullin and Puzarenko [14] based on properties that are commonly studied in descriptive set theory. As part of this investigation, we give characterizations of three of their classes in terms of a special sub-collection of relativized
${\left \langle {\text {self}\kern1pt}\right \rangle }$-PA degrees. We study the relationship between these classes and other known classes of enumeration degrees. We also investigate a group of classes of enumeration degrees that were introduced by Kalimullin and Puzarenko [14] based on properties that are commonly studied in descriptive set theory. As part of this investigation, we give characterizations of three of their classes in terms of a special sub-collection of relativized  $\Pi ^0_1$ classes—the separating classes. These three can then be seen to be direct analogues of three of our classes. We completely determine the relative position of all classes in question.
$\Pi ^0_1$ classes—the separating classes. These three can then be seen to be direct analogues of three of our classes. We completely determine the relative position of all classes in question.