Article contents
THE MODAL LOGICS OF KRIPKE–FEFERMAN TRUTH
Published online by Cambridge University Press: 27 October 2020
Abstract
We determine the modal logic of fixed-point models of truth and their axiomatizations by Solomon Feferman via Solovay-style completeness results. Given a fixed-point model
 $\mathcal {M}$
, or an axiomatization S thereof, we find a modal logic M such that a modal sentence
$\mathcal {M}$
, or an axiomatization S thereof, we find a modal logic M such that a modal sentence
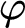 $\varphi $
is a theorem of M if and only if the sentence
$\varphi $
is a theorem of M if and only if the sentence
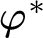 $\varphi ^*$
obtained by translating the modal operator with the truth predicate is true in
$\varphi ^*$
obtained by translating the modal operator with the truth predicate is true in
 $\mathcal {M}$
or a theorem of S under all such translations. To this end, we introduce a novel version of possible worlds semantics featuring both classical and nonclassical worlds and establish the completeness of a family of noncongruent modal logics whose internal logic is nonclassical with respect to this semantics.
$\mathcal {M}$
or a theorem of S under all such translations. To this end, we introduce a novel version of possible worlds semantics featuring both classical and nonclassical worlds and establish the completeness of a family of noncongruent modal logics whose internal logic is nonclassical with respect to this semantics.
Keywords
MSC classification
- Type
- Article
- Information
- Copyright
- © The Association for Symbolic Logic 2020
References
REFERENCES
- 3
- Cited by



