Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-15T01:26:20.175Z Has data issue: false hasContentIssue false
  • English
  • Français

Erdős-Rado without choice

Published online by Cambridge University Press:  12 March 2014

Thomas Forster
Thomas Forster*
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, Centre for Mathematical Sciences, Wilberforce Road, Cambridge, CB3 OWB., UK. E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A version of the Erdős-Rado theorem on partitions of the unordered n-tuples from uncountable sets is proved, without using the axiom of choice. The case with exponent 1 is just the Sierpinski-Hartogs' result that .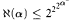

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 72 , Issue 3 , September 2007 , pp. 897 - 900
Copyright
Copyright © Association for Symbolic Logic 2007

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Erdős, P., Some set-theoretical properties of graphs. Revista Universidad Nacional de Tucuman, Serie A, vol. 3 (1942), pp. 363–367.Google Scholar
[2] Erdős, P. and Rado, R., A partition calculus in set theory, The Bulletin of the American Mathematical Society, (1956), pp. 427–498.Google Scholar