Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-28T15:36:47.032Z Has data issue: false hasContentIssue false
  • English
  • Français

Dual easy uniformization and model-theoretic descriptive set theory

Published online by Cambridge University Press:  12 March 2014

Shaughan Lavine
Shaughan Lavine*
Affiliation:
Department of Philosophy, Columbia University, New York, New York 10027
Get access Rights & Permissions [Opens in a new window]

Abstract

It is well known that, in the terminology of Moschovakis, Descriptive set theory (1980), every adequate normed pointclass closed under ∀ω has an effective version of the generalized reduction property (GRP) called the easy uniformization property (EUP). We prove a dual result: every adequate normed pointclass closed under ∃ω has the EUP. Moschovakis was concerned with the descriptive set theory of subsets of Polish topological spaces. We set up a general framework for parts of descriptive set theory and prove results that have as special cases not only the just-mentioned topological results, but also corresponding results concerning the descriptive set theory of classes of classes of structures.

Vaught (1973) asked whether the class of cPCδ classes of countable structures has the GRP. It does. A cPC(A) class is the class of all models of a sentence of the form 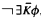, where ϕ is a sentence of ℒ∞ω that is in A and 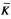is a set of relation symbols that is in A. Vaught also asked whether there is any primitive recursively closed set A such that some effective version of the GRP holds for the class of cPC(A) classes of countable structures. There is: The class of cPC(A) classes of countable structures has the EUP if ω ∈ A and A is countable and primitive recursively closed. Those results and some extensions are obtained by first showing that the relevant classes of classes of structures, which Vaught showed normed, are in a suitable sense adequate and closed under ∃ω, and then applying the dual easy uniformization theorem.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 56 , Issue 4 , December 1991 , pp. 1290 - 1316
Copyright
Copyright © Association for Symbolic Logic 1991

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[Bar75]Barwise, Jon, Admissible sets and structures, Perspectives in Mathematical Logic, Springer-Verlag, Berlin, 1975.CrossRefGoogle Scholar
[BM75]Burgess, John and Miller, Douglas, Remarks on invariant descriptive set theory, Fundamenta Mathematicae, vol. 90 (1975), pp. 53–75.CrossRefGoogle Scholar
[CK73]Chang, C. C. and Keisler, H. J., Model theory, Studies in Logic and the Foundations of Mathematics, vol. 73, North-Holland, Amsterdam, 1973.Google Scholar
[Kre62]Kreisel, G., The axiom of choice and the class of hyperarithmetic functions, Indagationes Mathematicae, vol. 24 (1962), pp. 307–319.CrossRefGoogle Scholar
[Kur36]Kuratowski, K., Sur les théorèmes de séparation dans la théorie des ensembles, Fundamenta Mathematicae, vol. 26 (1936), pp. 183–191.CrossRefGoogle Scholar
[Kur66]Kuratowski, K., Topology, Vol. 1, Academic Press, New York, 1966.Google Scholar
[Lav84]Lavine, Shaughan, Generalized reduction for complements of PCδ classes, Abstracts of Papers Presented to the American Mathematical Society, vol. 5 (1984), p. 388.Google Scholar
[Lav88]Lavine, Shaughan, [“Lavine, Michael A.”], Spector-Gandy and generalized reduction theorems for modeltheoretic analogs of the class of coanalytic sets, Ph.D. thesis, University of California, Berkeley, California, 1988.Google Scholar
[Mak64]Makkai, M., On PC⊿-classes in the theory of models, A Magyar Tudományos Akadémia Matematikai Kutató Intézetének Közleményei (Budapest), vol. 9 (1964), pp. 159–194.Google Scholar
[Mal59]Mal'cev, A. I., Model correspondences, Izvestiya Akademiya Nauk SSSR Seriya Matematicheskaya, vol. 23 (1959), pp. 313–336; English translation, Chapter XI in A. I. Mal'cev, The meta-mathematics of algebraic systems. Collected papers: 1936-1967, Studies in Logic and the Foundations of Mathematics, vol. 66, North-Holland, Amsterdam, 1971, pp. 66-94. See also E. Mendelson, Mathematical Reviews, vol. 22 (1961), no. 10909.Google Scholar
[Mil76]Miller, Douglas, Invariant descriptive set theory and the topological approach to model theory, Ph.D. thesis, University of California, Berkeley, California, 1976.Google Scholar
[Mos80]Moschovakis, Yiannis Nicholas, Descriptive set theory, Studies in Logic and the Foundations of Mathematics, vol. 100, North-Holland, Amsterdam, 1980.Google Scholar
[Vau73]Vaught, Robert, Descriptive set theory in Lω1ω, Cambridge summer school in mathematical logic (Mathias, A. R. D. and Rogers, H., editors), Lecture Notes in Mathematics, vol. 337, Springer-Verlag, Berlin, 1973, pp. 574–598.CrossRefGoogle Scholar
[Vau80]Vaught, Robert, On PCd(A)-classes for an admissible set A, Mathematical logic in Latin America (Arruda, A. I.et al., editors), Studies in Logic and the Foundations of Mathematics, vol. 99, North-Holland, Amsterdam, 1980, pp. 377–392.Google Scholar