No CrossRef data available.
Article contents
Definability and initial segments of c-degrees
Published online by Cambridge University Press: 12 March 2014
Abstract
We combine two techniques of set theory relating to mininal degrees of constructibility. Jensen constructed a minimal real which is additionally a 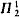 singleton. Groszek built an initial segment of order type 1 + α*, for any ordinal α. This paper shows how to force a
singleton. Groszek built an initial segment of order type 1 + α*, for any ordinal α. This paper shows how to force a 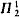 singleton such that the c-degrees beneath it, all represented by reals, are of type 1 + α*, for many ordinals α. We also examine the definability α needs to be so represented by a real.
singleton such that the c-degrees beneath it, all represented by reals, are of type 1 + α*, for many ordinals α. We also examine the definability α needs to be so represented by a real.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1988
References
REFERENCE
[A]
Adamowicz, Zofia, Constructible semi-lattices of degrees of constructibility, Set theory and hierarchy theory. V (Lachlan, A.
et al., editors), Lecture Notes in Mathematics, vol. 619, Springer-Verlag, Berlin, 1977, pp. 1–43.CrossRefGoogle Scholar
[J]
Jensen, Ronald, Definable sets of minimal degree, Mathematical logic and foundations of set theory (Bar-Hillel, Y., editor), North-Holland, Amsterdam, 1970, pp. 122–128.Google Scholar
[Lu]
Lubarsky, Robert, Lattices of c-degrees, Annals of Pure and Applied Logic, vol. 36 (1987), pp. 115–118.CrossRefGoogle Scholar
[Sa]
Sacks, Gerald E., Countable admissible ordinals and hyperdegrees, Advances in Mathematics, vol. 20 (1976), pp. 213–262.CrossRefGoogle Scholar
[Sa1]
Sacks, Gerald E., Forcing with perfect closed sets, Axiomatic set theory (Scott, D. S., editor), Proceedings of Symposia in Pure Mathematics, vol. 13, part 1, American Mathematical Society, Providence, Rhode Island, 1971, pp. 331–355.CrossRefGoogle Scholar


