No CrossRef data available.
Article contents
BASIS THEOREMS FOR 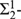 ${\rm{\Sigma }}_2^1$-SETS
${\rm{\Sigma }}_2^1$-SETS
Published online by Cambridge University Press: 11 February 2019
Abstract
We prove the following two basis theorems for 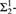 ${\rm{\Sigma }}_2^1$-sets of reals:
${\rm{\Sigma }}_2^1$-sets of reals:
(1) Every nonthin
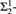 ${\rm{\Sigma }}_2^1$-set has a perfect
${\rm{\Sigma }}_2^1$-set has a perfect 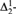 ${\rm{\Delta }}_2^1$-subset if and only if it has a nonthin
${\rm{\Delta }}_2^1$-subset if and only if it has a nonthin  ${\rm{\Delta }}_2^1$-subset, and this is equivalent to the statement that there is a nonconstructible real.
${\rm{\Delta }}_2^1$-subset, and this is equivalent to the statement that there is a nonconstructible real.(2) Every uncountable
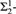 ${\rm{\Sigma }}_2^1$-set has an uncountable
${\rm{\Sigma }}_2^1$-set has an uncountable 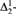 ${\rm{\Delta }}_2^1$-subset if and only if either every real is constructible or
${\rm{\Delta }}_2^1$-subset if and only if either every real is constructible or 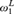 $\omega _1^L$ is countable.
$\omega _1^L$ is countable.
We also apply the method that proves (2) to show that if there is a nonconstructible real, then there is a perfect 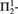 ${\rm{\Pi }}_2^1$-set with no nonempty
${\rm{\Pi }}_2^1$-set with no nonempty 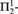 ${\rm{\Pi }}_2^1$-thin subset, strengthening a result of Harrington [4].
${\rm{\Pi }}_2^1$-thin subset, strengthening a result of Harrington [4].
Keywords
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2019


