Article contents
An algebraic result about soft model theoretical equivalence relations with an application to H. Friedman's fourth problem
Published online by Cambridge University Press: 12 March 2014
Abstract
We prove the following algebraic characterization of elementary equivalence: ≡ restricted to countable structures of finite type is minimal among the equivalence relations, other than isomorphism, which are preserved under reduct and renaming and which have the Robinson property; the latter is a faithful adaptation for equivalence relations of the familiar model theoretical notion. We apply this result to Friedman's fourth problem by proving that if 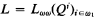 is an (ω1, ω)-compact logic satisfying both the Robinson consistency theorem on countable structures of finite type and the Löwenheim-Skolem theorem for some λ < ωω for theories having ω1 many sentences, then ≡L = ≡ on such structures.
is an (ω1, ω)-compact logic satisfying both the Robinson consistency theorem on countable structures of finite type and the Löwenheim-Skolem theorem for some λ < ωω for theories having ω1 many sentences, then ≡L = ≡ on such structures.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1981
References
REFERENCES
- 3
- Cited by


