Article contents
Amalgamations preserving ℵ1-categoricity
Published online by Cambridge University Press: 12 March 2014
Extract
Let L0, L1 and L2 be countable languages with L ∩ L1 = L0. Let M0 be an L0-structure and Mi, an expansion of M0 to an Li,-structure (i = 1,2). We will call an L1 ∪ L2-structure M an amalgamation of M1 and M2 if M∣Li ≅ Mi, (i = 1,2). Let's consider the following problem.
(*) Suppose that both M1 and M2 belong to the class 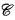 . Can we always find an amalgamation M in
. Can we always find an amalgamation M in 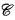 ?
?
Of course the existence of such an amalgamation depends on the class L. Some examples of 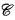 and the answers are given below.
and the answers are given below.
1. 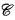 = Countably saturated strongly minimal structures with the DMP In [3], Hrushovski showed that any two strongly minimal theories formulated in totally different languages have a common extension which is still strongly minimal and with the DMP (DMP is the property that states that if a point
= Countably saturated strongly minimal structures with the DMP In [3], Hrushovski showed that any two strongly minimal theories formulated in totally different languages have a common extension which is still strongly minimal and with the DMP (DMP is the property that states that if a point 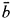 is sufficiently close to ā, then φ(
is sufficiently close to ā, then φ(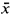 ,
, 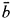 ) has the same rank and the same degree as φ(
) has the same rank and the same degree as φ(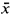 , ā).) His proof essentially shows that if L0 = ∅ then any two countably saturated strongly minimal structures with the DMP have a strongly minimal amalgamation. Also he gave an example that shows the condition L0 = ∅ is necessary.
, ā).) His proof essentially shows that if L0 = ∅ then any two countably saturated strongly minimal structures with the DMP have a strongly minimal amalgamation. Also he gave an example that shows the condition L0 = ∅ is necessary.
2. 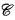 = ℵ1-categorical countable structures. Let M1 be the structure (ℚ, +) and let M2 be the {E, F}-structure defined by: (i) E is an equivalence relation which divides the universe into two infinite classes A and B, (ii) F is a bijection between A and B.
= ℵ1-categorical countable structures. Let M1 be the structure (ℚ, +) and let M2 be the {E, F}-structure defined by: (i) E is an equivalence relation which divides the universe into two infinite classes A and B, (ii) F is a bijection between A and B.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1997
References
REFERENCES
- 2
- Cited by


