Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-29T04:29:48.084Z Has data issue: false hasContentIssue false
  • English
  • Français

Modulationally stable envelope solitons in astrophysical magnetoplasmas with degenerate relativistic electrons

Published online by Cambridge University Press:  28 October 2015

M. Irfan ,
S. Ali ,
Arshad M. Mirza  and
Yunliang Wang
M. Irfan*
Affiliation:
Theoretical Plasma Physics Group, Department of Physics, Quaid-i-Azam University, Islamabad 45320, Pakistan National Centre for Physics at QAU Campus, Shahdra Valley Road, Islamabad 44000, Pakistan
S. Ali
Affiliation:
National Centre for Physics at QAU Campus, Shahdra Valley Road, Islamabad 44000, Pakistan
Arshad M. Mirza
Affiliation:
Theoretical Plasma Physics Group, Department of Physics, Quaid-i-Azam University, Islamabad 45320, Pakistan
Yunliang Wang
Affiliation:
Department of Physics, School of Mathematics and Physics, University of Science and Technology Beijing, Beijing 100083, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The formation and propagation characteristics of small-amplitude magnetoacoustic dark/grey solitons are investigated in a semi relativistic degenerate magnetoplasma whose constituents are electrons and singly ionized positive ions. For this purpose, the electrons are assumed to follow the degeneracy pressure law through the Chandrasekhar equation of state, while the inertial cold ions are taken as non-degenerate and magnetized. By solving the one-fluid quantum magnetohydrodynamic (QMHD) model with the aid of a reductive perturbation technique, a nonlinear Schrödinger (NLS) equation is derived for weakly nonlinear envelope magnetoacoustic solitons. The NLS equation admits the existence of stable excitations, e.g. dark and grey solitons for which the condition $P/Q<0$ holds. Numerical results reveal that the variation of plasma number density, magnetic field strength, relativistic parameter 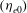$({\it\eta}_{e0})$ and the quantum parameter $(H)$ significantly modify the profiles of the envelope magnetoacoustic solitons. The present results are important to understanding of the nonlinear dynamics of magnetoacoustic solitons in astrophysical dense magnetoplasmas (viz., white dwarfs, magnetars, neutron stars, etc.), where the relativistic degeneracy effects play a vital role in collective interactions.

Type
Research Article
Information
Journal of Plasma Physics , Volume 81 , Issue 6 , December 2015 , 905810604
Copyright
© Cambridge University Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adlam, J. H. & Allen, J. E. 1958 The structure of strong collision-free hydromagnetic waves. Phil. Mag. 3, 448.Google Scholar
Agrawal, G. P. 1995 Nonlinear Fiber Optics. Academic.Google Scholar
Amin, M. R., Morfill, G. E. & Shukla, P. K. 1998 Modulational instability of dust-acoustic waves. Phys. Rev. E 58, 6517.CrossRefGoogle Scholar
Asano, N., Taniuti, T. & Yajima, N. 1969 Perturbation method for a nonlinear wave modulation. II. J. Math. Phys 10, 2020–2024.Google Scholar
Bains, A. S., Tribeche, M. & Gill, T. S. 2011 Modulational instability of electron-acoustic waves in a plasma with a q-nonextensive electron velocity distribution. Phys. Lett. A 375, 2059–2063.Google Scholar
Burger, S., Bongs, K., Dettmer, S., Ertmer, W. & Sengstock, K. 1999 Dark solitons in Bose–Einstein condensates. Phys. Rev. Lett. 83, 5198.Google Scholar
Chabrier, G., Douchin, F. & Potekhin, A. Y. 2002 Dense astrophysical plasmas. J. Phys.: Condens. Matter 14, 9133.Google Scholar
Chabchoub, A., Kimmoun, O., Branger, H., Hoffmann, N., Proment, D., Onorato, M. & Akhmediev, N. 2013 Experimental observation of dark solitons on water surface. Phys. Rev. Lett. 110, 124101.Google Scholar
Chandrasekhar, S. 1935a Stellar configurations with degenerate cores. Mon. Not. R. Astron. Soc. 95, 226.Google Scholar
Chandrasekhar, S. 1935b Mon. Not. R. Astron. Soc. 170, 405.Google Scholar
Chandrasekhar, S. 1931a The density of white dwarf stars. Phil. Mag. 11, 592.Google Scholar
Chandrasekhar, S. 1931b The maximum mass of ideal white dwarfs. Astrophys. J. 74, 81.Google Scholar
Chen, F. F. 1983 Introduction to Plasma Physics and Controlled Fusion. Plenum.Google Scholar
Crouseilles, N., Hervieux, P.-A. & Manfredi, G. 2008 Quantum hydrodynamic model for the nonlinear electron dynamics in thin metal films. Phys. Rev. B 78, 155412.Google Scholar
Fedele, R., Schamel, H. & Shukla, P. K. 2002 Solitons in the Madelung’s fluid. Phys. Scr. T 98, 18–23.Google Scholar
Fedele, R. & Schamel, H. 2002 Solitary waves in the Madelung’s fluid: Connection between the nonlinear Schrödinger equation and the Korteweg–de Vries equation. Eur. Phys. J. B 27, 313–320.CrossRefGoogle Scholar
García-Berro, E., Torres, S., Althaus, L. G., Renedo, I., Lorén-Aguilar, P., Córsico, A. H., Rohrmann, R. D., Salaris, M. & Isern, J. 2010 A white dwarf cooling age of 8 Gyr for NGC 6791 from physical separation processes. Nature 465, 194–196.CrossRefGoogle Scholar
Haas, F. 2005 A magnetohydrodynamic model for quantum plasmas. Phys. Plasmas 12, 062117.CrossRefGoogle Scholar
Hussain, S., Mushtaq, A. & Mahmood, S. 2013 An arbitrary amplitude fast magnetosonic soliton in quantum electron–positron–ion plasmas. Phys. Scr. 87, 025502.CrossRefGoogle Scholar
Kakutani, T. & Ono, H. 1969 Weak non-linear hydromagnetic waves in a cold collision-free plasma. J. Phys. Soc. Japan. 26, 1305–1318.Google Scholar
Kalinikos, B. A., Scott, M. M. & Patton, C. E. 2000 Self-generation of fundamental dark solitons in magnetic films. Phys. Rev. Lett. 84, 4697.Google Scholar
Kawahara, T. 1973 The derivative expansion method and nonlinear dispersive waves. J. Phys. Soc. Japan. 35, 1537–1544.CrossRefGoogle Scholar
Koester, D. & Chanmugam, G. 1990 Physics of white dwarf stars. Rep. Prog. Phys. 53, 837–915.Google Scholar
Kourakis, I. & Shukla, P. K. 2004 Finite ion temperature effects on oblique modulational stability and envelope excitations of dust-ion acoustic waves. Eur. Phys. J. D 28, 109–117.Google Scholar
Kourakis, I., Mc kerr, M. & Rahman, A. 2013 Semiclassical relativistic fluid theory for electrostatic envelope modes in dense electron–positron–ion plasmas: Modulational instability and rogue waves. J. Plasma Phys. 79, 1089–1094.Google Scholar
Lonngren, K. & Scott, A. 1978 Solitons in Action. Academic.Google Scholar
Mahmood, S., Sadiq, S. & Haque, Q. 2013 Nonlinear electrostatic excitations in magnetized dense plasmas with non-relativistic and ultra-relativistic degenerate electrons. Phys. Plasmas 20, 122305.Google Scholar
Manfredi, G. 2005 How to model quantum plasmas. Fields Inst. Commun. 46, 263–287.Google Scholar
Markowich, P. A., Ringhofer, C. & Schmeiser, C. 1990 Semiconductor Equations. Springer.Google Scholar
Masood, W. & Eliasson, B. 2011 Electrostatic solitary waves in a quantum plasma with relativistically degenerate electrons. Phys. Plasmas 18, 034503.CrossRefGoogle Scholar
Mc Kerr, M., Kourakis, I. & Hass, F. 2014 Freak waves and electrostatic wavepacket modulation in a quantum electron–positron–ion plasma. Plasma Phys. Control. Fusion 56, 035007.Google Scholar
Misra, A. P. 2014 Modulation of drift-wave envelopes in a nonuniform quantum magnetoplasma. Phys. Plasmas 21, 042306.CrossRefGoogle Scholar
Mushtaq, A. & Vladimirov, S. V. 2010 Fast and slow magnetosonic waves in two-dimensional spin-1/2 quantum plasma. Phys. Plasmas 17, 102310.CrossRefGoogle Scholar
Rahman, A., Mc Kerr, M., El-Taibany, W. F., Kourakis, I. & Qamar, A. 2015 Amplitude modulation of quantum-ion-acoustic wavepackets in electron–positron–ion plasmas: Modulational instability, envelope modes, extreme waves. Phys. Plasmas 22, 022305.CrossRefGoogle Scholar
Remoissenet, M. 1999 Waves Called Solitons: Concepts and Experiments. Springer.Google Scholar
Ren, H., Wu, Z., Cao, J. & Chu, P. K. 2009 Electrostatic drift modes in quantum dusty plasmas with Jeans terms. Phys. Plasmas 16, 103705.Google Scholar
Sabry, R., Moslem, W. M., Shukla, P. K. & Saleem, H. 2009 Cylindrical and spherical ion-acoustic envelope solitons in multicomponent plasmas with positrons. Phys. Rev. E 79, 056402.Google Scholar
Salimullah, M., Jamil, M., Shah, H. A. & Murtaza, G. 2009 Jeans instability in a quantum dusty magnetoplasma. Phys. Plasmas 16, 014502.CrossRefGoogle Scholar
Scott, M. M., Kostylev, M. P., Kalinikos, B. A. & Patton, C. E. 2005 Excitation of bright and dark envelope solitons for magnetostatic waves with attractive nonlinearity. Phys. Rev. B 71, 174440.Google Scholar
Serbeto, A., Mendonca, J. T., Tsui, K. H. & Bonifacio, R. 2008 Quantum wave kinetics of high-gain free-electron lasers. Phys. Plasmas 15, 013110.CrossRefGoogle Scholar
Shaikh, D. & Shukla, P. K. 2007 Fluid turbulence in quantum plasmas. Phys. Rev. Lett. 99, 125002.Google Scholar
Shukla, P. K., Eliasson, B. & Stenflo, L. 2011 Dark and grey compressional dispersive Alfvén solitons in plasmas. Phys. Plasmas 18, 064511.Google Scholar
Stockman, M. I. 2011 Nanoplasmonics: The physics behind the applications. Phys. Today 64, 39.Google Scholar
Taniuti, T. & Yajima, N. 1969 Perturbation method for a nonlinear wave modulation. J. Math. Phys. 10, 1369–1372.Google Scholar
Wang, Y., Lü, X. & Eliasson, B. 2013a Modulational instability of spin modified quantum magnetosonic waves in Fermi–Dirac–Pauli plasmas. Phys. Plasmas 20, 112115.Google Scholar
Wang, Y. Y., Li, J. T., Dai, C. Q., Chen, X. F. & Zhang, J. F. 2013b Solitary waves and rogue waves in a plasma with nonthermal electrons featuring Tsallis distribution. Phys. Lett. A 377, 2097–2104.Google Scholar
Wu, Z., Ren, H., Cao, J. & Chu, P. K. 2008 Electrostatic drift waves in nonuniform quantum magnetized plasmas. Phys. Plasmas 15, 082103.Google Scholar