Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chandran, Benjamin D. G.
and
Perez, Jean C.
2019.
Reflection-driven magnetohydrodynamic turbulence in the solar atmosphere and solar wind.
Journal of Plasma Physics,
Vol. 85,
Issue. 4,
Rincon, François
2019.
Dynamo theories.
Journal of Plasma Physics,
Vol. 85,
Issue. 4,
Kingsland, Matthew
Yang, H.-Y. Karen
Reynolds, Christopher S.
and
Zuhone, John A.
2019.
Effects of Anisotropic Viscosity on the Evolution of Active Galactic Nuclei Bubbles in Galaxy Clusters.
The Astrophysical Journal Letters,
Vol. 883,
Issue. 1,
p.
L23.
Zhuravleva, I.
Churazov, E.
Schekochihin, A. A.
Allen, S. W.
Vikhlinin, A.
and
Werner, N.
2019.
Suppressed effective viscosity in the bulk intergalactic plasma.
Nature Astronomy,
Vol. 3,
Issue. 9,
p.
832.
Kempski, Philipp
Quataert, Eliot
Squire, Jonathan
and
Kunz, Matthew W
2019.
Shearing-box simulations of MRI-driven turbulence in weakly collisional accretion discs.
Monthly Notices of the Royal Astronomical Society,
Vol. 486,
Issue. 3,
p.
4013.
Berlok, Thomas
Pakmor, Rüdiger
and
Pfrommer, Christoph
2020.
Braginskii viscosity on an unstructured, moving mesh accelerated with super-time-stepping.
Monthly Notices of the Royal Astronomical Society,
Vol. 491,
Issue. 2,
p.
2919.
St-Onge, D. A.
Kunz, M. W.
Squire, J.
and
Schekochihin, A. A.
2020.
Fluctuation dynamo in a weakly collisional plasma.
Journal of Plasma Physics,
Vol. 86,
Issue. 5,
Li, Yuan
Gendron-Marsolais, Marie-Lou
Zhuravleva, Irina
Xu, Siyao
Simionescu, Aurora
Tremblay, Grant R.
Lochhaas, Cassandra
Bryan, Greg L.
Quataert, Eliot
Murray, Norman W.
Boselli, Alessandro
Hlavacek-Larrondo, Julie
Zheng, Yong
Fossati, Matteo
Li, Miao
Emsellem, Eric
Sarzi, Marc
Arzamasskiy, Lev
and
Vishniac, Ethan T.
2020.
Direct Detection of Black Hole-driven Turbulence in the Centers of Galaxy Clusters.
The Astrophysical Journal Letters,
Vol. 889,
Issue. 1,
p.
L1.
Chen, C. H. K.
Bale, S. D.
Bonnell, J. W.
Borovikov, D.
Bowen, T. A.
Burgess, D.
Case, A. W.
Chandran, B. D. G.
de Wit, T. Dudok
Goetz, K.
Harvey, P. R.
Kasper, J. C.
Klein, K. G.
Korreck, K. E.
Larson, D.
Livi, R.
MacDowall, R. J.
Malaspina, D. M.
Mallet, A.
McManus, M. D.
Moncuquet, M.
Pulupa, M.
Stevens, M. L.
and
Whittlesey, P.
2020.
The Evolution and Role of Solar Wind Turbulence in the Inner Heliosphere.
The Astrophysical Journal Supplement Series,
Vol. 246,
Issue. 2,
p.
53.
Tenerani, Anna
and
Velli, Marco
2020.
Alfvénic fluctuations in the solar wind: nonlinearities and pressure anisotropy effects.
Plasma Physics and Controlled Fusion,
Vol. 62,
Issue. 1,
p.
014001.
Zhdankin, Vladimir
Uzdensky, Dmitri A
Werner, Gregory R
and
Begelman, Mitchell C
2020.
Kinetic turbulence in shining pair plasma: intermittent beaming and thermalization by radiative cooling.
Monthly Notices of the Royal Astronomical Society,
Vol. 493,
Issue. 1,
p.
603.
Squire, J.
Chandran, B. D. G.
and
Meyrand, R.
2020.
In-situ Switchback Formation in the Expanding Solar Wind.
The Astrophysical Journal Letters,
Vol. 891,
Issue. 1,
p.
L2.
Li, Zhihui
Hopkins, Philip F
Squire, Jonathan
and
Hummels, Cameron
2020.
On the survival of cool clouds in the circumgalactic medium.
Monthly Notices of the Royal Astronomical Society,
Vol. 492,
Issue. 2,
p.
1841.
Berlok, Thomas
Quataert, Eliot
Pessah, Martin E
and
Pfrommer, Christoph
2021.
Suppressed heat conductivity in the intracluster medium: implications for the magneto-thermal instability.
Monthly Notices of the Royal Astronomical Society,
Vol. 504,
Issue. 3,
p.
3435.
Schekochihin, Alexander A.
2022.
MHD turbulence: a biased review.
Journal of Plasma Physics,
Vol. 88,
Issue. 5,
Kunz, Matthew W.
Jones, Thomas W.
and
Zhuravleva, Irina
2022.
Handbook of X-ray and Gamma-ray Astrophysics.
p.
1.
Kempf, J. M.
Rincon, F.
and
Clerc, N.
2023.
Dynamical properties and detectability of the magneto-thermal instability in the intracluster medium.
Astronomy & Astrophysics,
Vol. 680,
Issue. ,
p.
A24.
Squire, J.
Kunz, M.W.
Arzamasskiy, L.
Johnston, Z.
Quataert, E.
and
Schekochihin, A.A.
2023.
Pressure anisotropy and viscous heating in weakly collisional plasma turbulence.
Journal of Plasma Physics,
Vol. 89,
Issue. 4,
Arzamasskiy, Lev
Kunz, Matthew W.
Squire, Jonathan
Quataert, Eliot
and
Schekochihin, Alexander A.
2023.
Kinetic Turbulence in Collisionless High-
β
Plasmas.
Physical Review X,
Vol. 13,
Issue. 2,
Ignesti, Alessandro
Brunetti, Gianfranco
Gullieuszik, Marco
Akerman, Nina
Marasco, Antonino
Poggianti, Bianca M.
Li, Yuan
Vulcani, Benedetta
Gitti, Myriam
Moretti, Alessia
Giunchi, Eric
Tomičić, Neven
Bacchini, Cecilia
Paladino, Rosita
Radovich, Mario
and
Wolter, Anna
2024.
Investigating the Intracluster Medium Viscosity Using the Tails of GASP Jellyfish Galaxies.
The Astrophysical Journal,
Vol. 977,
Issue. 2,
p.
219.
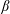 $\unicode[STIX]{x1D6FD}$) plasmas its dynamical relevance is similar to that of incompressibility. Simulations of magnetized turbulence using the weakly collisional Braginskii model show that magneto-immutable turbulence is surprisingly similar, in most statistical measures, to critically balanced magnetohydrodynamic turbulence. However, in order to minimize magnetic-field variation, the flow direction becomes more constrained than in magnetohydrodynamics, and the turbulence is more strongly dominated by magnetic energy (a non-zero ‘residual energy’). These effects represent key differences between pressure-anisotropic and fluid turbulence, and should be observable in the
$\unicode[STIX]{x1D6FD}$) plasmas its dynamical relevance is similar to that of incompressibility. Simulations of magnetized turbulence using the weakly collisional Braginskii model show that magneto-immutable turbulence is surprisingly similar, in most statistical measures, to critically balanced magnetohydrodynamic turbulence. However, in order to minimize magnetic-field variation, the flow direction becomes more constrained than in magnetohydrodynamics, and the turbulence is more strongly dominated by magnetic energy (a non-zero ‘residual energy’). These effects represent key differences between pressure-anisotropic and fluid turbulence, and should be observable in the 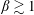 $\unicode[STIX]{x1D6FD}\gtrsim 1$ turbulent solar wind.
$\unicode[STIX]{x1D6FD}\gtrsim 1$ turbulent solar wind.

