Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-25T05:27:20.624Z Has data issue: false hasContentIssue false
  • English
  • Français

Equilibrium 𝛽-limits in classical stellarators

Part of: Focus on Fusion Frontiers in Plasma Physics Conference

Published online by Cambridge University Press:  17 November 2017

J. Loizu ,
S. R. Hudson ,
C. Nührenberg ,
J. Geiger  and
P. Helander
J. Loizu*
Affiliation:
Max-Planck-Institut für Plasmaphysik, D-17491 Greifswald, Germany
S. R. Hudson
Affiliation:
Princeton Plasma Physics Laboratory, PO Box 451, Princeton, NJ 08543, USA
C. Nührenberg
Affiliation:
Max-Planck-Institut für Plasmaphysik, D-17491 Greifswald, Germany
J. Geiger
Affiliation:
Max-Planck-Institut für Plasmaphysik, D-17491 Greifswald, Germany
P. Helander
Affiliation:
Max-Planck-Institut für Plasmaphysik, D-17491 Greifswald, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

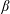A numerical investigation is carried out to understand the equilibrium $\unicode[STIX]{x1D6FD}$-limit in a classical stellarator. The stepped-pressure equilibrium code (Hudson et al., Phys. Plasmas, vol. 19 (11), 2012) is used in order to assess whether or not magnetic islands and stochastic field-lines can emerge at high 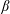$\unicode[STIX]{x1D6FD}$. Two modes of operation are considered: a zero-net-current stellarator and a fixed-iota stellarator. Despite the fact that relaxation is allowed (Taylor, Rev. Mod. Phys., vol. 58 (3), 1986, pp. 741–763), the former is shown to maintain good flux surfaces up to the equilibrium 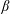$\unicode[STIX]{x1D6FD}$-limit predicted by ideal-magnetohydrodynamics (MHD), above which a separatrix forms. The latter, which has no ideal equilibrium 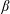$\unicode[STIX]{x1D6FD}$-limit, is shown to develop regions of magnetic islands and chaos at sufficiently high 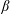$\unicode[STIX]{x1D6FD}$, thereby providing a ‘non-ideal $\unicode[STIX]{x1D6FD}$-limit’. Perhaps surprisingly, however, the value of 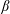$\unicode[STIX]{x1D6FD}$ at which the Shafranov shift of the axis reaches a fraction of the minor radius follows in all cases the scaling laws predicted by ideal-MHD. We compare our results to the High-Beta-Stellarator theory of Freidberg (Ideal MHD, 2014, Cambridge University Press) and derive a new prediction for the non-ideal equilibrium 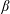$\unicode[STIX]{x1D6FD}$-limit above which chaos emerges.

Keywords

fusion plasmaplasma confinement
Type
Research Article
Information
Journal of Plasma Physics , Volume 83 , Issue 6 , December 2017 , 715830601
Copyright
© EUROfusion Consortium Research Institutions 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bhattacharjee, A., Hayashi, T., Hegna, C. C., Nakajima, N. & Sato, T. 1995 Theory of pressure-induced islands and self-healing in three-dimensional toroidal magnetohydrodynamic equilibria. Phys. Plasmas 2 (3), 883.Google Scholar
Boozer, A. H. 2004 Physics of magnetically confined plasmas. Rev. Mod. Phys. 76 (4), 1071–1141.Google Scholar
Cary, J. R. & Hanson, J. D. 1986 Stochasticity reduction. Phys. Fluids 29, 2464.CrossRefGoogle Scholar
Chirikov, B. V. 1979 A universal instability of many-dimensional oscillator systems. Phys. Rep. 52 (5), 263–379.CrossRefGoogle Scholar
Dennis, G. R., Hudson, S. R., Dewar, R. L. & Hole, M. J. 2013a The infinite interface limit of multiple-region relaxed magnetohydrodynamics. Phys. Plasmas 20 (3), 032509.CrossRefGoogle Scholar
Dennis, G. R., Hudson, S. R., Terranova, D., Franz, P., Dewar, R. L. & Hole, M. J. 2013b Minimally constrained model of self-organized helical states in reversed-field pinches. Phys. Rev. Lett. 111 (5), 1–5.Google Scholar
Drevlak, M., Monticello, D. & Reiman, A. 2005 PIES free boundary stellarator equilibria with improved initial boundary conditions. Nucl. Fusion 45, 731–740.Google Scholar
Freidberg, J. P. 2014 Ideal MHD. Cambridge University Press.Google Scholar
Greene, J. M. & Johnson, J. L. 1961 Determination of hydromagnetic equilibria. Phys. Fluids 4, 875.CrossRefGoogle Scholar
Hanson, J. D. & Cary, J. R. 1984 Elimination of stochasticity in stellarators. Phys. Fluids 27, 767.CrossRefGoogle Scholar
Hegna, C. C. 2012 Plasma flow healing of magnetic islands in stellarators. Phys. Plasmas 19 (5), 056101.CrossRefGoogle Scholar
Helander, P. 2014 Theory of plasma confinement in non-axisymmetric magnetic fields. Rep. Prog. Phys. 77 (8), 087001.CrossRefGoogle ScholarPubMed
Helander, P., Beidler, C. D., Bird, T. M., Drevlak, M., Feng, Y., Hatzky, R., Jenko, F., Kleiber, R., Proll, J. H. E., Turkin, Y. et al. 2012 Stellarator and tokamak plasmas: a comparison. Plasma Phys. Control. Fusion 54 (12), 124009.Google Scholar
Hirshman, S. P., Sanchez, R. & Cook, C. R. 2011 SIESTA: a scalable iterative equilibrium solver for toroidal applications. Phys. Plasmas 18 (6), 062504.CrossRefGoogle Scholar
Hirshman, S. P. & Whitson, J. C. 1983 Steepest-descent moment method for three-dimensional magnetohydrodynamic equilibria. Phys. Fluids 26 (1), 3553–3568.Google Scholar
Hole, M. J., Hudson, S. R. & Dewar, R. L. 2007 Equilibria and stability in partially relaxed plasma–vacuum systems. Nucl. Fusion 47 (8), 746–753.CrossRefGoogle Scholar
Hudson, S. R. & Dewar, R. L. 1997 Manipulation of islands in a heliac vacuum field. Phys. Lett. A 226, 85–92.CrossRefGoogle Scholar
Hudson, S. R., Dewar, R. L., Dennis, G., Hole, M. J., McGann, M., Von Nessi, G. & Lazerson, S. 2012 Computation of multi-region relaxed magnetohydrodynamic equilibria. Phys. Plasmas 19 (11), 112502.Google Scholar
Hudson, S. R., Hole, M. J. & Dewar, R. L. 2007 Eigenvalue problems for Beltrami fields arising in a three-dimensional toroidal magnetohydrodynamic equilibrium problem. Phys. Plasmas 14 (5), 052505.Google Scholar
Kruskal, M. D. & Kulsrud, R. M. 1958 Equilibrium of a magnetically confined plasma in a toroid. Phys. Fluids 1 (4), 265.Google Scholar
Loizu, J., Hudson, S., Bhattacharjee, A. & Helander, P. 2015a Magnetic islands and singular currents at rational surfaces in three-dimensional magnetohydrodynamic equilibria. Phys. Plasmas 22 (2), 022501.CrossRefGoogle Scholar
Loizu, J., Hudson, S. R., Bhattacharjee, A., Lazerson, S. & Helander, P. 2015b Existence of three-dimensional ideal-magnetohydrodynamic equilibria with current sheets. Phys. Plasmas 22 (9), 090704.CrossRefGoogle Scholar
Loizu, J., Hudson, S. R., Helander, P., Lazerson, S. A. & Bhattacharjee, A. 2016a Pressure-driven amplification and penetration of resonant magnetic perturbations. Phys. Plasmas 23 (5), 055703.Google Scholar
Loizu, J., Hudson, S. R. & Nührenberg, C. 2016b Verification of the SPEC code in stellarator geometries. Phys. Plasmas 23 (11), 112505.CrossRefGoogle Scholar
Meiss, J. D. 1992 Symplectic maps, variational principles and transport. Rev. Mod. Phys. 64 (3), 795–848.Google Scholar
Miyamoto, K. 2005 Plasma Physics and Controlled Nuclear Fusion. Springer.Google Scholar
Narushima, Y., Watanabe, K. Y., Sakakibara, S., Narihara, K., Yamada, I., Suzuki, Y., Ohdachi, S., Ohyabu, N., Yamada, H. & Nakamura, Y. 2008 Dependence of spontaneous growth and suppression of the magnetic island on beta and collisionality in the LHD. Nucl. Fusion 48 (7), 075010.Google Scholar
Pedersen, T. S., Otte, M., Lazerson, S., Helander, P., Bozhenkov, S., Biedermann, C., Klinger, T., Wolf, R. C., Bosch, H. S.& The Wendelstein 7-X Team 2016 Confirmation of the topology of the Wendelstein 7-X magnetic field to better than 1:100 000. Nat. Commun. 7, 13493.Google Scholar
Suzuki, Y., Nakajima, N., Watanabe, K., Nakamura, Y. & Hayashi, T. 2006 Development and application of HINT2 to helical system plasmas. Nucl. Fusion 46 (11), L19–L24.CrossRefGoogle Scholar
Suzuki, Y., Watanabe, K. Y., Sakakibara, S., Nakajima, N. & Ohyabu, N. 2008 Theoretical studies of equilibrium beta limit in heliotron plasmas. In Proceedings of the 22nd IAEA Fusion Energy Conference, Geneva, pp. 9–19. Nuclear Fusion.Google Scholar
Taylor, J. B. 1974 Relaxation of toroidal plasma and generation of reverse magnetic fields. Phys. Rev. Lett. 33 (19), 1139–1141.CrossRefGoogle Scholar
Taylor, J. B. 1986 Relaxation and magnetic reconnection in plasmas. Rev. Mod. Phys. 58 (3), 741–763.CrossRefGoogle Scholar
Waelbroeck, F. L. 1989 Current sheets and nonlinear growth of the $m=1$ kink-tearing mode. Phys. Fluids B 1 (12), 2372–2380.Google Scholar
Zakharov, L., Rogers, B. & Migliuolo, S. 1993 The theory of the early nonlinear stage of $m=1$ reconnection in tokamaks. Phys. Fluids B 5 (7), 2498–2505.Google Scholar