Article contents
Electron phase-space hole transverse instability at high magnetic field
Published online by Cambridge University Press: 04 September 2019
Abstract
Analytic treatment is presented of the electrostatic instability of an initially planar electron hole in a plasma of effectively infinite particle magnetization. It is shown that there is an unstable mode consisting of a rigid shift of the hole in the trapping direction. Its low frequency is determined by the real part of the force balance between the Maxwell stress arising from the transverse wavenumber 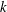 $k$ and the kinematic jetting from the hole’s acceleration. The very low growth rate arises from a delicate balance in the imaginary part of the force between the passing-particle jetting, which is destabilizing, and the resonant response of the trapped particles, which is stabilizing. Nearly universal scalings of the complex frequency and
$k$ and the kinematic jetting from the hole’s acceleration. The very low growth rate arises from a delicate balance in the imaginary part of the force between the passing-particle jetting, which is destabilizing, and the resonant response of the trapped particles, which is stabilizing. Nearly universal scalings of the complex frequency and 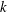 $k$ with hole depth are derived. Particle in cell simulations show that the slow-growing instabilities previously investigated as coupled hole–wave phenomena occur at the predicted frequency, but with growth rates 2 to 4 times greater than the analytic prediction. This higher rate may be caused by a reduced resonant stabilization because of numerical phase-space diffusion in the simulations.
$k$ with hole depth are derived. Particle in cell simulations show that the slow-growing instabilities previously investigated as coupled hole–wave phenomena occur at the predicted frequency, but with growth rates 2 to 4 times greater than the analytic prediction. This higher rate may be caused by a reduced resonant stabilization because of numerical phase-space diffusion in the simulations.
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press 2019
References
- 6
- Cited by


