Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sorriso-Valvo, Luca
Catapano, Filomena
Retinò, Alessandro
Le Contel, Olivier
Perrone, Denise
Roberts, Owen W.
Coburn, Jesse T.
Panebianco, Vincenzo
Valentini, Francesco
Perri, Silvia
Greco, Antonella
Malara, Francesco
Carbone, Vincenzo
Veltri, Pierluigi
Pezzi, Oreste
Fraternale, Federico
Di Mare, Francesca
Marino, Raffaele
Giles, Barbara
Moore, Thomas E.
Russell, Christopher T.
Torbert, Roy B.
Burch, Jim L.
and
Khotyaintsev, Yuri V.
2019.
Turbulence-Driven Ion Beams in the Magnetospheric Kelvin-Helmholtz Instability.
Physical Review Letters,
Vol. 122,
Issue. 3,
Andrés, N.
Sahraoui, F.
Galtier, S.
Hadid, L. Z.
Ferrand, R.
and
Huang, S. Y.
2019.
Energy Cascade Rate Measured in a Collisionless Space Plasma with MMS Data and Compressible Hall Magnetohydrodynamic Turbulence Theory.
Physical Review Letters,
Vol. 123,
Issue. 24,
Ferrand, R.
Galtier, S.
Sahraoui, F.
Meyrand, R.
Andrés, N.
and
Banerjee, S.
2019.
On Exact Laws in Incompressible Hall Magnetohydrodynamic Turbulence.
The Astrophysical Journal,
Vol. 881,
Issue. 1,
p.
50.
Andrés, Nahuel
and
Banerjee, Supratik
2019.
Statistics of incompressible hydrodynamic turbulence: An alternative approach.
Physical Review Fluids,
Vol. 4,
Issue. 2,
Girichidis, Philipp
Offner, Stella S. R.
Kritsuk, Alexei G.
Klessen, Ralf S.
Hennebelle, Patrick
Kruijssen, J. M. Diederik
Krause, Martin G. H.
Glover, Simon C. O.
and
Padovani, Marco
2020.
Physical Processes in Star Formation.
Space Science Reviews,
Vol. 216,
Issue. 4,
Matthaeus, William H.
Yang, Yan
Wan, Minping
Parashar, Tulasi N.
Bandyopadhyay, Riddhi
Chasapis, Alexandros
Pezzi, Oreste
and
Valentini, Francesco
2020.
Pathways to Dissipation in Weakly Collisional Plasmas.
The Astrophysical Journal,
Vol. 891,
Issue. 1,
p.
101.
Banerjee, Supratik
and
Andrés, Nahuel
2020.
Scale-to-scale energy transfer rate in compressible two-fluid plasma turbulence.
Physical Review E,
Vol. 101,
Issue. 4,
Grete, Philipp
O’Shea, Brian W.
and
Beckwith, Kris
2020.
As a Matter of State: The Role of Thermodynamics in Magnetohydrodynamic Turbulence.
The Astrophysical Journal,
Vol. 889,
Issue. 1,
p.
19.
Ferrand, R.
Galtier, S.
Sahraoui, F.
and
Federrath, C.
2020.
Compressible Turbulence in the Interstellar Medium: New Insights from a High-resolution Supersonic Turbulence Simulation.
The Astrophysical Journal,
Vol. 904,
Issue. 2,
p.
160.
Sahraoui, Fouad
Hadid, Lina
and
Huang, Shiyong
2020.
Magnetohydrodynamic and kinetic scale turbulence in the near-Earth space plasmas: a (short) biased review.
Reviews of Modern Plasma Physics,
Vol. 4,
Issue. 1,
Andrés, Nahuel
Romanelli, Norberto
Hadid, Lina Z.
Sahraoui, Fouad
DiBraccio, Gina
and
Halekas, Jasper
2020.
Solar Wind Turbulence Around Mars: Relation between the Energy Cascade Rate and the Proton Cyclotron Waves Activity.
The Astrophysical Journal,
Vol. 902,
Issue. 2,
p.
134.
Rakhmanova, Liudmila
Riazantseva, Maria
and
Zastenker, Georgy
2021.
Plasma and Magnetic Field Turbulence in the Earth’s Magnetosheath at Ion Scales.
Frontiers in Astronomy and Space Sciences,
Vol. 7,
Issue. ,
Magyar, N.
and
Nakariakov, V. M.
2021.
Three-dimensional Simulations of the Inhomogeneous Low Solar Wind.
The Astrophysical Journal,
Vol. 907,
Issue. 1,
p.
55.
Andrés, N.
Sahraoui, F.
Hadid, L. Z.
Huang, S. Y.
Romanelli, N.
Galtier, S.
DiBraccio, G.
and
Halekas, J.
2021.
The Evolution of Compressible Solar Wind Turbulence in the Inner Heliosphere: PSP, THEMIS, and MAVEN Observations.
The Astrophysical Journal,
Vol. 919,
Issue. 1,
p.
19.
Vásconez, Christian L.
Perrone, D.
Marino, R.
Laveder, D.
Valentini, F.
Servidio, S.
Mininni, P.
and
Sorriso-Valvo, L.
2021.
Local and global properties of energy transfer in models of plasma turbulence.
Journal of Plasma Physics,
Vol. 87,
Issue. 1,
Yang, Yan
Wan, Minping
Matthaeus, William H.
and
Chen, Shiyi
2021.
Energy budget in decaying compressible MHD turbulence.
Journal of Fluid Mechanics,
Vol. 916,
Issue. ,
Ferrand, Renaud
Galtier, Sébastien
and
Sahraoui, Fouad
2021.
A compact exact law for compressible isothermal Hall magnetohydrodynamic turbulence.
Journal of Plasma Physics,
Vol. 87,
Issue. 2,
Simon, P.
and
Sahraoui, F.
2021.
General Exact Law of Compressible Isentropic Magnetohydrodynamic Flows: Theory and Spacecraft Observations in the Solar Wind.
The Astrophysical Journal,
Vol. 916,
Issue. 1,
p.
49.
Matthaeus, W. H.
2021.
Turbulence in space plasmas: Who needs it?.
Physics of Plasmas,
Vol. 28,
Issue. 3,
p.
032306.
Quijia, Paulina
Fraternale, Federico
Stawarz, Julia E
Vásconez, Christian L
Perri, Silvia
Marino, Raffaele
Yordanova, Emiliya
and
Sorriso-Valvo, Luca
2021.
Comparing turbulence in a Kelvin–Helmholtz instability region across the terrestrial magnetopause.
Monthly Notices of the Royal Astronomical Society,
Vol. 503,
Issue. 4,
p.
4815.
 $M_{S}$ and different magnetic guide fields
$M_{S}$ and different magnetic guide fields 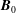 $\boldsymbol{B}_{0}$ . The dominant contribution to the energy cascade rate comes from the compressible flux, which depends weakly on the magnetic guide field
$\boldsymbol{B}_{0}$ . The dominant contribution to the energy cascade rate comes from the compressible flux, which depends weakly on the magnetic guide field 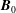 $\boldsymbol{B}_{0}$ , unlike the other terms whose moduli increase significantly with
$\boldsymbol{B}_{0}$ , unlike the other terms whose moduli increase significantly with 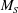 $M_{S}$ and
$M_{S}$ and 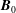 $\boldsymbol{B}_{0}$ . In particular, for strong
$\boldsymbol{B}_{0}$ . In particular, for strong 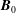 $\boldsymbol{B}_{0}$ the source and hybrid terms are dominant at small scales with almost the same amplitude but with a different sign. A statistical analysis undertaken with an isotropic decomposition based on the SO(3) rotation group is shown to generate spurious results in the presence of
$\boldsymbol{B}_{0}$ the source and hybrid terms are dominant at small scales with almost the same amplitude but with a different sign. A statistical analysis undertaken with an isotropic decomposition based on the SO(3) rotation group is shown to generate spurious results in the presence of 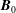 $\boldsymbol{B}_{0}$ , when compared with an axisymmetric decomposition better suited to the geometry of the problem. Our numerical results are compared with previous analyses made with in situ measurements in the solar wind and the terrestrial magnetosheath.
$\boldsymbol{B}_{0}$ , when compared with an axisymmetric decomposition better suited to the geometry of the problem. Our numerical results are compared with previous analyses made with in situ measurements in the solar wind and the terrestrial magnetosheath.