Published online by Cambridge University Press: 14 June 2018
So far, only one distribution function giving rise to a collisionless nonlinear force-free current sheet equilibrium allowing for a plasma beta less than one is known (Allanson et al., Phys. Plasmas, vol. 22 (10), 2015, 102116; Allanson et al., J. Plasma Phys., vol. 82 (3), 2016a, 905820306). This distribution function can only be expressed as an infinite series of Hermite functions with very slow convergence and this makes its practical use cumbersome. It is the purpose of this paper to present a general method that allows us to find distribution functions consisting of a finite number of terms (therefore easier to use in practice), but which still allow for current sheet equilibria that can, in principle, have an arbitrarily low plasma beta. The method involves using known solutions and transforming them into new solutions using transformations based on taking integer powers ( $N$) of one component of the pressure tensor. The plasma beta of the current sheet corresponding to the transformed distribution functions can then, in principle, have values as low as
$N$) of one component of the pressure tensor. The plasma beta of the current sheet corresponding to the transformed distribution functions can then, in principle, have values as low as 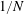 $1/N$. We present the general form of the distribution functions for arbitrary
$1/N$. We present the general form of the distribution functions for arbitrary  $N$ and then, as a specific example, discuss the case for
$N$ and then, as a specific example, discuss the case for  $N=2$ in detail.
$N=2$ in detail.