Article contents
Deformation Kinetics of Steady Creep in Sn/Pb Solder Alloys With Applications
Published online by Cambridge University Press: 05 May 2011
Abstract
In this paper, a constitutive equation of steady creep rates:
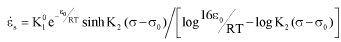
is derived based on a liaison of the theory of irreversible thermodynamics of continuous media with internal state variables (ISV); and the deformation kinetics. In steady creep, only one ISV is used, whose evolution equation is derived by the concepts of deformation kinetics, in which micromotions are generated by a group of atoms climbing over a tilted potential barrier of the highest height ε0.
Applications of the constitutive equation in the cases of some Sn/Pb solder alloys-63Sn/37Pb, 60Sn/40Pb and 97.5Pb/2.5Sn under shear creep tests; and 98Pb/2Sn under tensile creep tests, show that the theoretical results can describe the experimental data quite well. These results pave the way for future research in the comparisons of creep resistance among solders with various Sn/Pb compositions and in the generalization of three-dimensional constitutive equation.
Keywords
- Type
- Articles
- Information
- Copyright
- Copyright © The Society of Theoretical and Applied Mechanics, R.O.C. 2004
References
- 1
- Cited by


