Article contents
A van der Waals contact-bond model for low-dimensional nanoscale carbon materials based on the quasi-continuum method
Published online by Cambridge University Press: 09 December 2019
Abstract
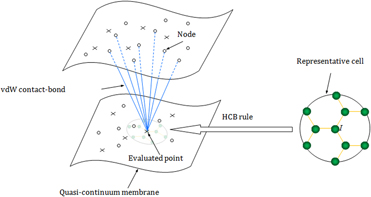
In order to develop an efficient and accurate quasi-continuum approach for contact problems of low-dimensional nanoscale carbon materials, a van der Waals contact-bond model is proposed in this study. This method can involve the important information of nano- and micro-structures, such as the bonded carbon atom interactions and the long-range van der Waals effect, which is usually homogenized and neglected in continuum methods. The degree of freedom of the atomic systems can be reduced dramatically; therefore, the model is beneficial for rapid simulations and large-scale computations of carbon nano-components. The so-called higher-order Cauchy–Born rule is used to establish the geometrically consistent constitutive model, and a meshless local Petrov–Galerkin-based computational framework is constructed for the mechanical responses of carbon nanoscale systems. The stiffness matrix is derived analytically, and the incremental governing equation is solved with the Newton–Raphson iteration method. Consequently, this method is much faster than order-N2 approaches such as molecular dynamic simulation.
Keywords
- Type
- Article
- Information
- Copyright
- Copyright © Materials Research Society 2019
References
- 7
- Cited by


