Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-16T13:24:16.579Z Has data issue: false hasContentIssue false
  • English
  • Français

Residual stress–driven test technique for freestanding ultrathin films: Elastic behavior and residual strain

Published online by Cambridge University Press:  14 October 2019

Gayatri K. Cuddalorepatta Open the ORCID record for Gayatri K. Cuddalorepatta [Opens in a new window] ,
Gi-Dong Sim ,
Han Li ,
Daniel Pantuso  and
Joost J. Vlassak
Gayatri K. Cuddalorepatta*
Affiliation:
School of Engineering and Applied Science, Harvard University, Cambridge, Massachusetts 02138, USA
Gi-Dong Sim
Affiliation:
School of Engineering and Applied Science, Harvard University, Cambridge, Massachusetts 02138, USA
Han Li
Affiliation:
Technology Manufacturing Group, Intel Corporation, Hillsboro, Oregon 97124, USA
Daniel Pantuso
Affiliation:
Technology Manufacturing Group, Intel Corporation, Hillsboro, Oregon 97124, USA
Joost J. Vlassak
Affiliation:
School of Engineering and Applied Science, Harvard University, Cambridge, Massachusetts 02138, USA
*
a)Address all correspondence to this author. [email protected]
Get access

Abstract

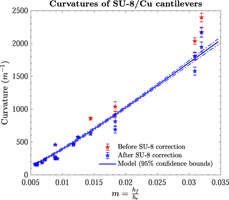
Elastic modulus and residual stress in freestanding ultrathin films (<100 nm) are characterized using bilayer cantilevers. The cantilevers comprise a test film and a well-characterized reference material (SU-8). When released from the substrate, residual stresses in the bilayer cantilever cause it to deflect with measurable curvatures, allowing the determination of both stiffness and residual stress of the test film. The technique does not require sophisticated mechanical test equipment and serves as a useful metrology tool for characterizing coatings immediately after fabrication in a clean room assembly line. The measured biaxial modulus and residual strain of 75 nm copper films are 211 ± 19 GPa and (7.05 ± 0.22) × 10−3, respectively. Additional experiments on the freestanding structures yield a mean Young’s modulus of 115 GPa. These properties are in close agreement with those measured from additional residual stress–driven structures developed on the same coatings by the authors.

Keywords

ultrathin filmbiaxial moduluselastic behaviorresidual strainresidual stresscurvaturefreestandingXeF2 etchcopperforce deflectionSU-8
Type
Article
Information
Journal of Materials Research , Volume 34 , Issue 20 , 28 October 2019 , pp. 3474 - 3482
Copyright
Copyright © Materials Research Society 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

b)

Present Address: KAIST, Daejeon, South Korea.

References

Arzt, E.: Size effects in materials due to microstructural and dimensional constraints: A comparative review. Acta Mater. 46, 5611–5626 (1998).CrossRefGoogle Scholar
Hahn, E.N. and Meyers, M.A.: Grain-size dependent mechanical behavior of nanocrystalline metals. Mater. Sci. Eng., A 646, 101–134 (2015).CrossRefGoogle Scholar
Chokshi, A.H., Rosen, A., Karch, J., and Gleiter, H.: On the validity of the Hall–Petch relationship in nanocrystalline materials. Scr. Metall. 23, 1679–1683 (1989).CrossRefGoogle Scholar
Haque, M.A. and Saif, M.T.A.: Mechanical behavior of 30–50 mn thick aluminum films under uniaxial tension. Scr. Mater. 47, 863–867 (2002).CrossRefGoogle Scholar
Budiansky, B. and O’Connell, R.J.: Elastic-moduli of a cracked solid. Int. J. Solids Struct. 12, 81–97 (1976).CrossRefGoogle Scholar
Huang, H.B.: Mechanical properties of freestanding polycrystalline metallic thin films and multilayers. Ph.D. thesis, Harvard University, Cambridge, Massachusetts, 1998.Google Scholar
Nan, C.W., Li, X.P., Cai, K.F., and Tong, J.Z.: Grain size-dependent elastic moduli of nanocrystals. J. Mater. Sci. Lett. 17, 1917–1919 (1998).CrossRefGoogle Scholar
Phillpot, S.R., Wolf, D., and Gleiter, H.: Molecular-dynamics study of the synthesis and characterization of a fully dense, 3-dimensional nanocrystalline material. J. Appl. Phys. 78, 847–861 (1995).CrossRefGoogle Scholar
Zhou, K.: Effects of grain size and shape on mechanical properties of nanocrystalline copper investigated by molecular dynamics. Mater. Sci. Eng., A 615, 92–97 (2014).CrossRefGoogle Scholar
Okolo, B.C.: Stress and microstructure of sputter deposited thin copper and niobium films. Ph.D. thesis, Universitätsbibliothek der Universität Stuttgart, Stuttgart, 2003.Google Scholar
Li, X.X.: Ultrathin single-crystalline-silicon cantilever resonators: Fabrication technology and significant specimen size effect on Young’s modulus. Appl. Phys. Lett. 83, 3081–3308 (2003).CrossRefGoogle Scholar
Suresh, S., Nieh, T.G., and Choi, B.W.: Nano-indentation of copper thin films on silicon substrates. Scr. Mater. 41, 951–957 (1999).CrossRefGoogle Scholar
Vlassak, J.J. and Nix, W.D.: A new bulge test technique for the determination of Young modulus and Poisson’s ratio of thin-films. J. Mater. Res. 7, 3242–3249 (1992).CrossRefGoogle Scholar
Sim, G.D., Park, J.H., Uchic, M.D., Shade, P.A., Lee, S.B., and Vlassak, J.J.: An apparatus for performing microtensile tests at elevated temperatures inside a scanning electron microscope. Acta Mater. 61, 7500–7510 (2013).CrossRefGoogle Scholar
Chang, J.Y., Yu, G.P., and Huang, J.H.: Determination of Young’s modulus and Poisson’s ratio of thin films by combining sin2ψ X-ray diffraction and laser curvature methods. Thin Solid Films 517, 6759–6766 (2009).CrossRefGoogle Scholar
Coulombier, M., Guisbiers, G., Colla, M.S., Vayrette, R., Raskin, J.P., and Pardoen, T.: On-chip stress relaxation testing method for freestanding thin film materials. Rev. Sci. Instrum. 83, 9 (2012).CrossRefGoogle ScholarPubMed
Favache, A.: A generic “micro-stoney” method for the measurement of internal stress and elastic modulus of ultrathin films. Rev. Sci. Instrum. 87, 9 (2016).CrossRefGoogle ScholarPubMed
Weihs, T.P., Hong, S., Bravman, J.C., and Nix, W.D.: Mechanical deflection of cantilever microbeams—A new technique for testing the mechanical-properties of thin-films. J. Mater. Res. 3, 931–942 (1988).CrossRefGoogle Scholar
Cuddalorepatta, G.K., Li, H., Pantuso, D., and Vlassak, J.J.: Stress–strain behavior of freestanding ultra thin films (2019). Manuscript in preparation.CrossRefGoogle Scholar
Cuddalorepatta, G.K., van Rees, W.M., Li, H., Pantuso, D., Mahadevan, L.N., and Vlassak, J.J.: Poisson’s ratio and residual strain of freestanding ultra thin films. J. Mech. Phys. Solids (2019). Manuscript in preparation.Google Scholar
Lorenz, H., Despont, M., Fahrni, N., LaBianca, N., Renaud, P., and Vettiger, P.: SU-8: A low-cost negative resist for MEMS. J. Micromech. Microeng. 7, 121–124 (1997).CrossRefGoogle Scholar
Hopcroft, M., Kramer, T., Kim, G., Takashima, K., Higo, Y., Moore, D., and Brugger, J.: Micromechanical testing of SU-8 cantilevers. Fatigue Fract. Eng. Mater. Struct. 28, 735–742 (2005).CrossRefGoogle Scholar
Landolt, H. and Börnstein, R.: Single Crystal Elastic Constants and Calculated Aggregate Properties: A Handbook (Springer-Verlag, Berlin, 1979).Google Scholar
Schiotz, J., Di Tolla, F.D., and Jacobsen, K.W.: Softening of nanocrystalline metals at very small grain sizes. Nature 391, 561–563 (1998).CrossRefGoogle Scholar
Prokoshkina, D. and Esin, V.A.: Grain boundary width, energy and self-diffusion in nickel: Effect of material purity. Acta Mater. 61, 5188 (2013).CrossRefGoogle Scholar
Sim, G.D., Choi, Y.S., Lee, D., Oh, K.H., and Vlassak, J.J.: High tensile strength of sputter-deposited ZrB2 ceramic thin films measured up to 1016 K. Acta Mater. 113, 32–40 (2016).CrossRefGoogle Scholar
Eshelby, J.D.: The determination of the elastic field of an ellipsoidal inclusion and related problems. Proc. R. Soc. London, Ser. A 241, 376–396 (1957).Google Scholar
Huang, H.B. and Spaepen, F.: Tensile testing of free-standing cu, ag and al thin films and Ag/Cu multilayers. Acta Mater. 48, 3261–3269 (2000).CrossRefGoogle Scholar
Ledbetter, H.M. and Naimon, E.R.: Elastic properties of metals and alloys. II. Copper. J. Phys. Chem. Ref. Data 3, 897 (1974).CrossRefGoogle Scholar
Keller, S.S., Blagoi, G., Lillemose, M., Haefliger, D., and Boisen, A.: Processing of thin SU-8 films. J. Micromech. Microeng. 18, 10 (2008).CrossRefGoogle Scholar
Nordstrom, M., Keller, S., and Lillemose, M.: SU-8 cantilevers for bio/chemical sensing; fabrication, characterization and development of novel read-out methods. Sensors 8, 1595–1612 (2008).CrossRefGoogle Scholar
Lee, S.J., Shi, W., Maciel, P., and Cha, S.W.: Top-edge profile control for SU-8 structural photoresist. In Proceedings of the 15th Biennial University/Government/Industry Microelectronics Symposium (Cat. No. 03CH37488) (IEEE, Boise, Idaho, 2003); pp. 389–390.CrossRefGoogle Scholar
Thompson, C.V.: Structure evolution during processing of polycrystalline films. Annu. Rev. Mater. Sci. 30, 159–190 (2000).CrossRefGoogle Scholar
Freund, L.B., Floro, J.A., and Chason, E.: Extensions of the stoney formula for substrate curvature to configurations with thin substrates or large deformations. Appl. Phys. Lett. 74, 1987 (1999).CrossRefGoogle Scholar
Schafer, R.W.: What is a Savitzky–Golay filter? [lecture notes]. IEEE Signal Process. Mag. 28, 111–117 (2011).CrossRefGoogle Scholar
Supplementary material: File

Cuddalorepatta et al. supplementary material

Cuddalorepatta et al. supplementary material 1

Download Cuddalorepatta et al. supplementary material(File)
File 2.3 MB
Supplementary material: Image

Cuddalorepatta et al. supplementary material

Cuddalorepatta et al. supplementary material 2

Download Cuddalorepatta et al. supplementary material(Image)
Image 406.5 KB