Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-24T06:38:55.706Z Has data issue: false hasContentIssue false
  • English
  • Français

Many-electron effects on optical absorption spectra of strained graphene

Published online by Cambridge University Press:  20 December 2011

Yufeng Liang ,
Shouting Huang  and
Li Yang
Yufeng Liang
Affiliation:
Department of Physics, Washington University, St. Louis, Missouri 63130
Shouting Huang
Affiliation:
Department of Physics, Washington University, St. Louis, Missouri 63130
Li Yang*
Affiliation:
Department of Physics, Washington University, St. Louis, Missouri 63130
*
a)Address all correspondence to this author. e-mail: [email protected]
Get access

Abstract

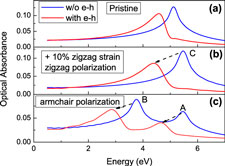
We use the first-principles GW + Bethe–Salpeter equation approach to study the electronic structure and optical absorption spectra of uniaxial strained graphene. Applied strain induces an anisotropic Fermi velocity and tilts the axis of the Dirac cone. As a result, the optical response of strained graphene is dramatically changed; the optical absorption is anisotropic; the characteristic single optical absorption peak of pristine graphene is split into two peaks with enhanced excitonic effects. Within the infrared regime, the optical absorbance of uniaxial strained graphene is no longer a constant because of the broken symmetry and anisotropic excitonic effects. Within the visible-light regime, we observe a prominent optical absorption peak due to an enhanced red shift by electron–hole interactions, enabling us to change the visible color and transparency of stretched graphene. Finally, we also reveal enhanced excitonic effects within the ultraviolet regime, where a few nearly bound excitons are identified.

Keywords

Optical propertiesCElectronic structure
Type
Invited Feature Paper
Information
Journal of Materials Research , Volume 27 , Issue 2 , 28 January 2012 , pp. 403 - 409
Copyright
Copyright © Materials Research Society 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

1.Novoselov, K.S., Geim, A.K., Morozov, S.V., Jiang, D., Zhang, Y., Dubonos, S.V., Grigorieva, I.V., and Firsov, A.A.: Electric field effect in atomically thin carbon films. Science 306, 666 (2004).CrossRefGoogle ScholarPubMed
2.Novoselov, K.S., Geim, A.K., Morozov, S.V., Jiang, D., Katsnelson, M.I., Grigorieva, I.V., Dubonos, S.V., and Firsov, A.A.: Two-dimensional gas of massless Dirac fermions in graphene. Nature 438, 197 (2005).CrossRefGoogle ScholarPubMed
3.Zhang, Y., Tan, Y-W., Stormer, H.L., and Kim, P.: Experimental observation of the quantum Hall effect and Berry’s phase in graphene. Nature 438, 201 (2005).CrossRefGoogle ScholarPubMed
4.Berger, C., Song, Z., Li, T., Li, X., Ogbazghi, A.Y., Feng, R., Dai, Z., Marchenkov, A.N., Conrad, E.H., First, P.N., and de Heer, W.A.: Ultrathin epitaxial graphite: 2D electron gas properties and a route toward graphene-based nanoelectronics. J. Phys. Chem. B 108, 19912 (2004).CrossRefGoogle Scholar
5.Geim, A.K. and Novoselov, K.S.: The rise of graphene. Nat. Mater. 6, 183 (2007).CrossRefGoogle ScholarPubMed
6.Castro Neto, A.H., Guinea, F., Peres, N.M.R., Novoselov, K.S., and Geim, A.K.: The electronic properties of graphene. Rev. Mod. Phys. 81, 109 (2009).CrossRefGoogle Scholar
7.Gusynin, V.P. and Sharapov, S.G.: Transport of Dirac quasiparticles in graphene: Hall and optical conductivities. Phys. Rev. B 73, 245411 (2006).CrossRefGoogle Scholar
8.Nair, R.R., Blake, P., Grigorenko, A.N., Novoselov, K.S., Booth, T.J., Stauber, T., Peres, N.M.R., and Geim, A.K.: Fine structure constant defines visual transparency of graphene. Science 320, 1308 (2008).CrossRefGoogle ScholarPubMed
9.Kravets, V.G., Grigorenko, A.N., Nair, R.R., Blake, P., Anissimova, S., Novoselov, K.S., and Geim, A.K.: Spectroscopic ellipsometry of graphene and an exciton-shifted van Hove peak in absorption. Phys. Rev. B 81, 155413 (2010).CrossRefGoogle Scholar
10.Mak, K.F., Sfeir, M.Y., Wu, Y., Lui, H., Misewich, J.A., and Heinz, T.F.: Measurement of the optical conductivity of graphene. Phys. Rev. Lett. 101, 196405 (2008).CrossRefGoogle ScholarPubMed
11.Wang, F., Zhang, Y., Tian, C., Girit, C., Zettl, A., Crommie, M., and Ron Shen, Y.: Gate-variable optical transitions in graphene. Science 320, 206 (2008).CrossRefGoogle ScholarPubMed
12.Kim, K.S., Zhao, Y., Jang, H., Lee, S.Y., Kim, J.M., Kim, K.S., Ahn, J.H., Kim, P., Choi, J., and Hong, B.H.: Large-scale pattern growth of graphene films for stretchable transparent electrodes. Nature 457, 706 (2009).CrossRefGoogle ScholarPubMed
13.Lee, C., Wei, X., Kysar, J.W., and Hone, J.: Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 321, 385 (2008).CrossRefGoogle ScholarPubMed
14.Pellegrino, F.M.D., Angilella, G.G.N., and Pucci, R.: Effect of uniaxial strain on the reflectivity of graphene. High Pressure Res. 29, 569 (2009).CrossRefGoogle Scholar
15.Pellegrino, F.M.D., Angilella, G.G.N., and Pucci, R.: Strain effect on the optical conductivity of graphene. Phys. Rev. B 81, 035411 (2010).CrossRefGoogle Scholar
16.Sinner, A., Sedrakyan, A., and Ziegler, K.: Optical conductivity of graphene in the presence of random lattice deformations. Phys. Rev. B 83, 155115 (2011).CrossRefGoogle Scholar
17.Pereira, V.M., Ribeiro, R.M., Peres, N.M.R., and Castro Neto, A.H.: Optical properties of strained graphene. Europhys. Lett. 92, 67001 (2011).CrossRefGoogle Scholar
18.Choi, S-M., Jhi, S-H., and Son, Y.W.: Effects of strain on electronic properties of graphene. Phys. Rev. B 81, 081407 (2010).CrossRefGoogle Scholar
19.Yang, L., Deslippe, J., Park, C-H., Cohen, M.L., and Louie, S.G.: Excitonic effects on the optical response of graphene and bilayer graphene. Phys. Rev. Lett. 103, 186802 (2009).CrossRefGoogle ScholarPubMed
20.Mak, K.F., Shan, J., and Heinz, T.F.: Seeing many-body effects in single- and few-layer graphene: Observation of two-dimensional saddle-point excitons. Phys. Rev. Lett. 106, 046401 (2011).CrossRefGoogle ScholarPubMed
21.Yang, L.: Excitonic effects on optical absorption spectra of doped graphene. Nano Lett. 11, 3844 (2011).CrossRefGoogle ScholarPubMed
22.Rohlfing, M. and Louie, S.G.: Electron-hole excitations and optical spectra from first principles. Phys. Rev. B 62, 4927 (2000).CrossRefGoogle Scholar
23.Hohenberg, P. and Kohn, W.: Inhomogeneous electron gas. Phys. Rev. 136, B864 (1964).CrossRefGoogle Scholar
24.Kohn, W. and Sham, L.J.: Self-consistent equations including exchange and correlation effects. Phys. Rev. 140, A1133 (1965).CrossRefGoogle Scholar
25.Hedin, L.: New method for calculating the one-particle Green’s function with application to the electron-gas problem. Phys. Rev. 139, A796 (1965).CrossRefGoogle Scholar
26.Hybertsen, M.S. and Louie, S.G.: Electron correlation in semiconductors and insulators: Band gaps and quasiparticle energies. Phys. Rev. B. 34, 5390 (1986).CrossRefGoogle ScholarPubMed
27.Onida, G., Reining, L., and Rubio, A.: Electronic excitations: Density-functional versus many-body Green’s-function approaches. Rev. Mod. Phys. 74, 601 (2002).CrossRefGoogle Scholar
28.Troullier, N. and Martins, J.L.: Efficient pseudopotentials for plane-wave calculations. Phys. Rev. B 43, 1993 (1991).CrossRefGoogle ScholarPubMed
29.Trevisanutto, P.E., Holzmann, M., Cote, M., and Olevano, V.: Ab initio high-energy excitonic effects in graphite and graphene. Phys. Rev. B 81, 121405 (2010).CrossRefGoogle Scholar
30.Yang, L.: Excitons in intrinsic and bilayer graphene. Phys. Rev. B 83, 085405 (2011).CrossRefGoogle Scholar
31.Fetter, A. and Walecka, J.D.: Quantum Theory of Many Particle Systems (McGraw-Hill, San Francisco, 1971), p. 538.Google Scholar