Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T09:40:25.922Z Has data issue: false hasContentIssue false
  • English
  • Français

Grain size effects on dynamic fracture instability in polycrystalline graphene under tear loading

Published online by Cambridge University Press:  13 March 2019

Yuxin Zhao ,
Yunfei Xu ,
Xiaoyi Liu Open the ORCID record for Xiaoyi Liu [Opens in a new window] ,
Jun Zhu  and
Sheng-Nian Luo
Yuxin Zhao
Affiliation:
College of Physical Science and Technology, Sichuan University, Chengdu, Sichuan 610064, People’s Republic of China; and The Peac Institute of Multiscale Sciences, Chengdu, Sichuan 610031, People’s Republic of China
Yunfei Xu
Affiliation:
The Peac Institute of Multiscale Sciences, Chengdu, Sichuan 610031, People’s Republic of China
Xiaoyi Liu
Affiliation:
The Peac Institute of Multiscale Sciences, Chengdu, Sichuan 610031, People’s Republic of China
Jun Zhu*
Affiliation:
College of Physical Science and Technology, Sichuan University, Chengdu, Sichuan 610064, People’s Republic of China
Sheng-Nian Luo*
Affiliation:
The Peac Institute of Multiscale Sciences, Chengdu, Sichuan 610031, People’s Republic of China; and Key Laboratory of Advanced Technologies of Materials, Ministry of Education, Southwest Jiaotong University, Chengdu, Sichuan 610031, People’s Republic of China
*
a)Address all correspondence to these authors. e-mail: [email protected]
b)e-mail: [email protected]
Get access

Abstract

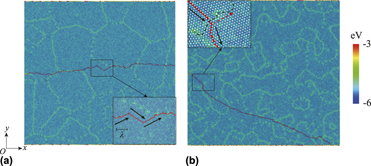
The stability of dynamic fracture is a fundamental and challenging problem in the field of materials science. The grain size effect on dynamic fracture instability in polycrystalline graphene under tear loading is explored via theoretical analysis and molecular dynamics simulations. The fracture stability phase diagram in terms of grain size and crack propagation velocity is obtained, and three regions of crack propagation are identified: stable, metastable, and unstable. For grain size above 2 nm, there exists a critical velocity beyond which fracture instability occurs, and this critical velocity depends linearly on grain size. Decreasing grain size leads to reduced characteristic time for correction of crack path deflection, which plays a dominant role in dynamic fracture instabilities. However, when grain size is below 2 nm, there does not exist a critical velocity for steady propagation of cracks due to discontinuous effects. Our results also provide a valuable insight into dynamic fracture of polycrystalline graphene as well as other 2D and quasi-2D materials.

Keywords

polycrystalfracturegrain size
Type
Invited Paper
Information
Journal of Materials Research , Volume 34 , Issue 13: Focus Issue: Intrinsic and Extrinsic Size Effects in Materials , 15 July 2019 , pp. 2209 - 2217
Copyright
Copyright © Materials Research Society 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Buehler, M.J. and Gao, H.: Dynamical fracture instabilities due to local hyperelasticity at crack tips. Nature 439, 307 (2006).CrossRefGoogle ScholarPubMed
Swadener, J., Baskes, M., and Nastasi, M.: Molecular dynamics simulation of brittle fracture in silicon. Phys. Rev. Lett. 89, 085503 (2002).CrossRefGoogle ScholarPubMed
Belytschko, T., Xiao, S., Schatz, G., and Ruoff, R.: Atomistic simulations of nanotube fracture. Phys. Rev. B 65, 235430 (2002).CrossRefGoogle Scholar
Freund, L.B.: Dynamic Fracture Mechanics (Cambridge university Press, Cambridge, U.K., 1998).Google Scholar
Broberg, K.B.: Cracks and Fracture (Elsevier, San Diego, California, 1999).Google Scholar
Yoffe, E.H.: The moving Griffith crack. Philos. Mag. 42, 739–750 (1951).CrossRefGoogle Scholar
Gao, H.: Surface roughening and branching instabilities in dynamic fracture. J. Mech. Phys. Solids 41, 457–486 (1993).CrossRefGoogle Scholar
Gao, H.: A theory of local limiting speed in dynamic fracture. J. Mech. Phys. Solids 44, 1453–1474 (1996).CrossRefGoogle Scholar
Gao, H.: Elastic waves in a hyperelastic solid near its plane-strain equibiaxial cohesive limit. Philos. Mag. Lett. 76, 307–314 (1997).CrossRefGoogle Scholar
Rezaei, S., Wulfinghoff, S., and Reese, S.: Prediction of fracture and damage in micro/nano coating systems using cohesive zone elements. Int. J. Solids Struct. 121, 62–74 (2017).CrossRefGoogle Scholar
Daniel, R., Meindlhumer, M., Baumegger, W., Zalesak, J., Sartory, B., Burghammer, M., Mitterer, C., and Keckes, J.: Grain boundary design of thin films: Using tilted brittle interfaces for multiple crack deflection toughening. Acta Mater. 122, 130–137 (2017).CrossRefGoogle Scholar
Tehranchi, A. and Curtin, W.: Atomistic study of hydrogen embrittlement of grain boundaries in nickel: I. Fracture. J. Mech. Phys. Solids 101, 150–165 (2017).CrossRefGoogle Scholar
Leitner, K., Scheiber, D., Jakob, S., Primig, S., Clemens, H., Povoden-Karadeniz, E., and Romaner, L.: How grain boundary chemistry controls the fracture mode of molybdenum. Mater. Des. 142, 36–43 (2018).CrossRefGoogle Scholar
Hull, D. and Rimmer, D.: The growth of grain-boundary voids under stress. Philos. Mag. 4, 673–687 (1959).CrossRefGoogle Scholar
Anderson, T.L.: Fracture Mechanics: Fundamentals and Applications (CRC Press, Boca Raton, 2017).CrossRefGoogle Scholar
Holland, D. and Marder, M.: Ideal brittle fracture of silicon studied with molecular dynamics. Phys. Rev. Lett. 80, 746 (1998).CrossRefGoogle Scholar
Holian, B.L. and Ravelo, R.: Fracture simulations using large-scale molecular dynamics. Phys. Rev. B 51, 11275 (1995).CrossRefGoogle ScholarPubMed
Bittencourt, T., Wawrzynek, P., Ingraffea, A., and Sousa, J.: Quasi-automatic simulation of crack propagation for 2D LEFM problems. Eng. Fract. Mech. 55, 321–334 (1996).CrossRefGoogle Scholar
Rafii-Tabar, H., Hua, L., and Cross, M.: A multi-scale atomistic-continuum modelling of crack propagation in a two-dimensional macroscopic plate. J. Phys.: Condens. Matter 10, 2375 (1998).Google Scholar
Mas-Balleste, R., Gomez-Navarro, C., Gomez-Herrero, J., and Zamora, F.: 2D materials: To graphene and beyond. Nanoscale 3, 20–30 (2011).CrossRefGoogle Scholar
Novoselov, K.S., Geim, A.K., Morozov, S.V., Jiang, D., Zhang, Y., Dubonos, S.V., Grigorieva, I.V., and Firsov, A.A.: Electric field effect in atomically thin carbon films. Science 306, 666–669 (2004).CrossRefGoogle ScholarPubMed
Bolotin, K.I., Sikes, K., Jiang, Z., Klima, M., Fudenberg, G., Hone, J., Kim, P., and Stormer, H.: Ultrahigh electron mobility in suspended graphene. Solid State Commun. 146, 351–355 (2008).CrossRefGoogle Scholar
Lee, C., Wei, X., Kysar, J.W., and Hone, J.: Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 321, 385–388 (2008).CrossRefGoogle ScholarPubMed
Kim, K.S., Zhao, Y., Jang, H., Lee, S.Y., Kim, J.M., Kim, K.S., Ahn, J-H., Kim, P., Choi, J-Y., and Hong, B.H.: Large-scale pattern growth of graphene films for stretchable transparent electrodes. Nature 457, 706 (2009).CrossRefGoogle ScholarPubMed
Özyilmaz, B., Jarillo-Herrero, P., Efetov, D., Abanin, D.A., Levitov, L.S., and Kim, P.: Electronic transport and quantum hall effect in bipolar graphene p-n-p junctions. Phys. Rev. Lett. 99, 166804 (2007).CrossRefGoogle ScholarPubMed
Bunch, J.S., Van Der Zande, A.M., Verbridge, S.S., Frank, I.W., Tanenbaum, D.M., Parpia, J.M., Craighead, H.G., and McEuen, P.L.: Electromechanical resonators from graphene sheets. Science 315, 490–493 (2007).CrossRefGoogle ScholarPubMed
Frank, I., Tanenbaum, D.M., van der Zande, A.M., and McEuen, P.L.: Mechanical properties of suspended graphene sheets. J. Vac. Sci. Technol., B: Microelectron. Nanometer Struct.–Process., Meas., Phenom. 25, 2558–2561 (2007).CrossRefGoogle Scholar
Zhang, T., Li, X., and Gao, H.: Fracture of graphene: A review. Int. J. Fract. 196, 1–31 (2015).CrossRefGoogle Scholar
Akinwande, D., Brennan, C.J., Scott Bunch, J., Egberts, P., Felts, J.R., Gao, H., Huang, R., Kim, J-S., Li, T., Li, Y., Liechti, K.M., Lu, N., Park, H.S., Reed, E.J., Wang, P., Yakobson, B.I., Zhang, T., Zhang, Y-W., Zhou, Y., and Zhu, Y.: A review on mechanics and mechanical properties of 2D materials—Graphene and beyond. Extreme Mech. Lett. 13, 42–77 (2017).CrossRefGoogle Scholar
Gupta, A., Sakthivel, T., and Seal, S.: Recent development in 2d materials beyond graphene. Prog. Mater. Sci. 73, 44–126 (2015).CrossRefGoogle Scholar
Le, M-Q. and Batra, R.C.: Single-edge crack growth in graphene sheets under tension. Comput. Mater. Sci. 69, 381–388 (2013).CrossRefGoogle Scholar
Orowan, E.: Fracture and strength of solids. Rep. Prog. Phys. 12, 185 (1949).CrossRefGoogle Scholar
Yin, H., Qi, H.J., Fan, F., Zhu, T., Wang, B., and Wei, Y.: Griffith criterion for brittle fracture in graphene. Nano Lett. 15, 1918–1924 (2015).CrossRefGoogle ScholarPubMed
Liu, X., Wang, F., and Wu, H.: Anisotropic propagation and upper frequency limitation of terahertz waves in graphene. Appl. Phys. Lett. 103, 071904 (2013).CrossRefGoogle Scholar
Liu, X., Wang, F., and Wu, H.: Anisotropic growth of buckling-driven wrinkles in graphene monolayer. Nanotechnology 26, 065701 (2015a).CrossRefGoogle Scholar
Liu, X., Wang, F., and Wu, H.: Anomalous twisting strength of tilt grain boundaries in armchair graphene nanoribbons. Phys. Chem. Chem. Phys. 17, 31911–31916 (2015b).CrossRefGoogle Scholar
Geim, A.K.: Graphene: Status and prospects. Science 324, 1530–1534 (2009).CrossRefGoogle ScholarPubMed
Lee, G-H., Cooper, R.C., An, S.J., Lee, S., Van Der Zande, A., Petrone, N., Hammerberg, A.G., Lee, C., Crawford, B., Oliver, W., Kysar, J.W., and Hone, J.: High-strength chemical-vapor–deposited graphene and grain boundaries. Science 340, 1073–1076 (2013).CrossRefGoogle ScholarPubMed
Grantab, R., Shenoy, V.B., and Ruoff, R.S.: Anomalous strength characteristics of tilt grain boundaries in graphene. Science 330, 946–948 (2010).CrossRefGoogle ScholarPubMed
Wang, M., Yan, C., Ma, L., Hu, N., and Chen, M.: Effect of defects on fracture strength of graphene sheets. Comput. Mater. Sci. 54, 236–239 (2012).CrossRefGoogle Scholar
López-Polín, G., Gómez-Herrero, J., and Gómez-Navarro, C.: Confining crack propagation in defective graphene. Nano Lett. 15, 2050–2054 (2015).CrossRefGoogle ScholarPubMed
Le, M-Q. and Batra, R.C.: Crack propagation in pre-strained single layer graphene sheets. Comput. Mater. Sci. 84, 238–243 (2014).CrossRefGoogle Scholar
Budarapu, P.R., Javvaji, B., Sutrakar, V., Roy Mahapatra, D., Zi, G., and Rabczuk, T.: Crack propagation in graphene. J. Appl. Phys. 118, 064307 (2015).CrossRefGoogle Scholar
Sen, D., Novoselov, K.S., Reis, P.M., and Buehler, M.J.: Tearing graphene sheets from adhesive substrates produces tapered nanoribbons. Small 6, 1108–1116 (2010).CrossRefGoogle ScholarPubMed
Moura, M.J. and Marder, M.: Tearing of free-standing graphene. Phys. Rev. E 88, 032405 (2013).CrossRefGoogle ScholarPubMed
Khare, R., Mielke, S.L., Paci, J.T., Zhang, S., Ballarini, R., Schatz, G.C., and Belytschko, T.: Coupled quantum mechanical/molecular mechanical modeling of the fracture of defective carbon nanotubes and graphene sheets. Phys. Rev. B 75, 075412 (2007).CrossRefGoogle Scholar
Zhang, P., Ma, L., Fan, F., Zeng, Z., Peng, C., Loya, P.E., Liu, Z., Gong, Y., Zhang, J., Zhang, X., Ajayan, P.M., Zhu, T., and Lou, J.: Fracture toughness of graphene. Nat. Commun. 5, 3782 (2014).CrossRefGoogle ScholarPubMed
Priester, L.: Grain Boundaries: From Theory to Engineering, Vol. 172 (Springer Science & Business Media, Dordrecht, 2012).Google Scholar
Zhang, T., Li, X., Kadkhodaei, S., and Gao, H.: Flaw insensitive fracture in nanocrystalline graphene. Nano Lett. 12, 4605–4610 (2012).CrossRefGoogle ScholarPubMed
Hirvonen, P., Ervasti, M.M., Fan, Z., Jalalvand, M., Seymour, M., Allaei, S.M.V., Provatas, N., Harju, A., Elder, K.R., and Ala-Nissila, T.: Multiscale modeling of polycrystalline graphene: A comparison of structure and defect energies of realistic samples from phase field crystal models. Phys. Rev. B 94, 035414 (2016).CrossRefGoogle Scholar
Plimpton, S.: Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 117, 1–19 (1995).CrossRefGoogle Scholar
Hossain, M., Hao, T., and Silverman, B.: Stillinger–weber potential for elastic and fracture properties in graphene and carbon nanotubes. J. Phys.: Condens. Matter 30, 055901 (2018a).Google Scholar
Hossain, M.Z., Ahmed, T., Silverman, B., Khawaja, M.S., Calderon, J., Rutten, A., and Tse, S.: Anisotropic toughness and strength in graphene and its atomistic origin. J. Mech. Phys. Solids 110, 118–136 (2018b).CrossRefGoogle Scholar
Stukowski, A.: Visualization and analysis of atomistic simulation data with ovito–the open visualization tool. Modell. Simul. Mater. Sci. Eng. 18, 015012 (2009).CrossRefGoogle Scholar
Xiao, S. and Belytschko, T.: A bridging domain method for coupling continua with molecular dynamics. Comput. Methods Appl. Mech. Eng. 193, 1645–1669 (2004).CrossRefGoogle Scholar
Terdalkar, S.S., Huang, S., Yuan, H., Rencis, J.J., Zhu, T., and Zhang, S.: Nanoscale fracture in graphene. Chem. Phys. Lett. 494, 218–222 (2010).CrossRefGoogle Scholar
Brakke, K.A.: Statistics of Random Plane Voronoi Tessellations (Department of Mathematical Sciences, Susquehanna University, Pennsylvania, 1987). (Manuscript 1987a).Google Scholar