Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-14T23:19:28.215Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical analysis of different methods to calculate residual stresses in thin films based on instrumented indentation data

Published online by Cambridge University Press:  01 June 2012

Carlos Eduardo Keutenedjian Mady ,
Sara Aida Rodriguez ,
Adriana Gómez Gómez  and
Roberto Martins Souza
Carlos Eduardo Keutenedjian Mady*
Affiliation:
Department of Mechanical Engineering, Polytechnic School of the University of São Paulo, 05508-900, São Paulo, Brazil
Sara Aida Rodriguez
Affiliation:
Department of Mechanical Engineering, Polytechnic School of the University of São Paulo, 05508-900, São Paulo, Brazil; and Research Group of Fatigue and Surface, Mechanical Engineering School, Universidad del, Valle, Cll 13 No 100-00 Cali, Colombia
Adriana Gómez Gómez
Affiliation:
Department of Mechanical Engineering, Polytechnic School of the University of São Paulo, 05508-900, São Paulo, Brazil; and Faculty of Engineering of Pontificia Universidad Javeriana Cali, Cll 18 No. 118-250 Cali, Colombia
Roberto Martins Souza
Affiliation:
Department of Mechanical Engineering, Polytechnic School of the University of São Paulo, 05508-900, São Paulo, Brazil
*
a)Address all correspondence to this author. e-mail: [email protected], [email protected]
Get access

Abstract

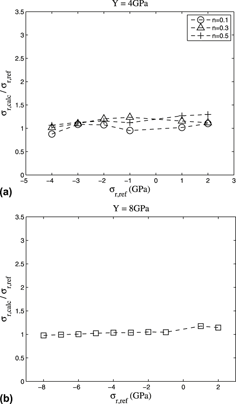
In this work, different methods to estimate the value of thin film residual stresses using instrumented indentation data were analyzed. This study considered procedures proposed in the literature, as well as a modification on one of these methods and a new approach based on the effect of residual stress on the value of hardness calculated via the Oliver and Pharr method. The analysis of these methods was centered on an axisymmetric two-dimensional finite element model, which was developed to simulate instrumented indentation testing of thin ceramic films deposited onto hard steel substrates. Simulations were conducted varying the level of film residual stress, film strain hardening exponent, film yield strength, and film Poisson’s ratio. Different ratios of maximum penetration depth hmax over film thickness t were also considered, including h/t = 0.04, for which the contribution of the substrate in the mechanical response of the system is not significant. Residual stresses were then calculated following the procedures mentioned above and compared with the values used as input in the numerical simulations. In general, results indicate the difference that each method provides with respect to the input values depends on the conditions studied. The method by Suresh and Giannakopoulos consistently overestimated the values when stresses were compressive. The method provided by Wang et al. has shown less dependence on h/t than the others.

Type
Articles
Information
Journal of Materials Research , Volume 27 , Issue 13 , 14 July 2012 , pp. 1732 - 1741
Copyright
Copyright © Materials Research Society 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

1.Tsui, T.Y., Oliver, W.C., and Pharr, G.M.: Influences of stress on the measurement of mechanical properties using nanoindentation: Part I. Experimental studies in an aluminum alloy. J. Mater. Res. 11, 752 (1996).CrossRefGoogle Scholar
2.Bolshakov, A., Oliver, W.C., and Pharr, G.M.: Influences of stress on the measurement of mechanical properties using nanoindentation: Part II. Finite element simulations. J. Mater. Res. 11, 760 (1996).CrossRefGoogle Scholar
3.Sines, G. and Carlson, R.: Hardness measurements for determination of residual stresses. ASTM Bull. 180, 35 (1952).Google Scholar
4.Oliver, W.C. and Pharr, G.M.: Improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 7, 1564 (1992).CrossRefGoogle Scholar
5.Oliver, W.C. and Pharr, G.M.: Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 19, 3 (2004).CrossRefGoogle Scholar
6.Mady, C.E.K., Rodriguez, S.A., Gomez, A.G., and Souza, R.M.: Effects of mechanical properties residual stress and indenter tip geometry on instrumented indentation data in thin films. Surf. Coat. Technol. 205, 1393 (2010).CrossRefGoogle Scholar
7.Larsson, P.: On the mechanical behavior at sharp indentation of materials with compressive residual stresses. Mater. Des. 32, 1427 (2011).CrossRefGoogle Scholar
8.Ling, L., Long, S., Ma, Z., and Liang, X.: Numerical study on the effects of equi-biaxial residual stress on mechanical properties of Nickel film by means of nanoindentation. J. Mater. Sci. Technol. 26, 1001 (2010).CrossRefGoogle Scholar
9.Jang, J.: Estimation of residual stress by instrumented indentation: A review. J. Ceram. Process. Res. 10, 391 (2009).Google Scholar
10.Suresh, S. and Giannakopoulos, A.E.: A new method for estimating residual stresses by instrumented sharp indentation. Acta Mater. 46, 5755 (1998).CrossRefGoogle Scholar
11.Atar, E., Sarioglu, C., Demirler, U., Sabri Kayali, E., and Cimenoglu, H.: Residual stress estimation of ceramic thin films by x-ray diffraction and indentation techniques. Scr. Mater. 48, 1331 (2003).CrossRefGoogle Scholar
12.Lee, Y.H. and Kwon, D.: Measurement of residual-stress effect by nanoindentation on elastically strained (100)W. Scr. Mater. 49, 459 (2003).CrossRefGoogle Scholar
13.Lee, Y.H. and Kwon, D.: Estimation of biaxial surface stress by instrumented indentation with sharp indenters. Acta Mater. 52, 1555 (2004).CrossRefGoogle Scholar
14.Carlsson, S. and Larsson, P.L.: On the determination of residual stress and strain fields by sharp indentation testing: Part I: Theoretical and numerical analysis. Acta Mater. 49, 2179 (2001).CrossRefGoogle Scholar
15.Carlsson, S. and Larsson, P.L.: On the determination of residual stress and strain fields by sharp indentation testing: Part II: Experimental investigation. Acta Mater. 49, 2193 (2001).CrossRefGoogle Scholar
16.Wang, Q., Ozaki, K., Ishikawa, H., Nakano, S., and Ogiso, H.: Indentation method to measure the residual stress induced by ion implantation. Nucl. Instrum. Methods Phys. Res. 242, 2823 (2006).CrossRefGoogle Scholar
17.Zhao, M., Chen, X., Yan, J., and Karlsson, A.: Determination of uniaxial residual stress and mechanical properties by instrumented indentation. Acta Mater. 54, 2823 (2006).CrossRefGoogle Scholar
18.Lee, J., Lee, H., Hyun, H., and Kim, M.: Numerical approaches and experimental verification of the conical indentation techniques for residual stress evaluation. J. Mater. Res. 25, 2212 (2010).CrossRefGoogle Scholar
19.Taljat, B. and Pharr, G.M.: Measurement of residual stresses by load and depth sensing spherical indentation, in Thin Films: Stresses and Mechanical Properties VIII, edited by Vinic, R., Kraft, O., Moody, N., and Shaffer, E. (Mater. Res. Soc. Symp. Proc. 594, Warrendale, PA, 2000), p. 519.Google Scholar
20.Swadener, J.G., Taljat, B., and Pharr, G.M.: Measurement of residual stress by load and depth sensing indentation with spherical indenters. J. Mater. Res. 16, 2091 (2001).CrossRefGoogle Scholar
21.Lepienski, C.M., Pharr, G.M., Park, Y.J., Watkins, T.R., Misra, A., and Zhang, X.: Factors limiting the measurement of residual stresses in thin films by nanoindentation. Thin Solid Films 447, 251 (2004).CrossRefGoogle Scholar
22.Choi, M., Lee, K., Kim, J., Kim, K., and Kwon, D.: Application of instrumented indentation technique to estimate strength and residual stress, in 10th Symposium on Recent Advancements in the Theory and Practice of Hardness Measurement (Hardmeko Proc. Tsukuba, Japan, 2007); p. 24.Google Scholar
23.Rodríguez Pulecio, S.A., Moré Farias, M.C., and Souza, R.M.: Analysis of the effects of conical indentation variables on the indentation response of elastic–plastic materials through factorial design of experiment. J. Mater. Res. 24, 1222 (2009).CrossRefGoogle Scholar
24.LaFontaine, W.R., Paszkiet, C.A., Korhonen, M.A., and Li, C-Y.: Residual stress measurements of thin aluminum metallizations by continuous indentation and x-ray stress measurement techniques. J. Mater. Res. 6, 2084 (1991).CrossRefGoogle Scholar