Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-30T21:08:10.316Z Has data issue: false hasContentIssue false
  • English
  • Français

Fiberwise KK-equivalence of continuous fields of C*-algebras

Published online by Cambridge University Press:  28 May 2008

Marius Dadarlat
Marius Dadarlat
Affiliation:
Department of Mathematics, Purdue University, West Lafayette IN 47907, U.S.A., [email protected].
Get access

Abstract

Let A and B be separable nuclear continuous C(X)-algebras over a finite dimensional compact metrizable space X. It is shown that an element σ of the parametrized Kasparov group KKX(A,B) is invertible if and only all its fiberwise components σx ∈ KK(A(x),B(x)) are invertible. This criterion does not extend to infinite dimensional spaces since there exist nontrivial unital separable continuous fields over the Hilbert cube with all fibers isomorphic to the Cuntz algebra . 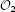Several applications to continuous fields of Kirchberg algebras are given. It is also shown that if each fiber of a separable nuclear continuous C(X)-algebra A over a finite dimensional locally compact space X satisfies the UCT, then A satisfies the UCT.

Keywords

Continuous fieldsK-theoryCuntz algebrasKirchberg algebras
Type
Research Article
Information
Journal of K-Theory , Volume 3 , Issue 2 , April 2009 , pp. 205 - 219
Copyright
Copyright © ISOPP 2009

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Bauval, A.. RKK(X)-nucléarité (d'après G. Skandalis). K-Theory 13 (1) (1998): 23–40CrossRefGoogle Scholar
2.Blackadar, B.. K-theory for operator algebras, volume 5 of Mathematical Sciences Research Institute Publications. Cambridge University Press, Cambridge, second edition, 1998Google Scholar
3.Blanchard, É.. Déformations de C*-algèbres de Hopf. Bull. Soc. Math. France 124 (1) (1996):141–215CrossRefGoogle Scholar
4.Blanchard, É.. Subtriviality of continuous fields of nuclear C*-algebras. J. Reine Angew. Math. 489 (1997) :133–149Google Scholar
5.Blanchard, É. and Kirchberg, E.. Global Glimm halving for C*-bundles. J. Oper. Theory 52 (2004):385–420Google Scholar
6.Blanchard, É. and Kirchberg, E.. Non-simple purely infinite C*-algebras: the Hausdorff case. J. Funct. Anal. 207 (2004):461–513CrossRefGoogle Scholar
7.Cuntz, J.. K-theory for certain C*-algebras. Ann. Math. 113 (1981):181–197CrossRefGoogle Scholar
8.Dadarlat, M.. Continuous fields of C*-algebras over finite dimensional spaces. arXiv:math.OA/0611405Google Scholar
9.Dadarlat, M.. The homotopy groups of the automorphism group of Kirchberg algebras. J. Noncomm. Geom. 1 (1) (2007):113–139CrossRefGoogle Scholar
10.Dadarlat, M.. Residually finite-dimensional C*-algebras. In Operator algebras and operator theory (Shanghai, 1997), volume 228 of Contemp. Math., pages 45–50. Amer. Math. Soc., Providence, RI, 1998CrossRefGoogle Scholar
11.Dadarlat, M.. Some remarks on the universal coefficient theorem in KK-theory. In Operator algebras and mathematical physics (Constanţa, 2001), 65–74. Theta, Bucharest, 2003Google Scholar
12.Dadarlat, M. and Pasnicu, C.. Continuous fields of Kirchberg C*-algebras. J. Funct. Anal., 226 (2005):429–451CrossRefGoogle Scholar
13.Dadarlat, M. and Winter, W.. Trivialization of C(X)-algebras with strongly selfabsorbing fibres. arXiv:math.OA/0705.1497Google Scholar
14.Fell, J. M. G.. The structure of algebras of operator fields. Acta Math., 106 (1961):233–280CrossRefGoogle Scholar
15.Fuchs, L.. Infinite abelian groups. vol. 1, Academic Press, New York and London, 1970Google Scholar
16.Hirshberg, I., Rørdam, M. and Winter, W.. C 0(X)-algebras, stability and strongly selfabsorbing C*-algebras. arXiv:math.OA/0610344Google Scholar
17.Husemoller, D.. Fibre Bundles. Number 20 in Graduate Texts in Mathematics. Springer Verlag, New York, 3rd. edition, 1966, 1994Google Scholar
18.Kasparov, G. G.. Equivariant KK-theory and the Novikov conjecture. Invent. Math., 91 (1) (1988) :147–201CrossRefGoogle Scholar
19.Kirchberg, E.. Das nicht-kommutative Michael-auswahlprinzip und die klassifikation nicht-einfacher algebren. In C*-algebras, 92–141, Berlin, 2000. Springer. (Münster, 1999)CrossRefGoogle Scholar
20.Kirchberg, E. and Rørdam, M.. Non-simple purely infinite C*-algebras. Amer. J. Math., 122 (3) (2000):637–666CrossRefGoogle Scholar
21.Kirchberg, E. and Rørdam, M.. Infinite non-simple C*-algebras: absorbing the Cuntz algebra O ∞. Advances in Math. 167 (2) (2002):195–264CrossRefGoogle Scholar
22.Lin, H.. Weak semiprojectivity in purely infinite C*-algebras. preprint 2002Google Scholar
23.Loring, T.. Lifting Solutions to Perturbing Problems in C*-Algebras, volume 8 of Fields Institute Monographs. Amer. Math. Soc., Providence, Rhode Island, 1997Google Scholar
24.Meyer, R. and Nest, R.. The Baum-Connes conjecture via localisation of categories. Topology 45 (2) (2006):209–259CrossRefGoogle Scholar
25.Rørdam, M.. Classification of nuclear, simple C*-algebras, volume 126 of Encyclopaedia Math. Sci. Springer, Berlin, 2002Google Scholar
26.Rosenberg, J. and Schochet, C.. The Künneth theorem and the universal coefficient theorem for Kasparov's generalized K-functor. Duke Math. J. 55 (2) (1987):431–474CrossRefGoogle Scholar
27.Schochet, C. L.. The UCT, the Milnor sequence, and a canonical decomposition of the Kasparov groups. K-Theory 10 (1)(1996):49–72CrossRefGoogle Scholar
28.Spielberg, J. S.. Semiprojectivity for certain purely infinite C*-algebras. arXiv:math.OA/0206185Google Scholar
29.Toms, A. and Winter, W.. Strongly self-absorbing C*-algebras. Trans. Amer. Math. Soc. 359 (2007):3999–4029CrossRefGoogle Scholar
30.Tu, J.-L.. La conjecture de Baum-Connes pour les feuilletages moyennables. K-Theory 17 (3) (1999):215–264CrossRefGoogle Scholar