Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-11-24T15:53:27.112Z Has data issue: false hasContentIssue false
  • English
  • Français

Weakly nonlinear shape oscillations of inviscid drops

Published online by Cambridge University Press:  22 July 2021

D. Zrnić  and
G. Brenn Open the ORCID record for G. Brenn [Opens in a new window]
D. Zrnić
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, Graz University of Technology, Inffeldgasse 25/F, 8010 Graz, Austria
G. Brenn*
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, Graz University of Technology, Inffeldgasse 25/F, 8010 Graz, Austria
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

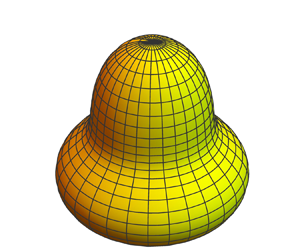
Nonlinear axisymmetric shape oscillations of an inviscid liquid drop in a vacuum are investigated theoretically for their relevance for transport processes across the drop surface. The weakly nonlinear approach is adopted as the theoretical method. For the two-lobed mode of deformation $m=2$, known nonlinear effects are an asymmetry of the times the drop spends in the oblate and prolate deformed states, and an oscillation frequency smaller than the linear one found by Rayleigh. The present analysis shows that, for $m=2$, the frequency decrease with increasing surface deformation of the droplet is a third-order effect. For higher deformation modes, the frequency decrease shows in the second-order approximation already. The analysis is carried out for modes of initial deformation up to $m=4$, but not limited to that. The nonlinearity is due to two different contributions: the coupling of different modes of deformation; and the forces from capillary pressure acting on different drop cross-sectional areas in different phases of the oscillation. For the two-lobed mode of deformation, at an aspect ratio of 1.5, the two effects reduce the oscillation frequency by 5 %. The present analysis represents the quasi-periodicity of nonlinear drop oscillations found by other authors in numerical simulations. The results show that drops in nonlinear oscillations at strong deformation may never reach the spherical shape, thus exhibiting a resultant increase of their surface area.

JFM classification

Drops and Bubbles: Drops Waves/Free-surface Flows: Capillary waves
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 923 , 25 September 2021 , A9
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alonso, C.T. 1974 The dynamics of colliding and oscillating drops. In Proceedings of the International Colloquium on Drops and Bubbles (ed. D.J. Collins, M.S. Plesset & M.M. Saffren). Jet Propulsion Laboratory.Google Scholar
Basaran, O.A. 1992 Nonlinear oscillations of viscous liquid drops. J. Fluid Mech. 241, 169–198.CrossRefGoogle Scholar
Becker, E., Hiller, W.J. & Kowalewski, T.A. 1991 Experimental and theoretical investigation of large-amplitude oscillations of liquid droplets. J. Fluid Mech. 231, 189–210.CrossRefGoogle Scholar
Bird, R.B., Stewart, W.E. & Lightfoot, E.N. 1962 Transport Phenomena. John Wiley & Sons.Google Scholar
Brenn, G. 2017 Analytical Solutions for Transport Processes. Springer.CrossRefGoogle Scholar
Chandrasekhar, S. 1959 The oscillations of a viscous liquid globe. Proc. Lond. Math. Soc. 9, 141–149.CrossRefGoogle Scholar
Dürr, H.M. & Siekmann, J. 1987 Numerical studies of fluid oscillation pproblem by boundary integral techniques. Acta Astronaut. 15 (11), 859–864.CrossRefGoogle Scholar
Foote, G.B. 1973 A numerical method for studying liquid drop behaviour: simple oscillation. J. Comput. Phys. 11, 507–530.CrossRefGoogle Scholar
Lamb, H. 1881 On the oscillations of a viscous spheroid. Proc. Lond. Math. Soc. 13, 51–66.CrossRefGoogle Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn. Cambridge University Press.Google Scholar
Lundgren, T.S. & Mansour, N.N. 1988 Oscillations of drops in zero gravity with weak viscous effects. J. Fluid Mech. 194, 479–510.CrossRefGoogle Scholar
Meradji, S., Lyubimova, T.P., Lyubimov, D.V. & Roux, B. 2001 Numerical simulations of a liquid drop freely oscillating. Cryst. Res. Technol. 36, 729–744.3.0.CO;2-3>CrossRefGoogle Scholar
Miller, C.A. & Scriven, L.E. 1968 The oscillations of a fluid drop immersed in another fluid. J. Fluid Mech. 32, 417–435.CrossRefGoogle Scholar
Natarajan, R. & Brown, R.A. 1987 Third-order resonance effects and the nonlinear stability of drop oscillations. J. Fluid Mech. 183, 95–121.CrossRefGoogle Scholar
Patzek, T.W., Benner, R.E., Basaran, O.A. & Scriven, L.E. 1991 Nonlinear oscillations of inviscid free drops. J. Comput. Phys. 97, 489–515.CrossRefGoogle Scholar
Prosperetti, A. 1980 a Free oscillations of drops and bubbles: the initial-value problem. J. Fluid Mech. 100, 333–347.CrossRefGoogle Scholar
Prosperetti, A. 1980 b Normal-mode analysis for the oscillations of a viscous liquid drop in an immiscible liquid. J. Méc. 19, 149–182.Google Scholar
Rayleigh, L. 1879 On the capillary phenomena of jets. Proc. R. Soc. Lond. A 29, 71–97.Google Scholar
Reid, W.H. 1960 The oscillations of a viscous liquid drop. Q. Appl. Maths 18 (1), 86–89.CrossRefGoogle Scholar
Renoult, M.-C., Brenn, G., Plohl, G. & Mutabazi, I. 2018 Weakly nonlinear instability of a newtonian liquid jet. J. Fluid Mech. 856, 169–201.CrossRefGoogle Scholar
Smith, W.R. 2010 Modulation equations for strongly nonlinear oscillations of an incompressible viscous drop. J. Fluid Mech. 654, 141–159.CrossRefGoogle Scholar
Trinh, E.H. & Wang, T.G. 1982 Large amplitude drop shape oscillations. In Proceedings of the 2nd International Colloquium on Drops and Bubbles, pp. 82–87. JPL Publication.Google Scholar
Tsamopoulos, J.A. 1990 Nonlinear dyamics and break-up of charged drops. AIP Conf. Proc. 197, 169–187.CrossRefGoogle Scholar
Tsamopoulos, J.A. & Brown, R.A. 1983 Nonlinear oscillations of inviscid drops and bubbles. J. Fluid Mech. 127, 519–537.CrossRefGoogle Scholar
Tsamopoulos, J.A. & Brown, R.A. 1984 Resonant oscillations of inviscid charged drops. J. Fluid Mech. 147, 373–395.CrossRefGoogle Scholar
Wang, T.G., Anilkumar, A.V. & Lee, C.P. 1996 Oscillations of liquid drops: results from USML-1 experiments in space. J. Fluid Mech. 308, 1–14.CrossRefGoogle Scholar
Yuen, M.-C. 1968 Non-linear capillary instability of a liquid jet. J. Fluid Mech. 33 (1), 151–163.CrossRefGoogle Scholar
Supplementary material: File

Zrnić and Brenn supplementary material

Zrnić and Brenn supplementary material

Download Zrnić and Brenn supplementary material(File)
File 147.5 KB