Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-24T15:36:34.010Z Has data issue: false hasContentIssue false
  • English
  • Français

Water entry without surface seal: extended cavity formation

Published online by Cambridge University Press:  04 March 2014

M. M. Mansoor ,
J. O. Marston ,
I. U. Vakarelski  and
S. T. Thoroddsen
M. M. Mansoor
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
J. O. Marston*
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
I. U. Vakarelski
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia Clean Combustion Research Centre, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
S. T. Thoroddsen
Affiliation:
Division of Physical Sciences and Engineering, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia Clean Combustion Research Centre, King Abdullah University of Science and Technology, Thuwal 23955-6900, Saudi Arabia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We report results from an experimental study of cavity formation during the impact of superhydrophobic spheres onto water. Using a simple splash-guard mechanism, we block the spray emerging during initial contact from closing thus eliminating the phenomenon known as ‘surface seal’, which typically occurs at Froude numbers 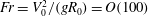$\mathit{Fr}= V_{0}^{2}/(gR_{0}) = O(100)$. As such, we are able to observe the evolution of a smooth cavity in a more extended parameter space than has been achieved in previous studies. Furthermore, by systematically varying the tank size and sphere diameter, we examine the influence of increasing wall effects on these guarded impact cavities and note the formation of surface undulations with wavelength 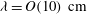$\lambda =O(10)~ \mathrm{cm}$ and acoustic waves $\lambda _{a}=O(D_{0})$ along the cavity interface, which produce multiple pinch-off points. Acoustic waves are initiated by pressure perturbations, which themselves are generated by the primary cavity pinch-off. Using high-speed particle image velocimetry (PIV) techniques we study the bulk fluid flow for the most constrained geometry and show the larger undulations (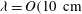$\lambda =O (10~ \mathrm{cm}$)) have a fixed nature with respect to the lab frame. We show that previously deduced scalings for the normalized (primary) pinch-off location (ratio of pinch-off depth to sphere depth at pinch-off time), 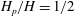$H_{p}/H = 1/2$, and pinch-off time, 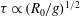$\tau \propto (R_{0}/g)^{1/2}$, do not hold for these extended cavities in the presence of strong wall effects (sphere-to-tank diameter ratio), 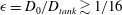$\epsilon = D_{0}/D_{tank} \gtrsim 1/16$. Instead, we find multiple distinct regimes for values of $H_{p}/H$ as the observed undulations are induced above the first pinch-off point as the impact speed increases. We also report observations of ‘kinked’ pinch-off points and the suppression of downward facing jets in the presence of wall effects. Surprisingly, upward facing jets emanating from first cavity pinch-off points evolve into a ‘flat’ structure at high impact speeds, both in the presence and absence of wall effects.

JFM classification

Drops and Bubbles: Cavitation Aerodynamics: High-speed flow Wakes/Jets: Jets
Type
Papers
Information
Journal of Fluid Mechanics , Volume 743 , 25 March 2014 , pp. 295 - 326
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aristoff, J. M. & Bush, J. W. M. 2009 Water entry of small hydrophobic spheres. J. Fluid Mech. 619, 45–78.Google Scholar
Aristoff, J. M., Truscott, T., Techet, A. H. & Bush, J. W. M. 2010 The water entry of decelerating spheres. Phys. Fluids. 22, 032102.Google Scholar
Bell, G. E. 1924 On the impact of a solid sphere with a fluid surface. Phil. Mag. J. Sci. 48, 753–765.CrossRefGoogle Scholar
Bergmann, R., Van der meer, D., Van der bos, A. & Lohse, D. 2009 Controlled impact of a disk on a water surface: cavity dynamics. J. Fluid Mech. 633, 381–409.Google Scholar
Birkhoff, G. & Isaacs, R. 1951 Transient cavities in air water entry, Tech. Rep. 1490 Navord Rep.Google Scholar
Burley, R. 1992 Air entrainment and the limits of coatability. JOCCA 75 (5), 192–202.Google Scholar
Duclaux, V., Caille, F., Duez, C., Ybert, C., Bocquet, L. & Clanet, C. 2007 Dynamics of transient cavities. J. Fluid Mech. 591, 1–19.Google Scholar
Duez, C., Ybert, C., Clanet, C. & Bocquet, L. 2007 Making a splash with water repellency. Nat. Phys. 3, 180–183.Google Scholar
Di Felice, R. 1996 A relationship for the wall effect on the settling velocity of a sphere at any flow regime. Int. J. Multiphase Flow 22, 527–533.Google Scholar
Gekle, S., Gordillo, J. M., Van der meer, D. & Lohse, D. 2009 High-speed jet formation after solid object impact. Phys. Rev. Lett. 102, 034502.Google Scholar
Gekle, S., Peters, I. R., Gordillo, J. M., Van der meer, D. & Lohse, D. 2010 Supersonic air flow due to solid–liquid impact. Phys. Rev. Lett. 104, 024501.Google Scholar
Gekle, S., Van der bos, A., Bergmann, R., Van der Meer, D. & Lohse, D. 2008 Noncontinuous Froude number scaling for the closure depth of a cylindrical cavity. Phys. Rev. Lett. 100, 084502.Google Scholar
Gilbarg, D. & Anderson, R. 1948 Influence of atmospheric pressure on the phenomena accompanying the entry of spheres into water. J. Appl. Phys. 19, 127–139.CrossRefGoogle Scholar
Glasheen, J. W. & McMahon, T. A. 1996 A hydrodynamical model of locomotion in the basilisk lizard. Nature 380, 340–342.Google Scholar
Grumstrup, T., Keller, J. B. & Belmonte, A. 2007 Cavity ripples observed during the impact of solid objects into liquids. Phys. Rev. Lett. 99, 114502.Google Scholar
Lee, M., Longoria, R. G. & Wilson, D. E. 1997 Cavity dynamics in high-speed water entry. Phys. Fluids. 9 (3), 540–550.CrossRefGoogle Scholar
Mallock, A. 1918 Sounds produced by drops falling on water. Proc. R. Soc. Lond. A 95, 138–143.Google Scholar
Marston, J. O., Vakarelski, I. U. & Thoroddsen, S. T. 2011 Bubble entrapment during sphere impact onto quiescent liquid surfaces. J. Fluid Mech. A 680, 660–670.CrossRefGoogle Scholar
Marston, J. O., Vakarelski, I. U. & Thoroddsen, S. T. 2012a Cavity formation by the impact of Leidenfrost spheres. J. Fluid Mech. 699, 465–488.Google Scholar
Marston, J. O., Zhu, Y., Vakarelski, I. U. & Thoroddsen, S. T. 2012b Deformed liquid marbles: freezing drop oscillations with powders. Powder Tech. 228, 424–428.CrossRefGoogle Scholar
May, A. 1951 Effect of surface condition of a sphere on its water-entry cavity. J. Appl. Phys. 22, 1219–1222.Google Scholar
May, A. 1952 Vertical entry of missiles into water. J. Appl. Phys. 22, 1362–1372.Google Scholar
May, A. & Hoover, W. R.1963 A study of the water-entry cavity. Unclassified NOLTR 63-264. United States Naval Ordinance Laboratory, White Oak, MD.Google Scholar
May, A. & Woodhull, J. C. 1948 Drag coefficients of steel spheres entering water vertically. J. Appl. Phys. 19, 1109–1121.Google Scholar
May, A. & Woodhull, J. C. 1950 The virtual mass of a sphere entering water vertically. J. Appl. Phys. 21, 1285–1289.Google Scholar
Metzger, M. A.1981 A computer program for modeling water entry cavity performance and comparison of predicted with observed cavities. Naval Surface Weapons Center, Silver Springs, MD, Report No. NSWC/TR-81-59.Google Scholar
Ramsauer, C. & Dobke, G. 1927 Die Bewegungserscheinungen des Wassers beim Durchgang schnell bewegter Kugeln. Ann. Phys. Lpz. 389, 697–720.CrossRefGoogle Scholar
Richardson, E. G. 1948 The impact of a solid on a liquid surface. Proc. Phys. Soc. 61, 352–367.CrossRefGoogle Scholar
Rosellini, L., Hersen, F., Clanet, C. & Bocquet, L. 2005 Skipping stones. J. Fluid Mech. 543, 137–146.Google Scholar
Royer, J. R., Corwin, E. I., Conyers, B., Flior, A., Rivers, M. L., Eng, P. J. & Jaeger, H. M. 2008 Birth and growth of a granular jet. Phys. Rev. E 78, 011305.Google Scholar
Shi, H. H., Itoh, M. & Takami, T. 2000 Optical observation of the supercavitation induced by high-speed water entry. Trans. ASME 122, 806–810.Google Scholar
Thoroddsen, S. T., Etoh, T. G., Takehara, K. & Takano, Y. 2004 Impact jetting by a solid sphere. J. Fluid Mech. 499, 139–148.CrossRefGoogle Scholar
Thoroddsen, S. T., Etoh, T. G. & Takehara, K. 2008 High-speed imaging of drops and bubbles. Annu. Rev. Fluid Mech 40, 257–285.Google Scholar
Truscott, T. T. & Techet, A. H. 2009 Water entry of spinning spheres. J. Fluid Mech. 625, 135–165.Google Scholar
Vakarelski, I. U., Marston, J. O., Chan, D. Y. C. & Thoroddsen, S. T. 2011 Drag reduction by Leidenfrost vapor layers. Phys. Rev. Lett. 106, 214501.Google Scholar
Vakarelski, I. U., Patankar, N. A., Marston, J. O., Chan, D. Y. C. & Thoroddsen, S. T. 2012 Stabilization of Leidenfrost vapour layer by textured superhydrophobic surfaces. Nature 489, 274–277.Google Scholar
Von Kann, S., Joubaud, S., Caballero-Robledo, G. A., Lohse, D. & Van der Meer, D. 2010 Effect of finite container size on granular jet formation. Phys. Rev. E 81, 041306.Google Scholar
Worthington, A. M. 1908 A study of splashes. Longmans Green.Google Scholar
Worthington, A. M. & Cole, R. S. 1897 Impact with a liquid surface, studied by the aid of instantaneous photography. Phil. Trans. R. Soc. Lond. A 189, 137–148.Google Scholar
Worthington, A. M. & Cole, R. S. 1900 Impact with a liquid surface, studied by the aid of instantaneous photography: paper 2. Phil. Trans. R. Soc. Lond. A 194, 175–1499.Google Scholar

Mansoor et al. supplementary movie

Comparison of “free” and “guarded” impact cavities formed during the impact of a 20 mm diameter sphere onto water at 8.4 m/s. The left panel is for free impact, where surface seal occurs, whilst the right panel shows the guarded impact, where surface seal is inhibited. Fr = 720, We = 9800, ε = 0.04, Bo = 13.6, Cn = 2.8. Original frame rate used was 10000 fps, playback speed is 30 fps showing only every 10th frame.

Download Mansoor et al. supplementary movie(Video)
Video 7.4 MB

Mansoor et al. supplementary movie

Comparison of “guarded” impact cavities formed during the impact of a 15 mm diameter sphere onto water under severe wall effects using a 8 cm square tank for various impact speeds of 2.8, 4.4, 6.4 and 8.4 m/s. Fr = 107 - 959, ε = 0.19, Bo = 7.6. Original frame rate used was 10000 fps, playback speed is 30 fps showing only every other frame.

Download Mansoor et al. supplementary movie(Video)
Video 9.5 MB

Mansoor et al. supplementary movie

Close-up view of the cavity pinch-off after the impact of a 20 mm diameter sphere onto water at 8.4 m/s under moderate wall effects using a 20 cm square tank. Fr = 720, ε = 0.1, Bo = 13.6. Original frame rate used was 10000 fps, playback speed is 30 fps showing only every 4th frame.

Download Mansoor et al. supplementary movie(Video)
Video 9.6 MB