Article contents
Viscous flow around three-dimensional macroscopic cavities in a granular material
Published online by Cambridge University Press: 26 November 2021
Abstract
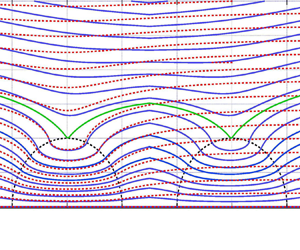
Viscous flow around spherical macroscopic cavities in a granular material is investigated. The Stokes equation inside and the Darcy–Brinkman equation outside the cavities are considered. In particular, the interaction of two equally sized cavities positioned in tandem is examined in detail, where the asymptotic effect of the other cavity is taken into account. The present analysis gives a reasonable estimate on the volume flow into the cavity and the local enhancement of stresses. This is applicable to predict the microscale waterway formation in that material, onset of landslides, collapse of cliffs and river banks, etc.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 4
- Cited by



