Unsteady flow adjacent to an oscillating or impulsively started porous wall

Abstract

Kang et al. (J. Fluid Mech., vol. 874, 2019, pp. 339–358) studied viscous dissipation within a permeable body with a view to maximizing the damping of oscillations of the body. They found that dissipation is maximal when the length scale for diffusion of vorticity in the fluid outside the body is similar to the length scale for decay of fluid motion within the body. Their results are examined in the context of the simpler problem of a porous half-space oscillating parallel to the interface between porous solid and fluid. The analysis is then extended to consider the impulsive start-up from rest of a porous plane surface adjacent to unbounded fluid.

1 Introduction

Interactions between flowing fluid and permeable solids are of interest over a wide range of problems at different length scales, including the motion of coiled polymer molecules (Felderhof 2014), the flow of blood adjacent to body tissue, and the absorption of wave energy by dykes built as piles of boulders rather than as solid walls (Chwang & Chan 1998). Kang et al. (2019) recently studied viscous energy losses inside oscillating permeable spheres and cylinders (both cylindrical and elliptical). The energy loss (and hence the degree of damping) can be maximized by choosing the permeability of the porous body to be such that the length scale for decay of fluid motion inside the porous body is comparable to the length scale for decay of vorticity outside the body.

Our aim here is to shed further light on the energy analysis of Kang et al. (2019) by considering a much simpler problem. The flow created by a rigid plane boundary oscillating in its own plane, first studied by Stokes (1851) (and known as Stokes’ second problem) is a standard textbook example taught to undergraduates (Batchelor 1973): here, we extend the analysis, replacing the boundary by a permeable half-space. For completeness, we then look at Stokes’ problem of the impulsive start-up from rest of a rigid plane moving in its own plane, and similarly replace the boundary by a permeable half-space.

The two-domain approach to studying hydrodynamic interactions between a porous body and surrounding fluid uses Darcy’s law to predict fluid motion within the porous body and the Navier–Stokes equations in the external fluid (Chwang & Chan 1998), but one then has to decide upon appropriate boundary conditions at the interface between the permeable body and external fluid. Beavers & Joseph (1967) showed that a tangential slip boundary condition can be more appropriate than no slip, though when slip is allowed the choice of the normal stress boundary condition is less obvious, since viscous normal stresses may be non-zero outside the body (Sherwood 1990) when the tangential velocity is non-uniform. The jump in normal stress has been discussed by e.g. Ochoa-Tapia & Whitaker (1995), Marciniak-Czochra & Mikelić (2012), Valdés-Parada et al. (2013) and Carraro et al. (2013). An alternative one-domain approach uses a single governing equation with material properties that are functions of position. The two approaches are compared by Valdés-Parada et al. (2013). As an alternative to Darcy’s law, Brinkman (1947) suggested that the effect of the solid matrix within the permeable medium can be modelled as a forcing term in the Navier–Stokes equations, with strength inversely proportional to the permeability (and therefore zero outside the medium), i.e. the permeable medium and adjacent fluid are treated as a single domain. This approach allows us to assume continuity of fluid velocity and stress at the interface.

Here we adopt a one-domain approach based on Brinkman’s equation within the porous medium, in part because it provides a simple method to illustrate the effect of permeability on the flows considered by Stokes, but also because this was the approach adopted by Kang et al. (2019), whose work was the motivation for the studies presented here. Brinkman’s equation makes no attempt to include details of the flow within the tortuous pore space of the permeable medium (Scheidegger 1957), and neglects any nonlinear inertial contributions to fluid inertia which are present at the pore scale even if the average, Darcy velocity is uniform. This neglect means that it is most likely to be appropriate in the limit of zero solids volume fraction, i.e. porosity $\unicode[STIX]{x1D719}=1$ (Auriault 2009).

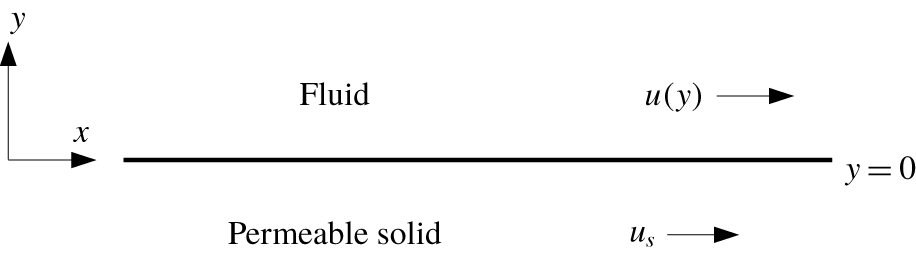
Figure 1. The region $y>0$ is occupied by fluid; the region $y<0$ is occupied by a permeable solid that oscillates in the $x$ direction with velocity $\tilde{u} _{s}\text{e}^{\text{i}\unicode[STIX]{x1D714}\tilde{t}}$ .

2 The oscillating plane permeable wall

The problem is two-dimensional, as depicted in figure 1. The region ${\tilde{y}}>0$ is occupied by fluid, and the region ${\tilde{y}}<0$ by a fluid-filled permeable solid that oscillates in the $\tilde{x}$ direction with velocity given by (the real part of) $\tilde{\boldsymbol{u}}_{s}=\tilde{u} _{s}\text{e}^{\text{i}\unicode[STIX]{x1D714}\tilde{t}}\hat{\boldsymbol{x}}$ , where $\unicode[STIX]{x1D714}$ is the angular frequency and $\tilde{t}$ is time. The resulting fluid velocity $\tilde{u} \text{e}^{\text{i}\unicode[STIX]{x1D714}\tilde{t}}$ is also in the $\tilde{x}$ direction, with $\tilde{u} \rightarrow 0$ as ${\tilde{y}}\rightarrow \infty$ , and $\tilde{u}$ tending to a constant as ${\tilde{y}}\rightarrow -\infty$ . Thus we have rectilinear flow, and nonlinear terms $\tilde{\boldsymbol{u}}\boldsymbol{\cdot }\unicode[STIX]{x1D735}\tilde{\boldsymbol{u}}$ in the Navier–Stokes equation are zero. The fluid has density $\unicode[STIX]{x1D70C}$ and viscosity $\unicode[STIX]{x1D707}$ . In the upper (fluid) half-space, the fluid velocity $\tilde{u}$ and pressure $\tilde{p}$ satisfy

(2.1) $$\begin{eqnarray}\unicode[STIX]{x1D70C}\frac{\unicode[STIX]{x2202}\tilde{u} }{\unicode[STIX]{x2202}\tilde{t}}=\unicode[STIX]{x1D707}\frac{\unicode[STIX]{x2202}^{2}\tilde{u} }{\unicode[STIX]{x2202}{\tilde{y}}^{2}}-\frac{\unicode[STIX]{x2202}\tilde{p}}{\unicode[STIX]{x2202}\tilde{x}},\quad {\tilde{y}}>0.\end{eqnarray}$$

The fluid is at rest far from the porous solid, with $\tilde{u}$ and $\unicode[STIX]{x2202}\tilde{p}/\unicode[STIX]{x2202}\tilde{x}$ tending to zero as ${\tilde{y}}\rightarrow \infty$ . The ${\tilde{y}}$ component of the Navier–Stokes equations tells us that $\unicode[STIX]{x2202}\tilde{p}/\unicode[STIX]{x2202}{\tilde{y}}=0$ , and we conclude that $\unicode[STIX]{x1D735}p=0$ everywhere in ${\tilde{y}}>0$ .

In the lower half-space, the fluid velocity is again assumed to be solely in the $\tilde{x}$ direction. The solid matrix has porosity $\unicode[STIX]{x1D719}$ and the mean fluid velocity within the pores is $\langle \tilde{u} \rangle$ (where the average is taken over only the pore space). We set $\tilde{u} _{D}=\unicode[STIX]{x1D719}\langle \tilde{u} \rangle$ , so that when the solid is at rest $\tilde{u} _{D}$ denotes the Darcy superficial velocity within the porous medium.

Brinkman (1947) modified Darcy’s equation by adding a viscous term $\unicode[STIX]{x1D707}_{B}\unicode[STIX]{x1D6FB}^{2}\boldsymbol{u}_{D}$ . There is no reason to suppose $\unicode[STIX]{x1D707}_{B}=\unicode[STIX]{x1D707}$ (as discussed in appendix A), but in §§ 2 and 3 we assume $\unicode[STIX]{x1D719}=1$ , in which case $\tilde{u} _{D}=\langle \tilde{u} \rangle =\tilde{u}$ and it is generally accepted that $\unicode[STIX]{x1D707}_{B}=\unicode[STIX]{x1D707}$ is a reasonable assumption. We add a linear inertial term to obtain a Brinkman equation of the form (when the solid matrix is at rest)

(2.2) $$\begin{eqnarray}\unicode[STIX]{x1D70C}\frac{\unicode[STIX]{x2202}\tilde{u} _{D}}{\unicode[STIX]{x2202}\tilde{t}}=\unicode[STIX]{x1D707}_{B}\frac{\unicode[STIX]{x2202}^{2}\tilde{u} _{D}}{\unicode[STIX]{x2202}{\tilde{y}}^{2}}-\frac{\unicode[STIX]{x2202}\tilde{p}}{\unicode[STIX]{x2202}\tilde{x}}-\frac{\unicode[STIX]{x1D707}}{k}\tilde{u} _{D},\quad {\tilde{y}}<0,\end{eqnarray}$$

where $k$ is the Darcy permeability of the porous medium.

When the solid moves with velocity $\tilde{u} _{s}$ , the mean velocity of the fluid relative to the solid matrix is $\langle \tilde{u} \rangle -\tilde{u} _{s}$ and the Darcy drag term is modified to become

(2.3) $$\begin{eqnarray}\unicode[STIX]{x1D70C}\frac{\unicode[STIX]{x2202}\tilde{u} _{D}}{\unicode[STIX]{x2202}\tilde{t}}=\unicode[STIX]{x1D707}_{B}\frac{\unicode[STIX]{x2202}^{2}\tilde{u} _{D}}{\unicode[STIX]{x2202}{\tilde{y}}^{2}}-\frac{\unicode[STIX]{x2202}\tilde{p}}{\unicode[STIX]{x2202}\tilde{x}}-\frac{\unicode[STIX]{x1D707}}{k}(\tilde{u} _{D}-\unicode[STIX]{x1D719}\tilde{u} _{s}),\quad {\tilde{y}}<0,\end{eqnarray}$$

where $\tilde{u} _{D}=\unicode[STIX]{x1D719}\langle \tilde{u} \rangle$ denotes the scaled fluid velocity in the laboratory frame, rather than a Darcy velocity relative to the solid matrix. In the main body of this paper we assume $\unicode[STIX]{x1D719}=1$ , so that $\tilde{u} _{D}=\langle \tilde{u} \rangle =\tilde{u}$ and $\unicode[STIX]{x1D707}_{B}=\unicode[STIX]{x1D707}$ . (We shall relax the assumption $\unicode[STIX]{x1D719}=1$ in appendix A.) The governing equation (2.3) therefore becomes

(2.4) $$\begin{eqnarray}\unicode[STIX]{x1D70C}\frac{\unicode[STIX]{x2202}\tilde{u} }{\unicode[STIX]{x2202}\tilde{t}}=\unicode[STIX]{x1D707}\frac{\unicode[STIX]{x2202}^{2}\tilde{u} }{\unicode[STIX]{x2202}{\tilde{y}}^{2}}-\frac{\unicode[STIX]{x2202}\tilde{p}}{\unicode[STIX]{x2202}\tilde{x}}-\frac{\unicode[STIX]{x1D707}}{k}(\tilde{u} -\tilde{u} _{s}),\quad {\tilde{y}}<0,\end{eqnarray}$$

and $\tilde{f}=\unicode[STIX]{x1D707}(\tilde{u} _{s}-\tilde{u} )/k$ is the force per unit volume acting on the fluid due to the Darcy resistance to flow through the porous medium, with $\tilde{f}=0$ in ${\tilde{y}}>0$ .

The rectilinear nature of the flow in ${\tilde{y}}<0$ ensures (as in ${\tilde{y}}>0$ ) that $\tilde{\boldsymbol{u}}\boldsymbol{\cdot }\unicode[STIX]{x1D735}\tilde{\boldsymbol{u}}$ terms are absent from Brinkman’s equation of motion, and we conclude from the ${\tilde{y}}$ component of the equation that $\unicode[STIX]{x2202}\tilde{p}/\unicode[STIX]{x2202}{\tilde{y}}=0$ , as in the upper half-plane.

As is usual in one-domain models, we assume that the fluid velocity $\tilde{u}$ , shear stress $\unicode[STIX]{x1D707}\unicode[STIX]{x2202}\tilde{u} /\unicode[STIX]{x2202}{\tilde{y}}$ and pressure $\tilde{p}$ are continuous at $y=0$ . There are therefore no pressure gradients in this uni-directional flow, so from henceforth we set $\tilde{p}=0$ . If we multiply the governing equations (2.1) and (2.4) by $\tilde{u}$ and integrate (2.4) over $-\tilde{L}<{\tilde{y}}<0$ and (2.1) over $0<{\tilde{y}}<\tilde{M}$ , we obtain the energy equation

(2.5) $$\begin{eqnarray}\displaystyle & & \displaystyle \unicode[STIX]{x1D707}\int _{-\tilde{L}}^{\tilde{M}}\left(\frac{\unicode[STIX]{x2202}\tilde{u} }{\unicode[STIX]{x2202}{\tilde{y}}}\right)^{2}\,\text{d}{\tilde{y}}+\unicode[STIX]{x1D70C}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}\tilde{t}}\int _{-\tilde{L}}^{\tilde{M}}\frac{\tilde{u} ^{2}}{2}\,\text{d}{\tilde{y}}-\int _{-\tilde{L}}^{0}(\tilde{u} -\tilde{u} _{s})\tilde{f}\,\text{d}{\tilde{y}}\nonumber\\ \displaystyle & & \displaystyle \quad =\unicode[STIX]{x1D707}\tilde{u} \left.\frac{\unicode[STIX]{x2202}\tilde{u} }{\unicode[STIX]{x2202}{\tilde{y}}}\right|_{{\tilde{y}}=\tilde{M}}-\unicode[STIX]{x1D707}\tilde{u} \left.\frac{\unicode[STIX]{x2202}\tilde{u} }{\unicode[STIX]{x2202}{\tilde{y}}}\right|_{{\tilde{y}}=-\tilde{L}}+\tilde{u} _{s}\int _{-\tilde{L}}^{0}\tilde{f}\,\text{d}{\tilde{y}}.\end{eqnarray}$$

The right-hand side of (2.5) represents the rate of input of energy by the solid matrix in ${\tilde{y}}<0$ as it moves with velocity $\tilde{u} _{s}$ , together with any work performed at the upper and lower boundaries ${\tilde{y}}=\tilde{M}$ , ${\tilde{y}}=-\tilde{L}$ . These boundary terms tend to zero as we allow $\tilde{L}$ and $\tilde{M}$ to tend to infinity. The left-hand side of (2.5) represents viscous dissipation due to shear within both the permeable medium and the adjacent fluid, the rate of change of kinetic energy of the fluid, and the rate at which the motion of the fluid relative to the solid matrix performs work. The rate at which the solid performs work on the liquid determines the rate of damping of interest to Kang et al. (2019), and we therefore direct our attention to this final term on the right-hand side of (2.5).

We look for oscillatory solutions in which all quantities vary as $\text{e}^{\text{i}\unicode[STIX]{x1D714}\tilde{t}}$ , and from now on drop explicit mention of this exponential factor. We scale time by $\unicode[STIX]{x1D714}^{-1}$ , lengths in the $y$ direction by $L_{d}=(\unicode[STIX]{x1D707}/\unicode[STIX]{x1D70C}\unicode[STIX]{x1D714})^{1/2}$ (the length scale for diffusion of vorticity), velocities by $\tilde{u} _{s}$ , stress by $\unicode[STIX]{x1D707}\tilde{u} _{s}/L_{d}$ and force per unit volume by $\unicode[STIX]{x1D707}\tilde{u} _{s}/L_{d}^{2}$ , and we denote non-dimensional quantities by the absence of a tilde. There are no pressure gradients. The governing equations become

(2.6a ) $$\begin{eqnarray}\displaystyle & \displaystyle \text{i}u=\frac{\unicode[STIX]{x2202}^{2}u}{\unicode[STIX]{x2202}y^{2}},\quad y>0, & \displaystyle\end{eqnarray}$$

(2.6b ) $$\begin{eqnarray}\displaystyle & \displaystyle \text{i}u=\frac{\unicode[STIX]{x2202}^{2}u}{\unicode[STIX]{x2202}y^{2}}-\unicode[STIX]{x1D6FE}(u-1),\quad y<0, & \displaystyle\end{eqnarray}$$

where

(2.7) $$\begin{eqnarray}\unicode[STIX]{x1D6FE}=\frac{L_{d}^{2}}{k}=\frac{\unicode[STIX]{x1D707}}{\unicode[STIX]{x1D70C}\unicode[STIX]{x1D714}k}.\end{eqnarray}$$

The solution of this pair of equations is straightforward, with

(2.8a ) $$\begin{eqnarray}\displaystyle u & = & \displaystyle U_{1}\exp \left(-\text{i}^{1/2}y\right),\quad y>0\end{eqnarray}$$

(2.8b ) $$\begin{eqnarray}\displaystyle & = & \displaystyle \frac{\unicode[STIX]{x1D6FE}}{\unicode[STIX]{x1D6FE}+\text{i}}+U_{2}\exp \left((\unicode[STIX]{x1D6FE}+\text{i})^{1/2}y\right),\quad y<0,\end{eqnarray}$$

where $\text{i}^{1/2}$ and $(\unicode[STIX]{x1D6FE}+\text{i})^{1/2}$ are chosen to have positive real part. Continuity of the fluid velocity and shear stress at $y=0$ requires

(2.9a,b ) $$\begin{eqnarray}U_{1}=\frac{\unicode[STIX]{x1D6FE}}{\unicode[STIX]{x1D6FE}+\text{i}+(\unicode[STIX]{x1D6FE}+\text{i})^{1/2}\text{i}^{1/2}},\quad U_{2}=\frac{-\text{i}^{1/2}\unicode[STIX]{x1D6FE}}{(\unicode[STIX]{x1D6FE}+\text{i})^{3/2}+(\unicode[STIX]{x1D6FE}+\text{i})\text{i}^{1/2}}.\end{eqnarray}$$

The non-dimensional force that the solid exerts on the fluid is $f=\unicode[STIX]{x1D6FE}(1-u)$ per unit volume, so that the rate of working of the solid as it oscillates with non-dimensional velocity $\text{Re}\{\text{e}^{\text{i}t}\}$ is

(2.10) $$\begin{eqnarray}W(y,t)=\unicode[STIX]{x1D6FE}\text{Re}\left\{\left[1-\frac{\unicode[STIX]{x1D6FE}}{\unicode[STIX]{x1D6FE}+\text{i}}-U_{2}\exp \left((\unicode[STIX]{x1D6FE}+\text{i})^{1/2}y\right)\right]\text{e}^{\text{i}t}\right\}\text{Re}\left\{\text{e}^{\text{i}t}\right\}\end{eqnarray}$$

and the mean rate of working is

(2.11) $$\begin{eqnarray}\overline{W}(y)=\frac{1}{2\unicode[STIX]{x03C0}}\int _{0}^{2\unicode[STIX]{x03C0}}W(y,t)\,\text{d}t=\frac{\unicode[STIX]{x1D6FE}}{2(\unicode[STIX]{x1D6FE}^{2}+1)}-\frac{\unicode[STIX]{x1D6FE}}{2}\text{Re}\left\{U_{2}\exp ((\unicode[STIX]{x1D6FE}+\text{i})^{1/2}y)\right\}.\end{eqnarray}$$

Fluid inertia prevents the pore fluid from moving with the velocity of the solid, so that, by (2.8b ), $u\rightarrow \unicode[STIX]{x1D6FE}/(\unicode[STIX]{x1D6FE}+\text{i})$ as $y\rightarrow -\infty$ , representing a fluid velocity that oscillates out of phase relative to the solid matrix. As a result, the rate of working of the solid matrix, per unit volume, tends to a non-zero constant as $y\rightarrow -\infty$ . The depth-integrated mean rate of working of the solid in the region $-L<y<0$ , with $L\gg \unicode[STIX]{x1D6FE}^{-1/2}$ , is

(2.12) $$\begin{eqnarray}\overline{V}=\int _{-L}^{0}\overline{W}(y)\,\text{d}y\sim \frac{F_{1}(\unicode[STIX]{x1D6FE})}{2}L+\frac{F_{2}(\unicode[STIX]{x1D6FE})}{2}+\cdots \!,\quad L\rightarrow \infty ,\end{eqnarray}$$

where

(2.13) $$\begin{eqnarray}F_{1}(\unicode[STIX]{x1D6FE})=\frac{\unicode[STIX]{x1D6FE}}{\unicode[STIX]{x1D6FE}^{2}+1}\end{eqnarray}$$

and

(2.14) $$\begin{eqnarray}F_{2}(\unicode[STIX]{x1D6FE})=-\unicode[STIX]{x1D6FE}\text{Re}\left\{\int _{-\infty }^{0}U_{2}\exp \left((\unicode[STIX]{x1D6FE}+\text{i})^{1/2}y\right)\,\text{d}y\right\}=\text{Re}\left\{\frac{\text{i}^{1/2}\unicode[STIX]{x1D6FE}^{2}}{(\unicode[STIX]{x1D6FE}+\text{i})^{2}+(\unicode[STIX]{x1D6FE}+\text{i})^{3/2}\text{i}^{1/2}}\right\}.\end{eqnarray}$$

In dimensional form, the total mean rate of working (per unit length in the $x$ direction) by the solid matrix is

(2.15) $$\begin{eqnarray}\tilde{\overline{V}}\sim \frac{\unicode[STIX]{x1D707}\tilde{u} _{s}^{2}}{2L_{d}}\left[F_{1}(\unicode[STIX]{x1D6FE})L+F_{2}(\unicode[STIX]{x1D6FE})\right]=\frac{\tilde{u} _{s}^{2}}{2}\left(\unicode[STIX]{x1D70C}\unicode[STIX]{x1D714}\unicode[STIX]{x1D707}\right)^{1/2}\left[F_{1}(\unicode[STIX]{x1D6FE})\tilde{L}\left(\frac{\unicode[STIX]{x1D70C}\unicode[STIX]{x1D714}}{\unicode[STIX]{x1D707}}\right)^{1/2}+F_{2}(\unicode[STIX]{x1D6FE})\right].\end{eqnarray}$$

If we allow the permeability $k$ to tend to zero, so that $\unicode[STIX]{x1D6FE}=L_{d}^{2}/k\rightarrow \infty$ , then

(2.16a,b ) $$\begin{eqnarray}F_{1}(\unicode[STIX]{x1D6FE})\sim \unicode[STIX]{x1D6FE}^{-1},\quad F_{2}(\unicode[STIX]{x1D6FE})\sim \frac{1}{2^{1/2}}\left(1+\frac{1}{\unicode[STIX]{x1D6FE}}-\frac{6}{(2\unicode[STIX]{x1D6FE})^{3/2}}+\cdots \!\right),\end{eqnarray}$$

and as $\unicode[STIX]{x1D6FE}\rightarrow \infty$ the total rate of working tends towards the classical result for the rate of dissipation in fluid adjacent to an oscillating plane impermeable wall. In this limit, fluid within the permeable solid moves at almost the same velocity as the solid, except within a boundary layer of thickness $\unicode[STIX]{x1D6FE}^{-1/2}$ , within which the relative velocity is $O(\unicode[STIX]{x1D6FE}^{-1/2})$ , shear rates are $O(1)$ and the force $f$ is $O(\unicode[STIX]{x1D6FE}^{1/2})$ . In consequence, the energy equation (2.5) represents a large force $\tilde{f}$ confined to a thin boundary layer at the surface of the permeable solid, balanced by dissipation almost entirely within the unbounded fluid in $y>0$ , together with an oscillating kinetic energy term.

As $\unicode[STIX]{x1D6FE}\rightarrow 0$ ,

(2.17a,b ) $$\begin{eqnarray}F_{1}(\unicode[STIX]{x1D6FE})\sim \unicode[STIX]{x1D6FE},\quad F_{2}(\unicode[STIX]{x1D6FE})\sim \frac{\unicode[STIX]{x1D6FE}^{2}}{2^{3/2}}\left(-1+\frac{7\unicode[STIX]{x1D6FE}}{4}+\cdots \!\right)\end{eqnarray}$$

and the highly permeable porous solid exerts little force on the fluid, which in consequence hardly moves. We plot the functions $F_{1}(\unicode[STIX]{x1D6FE})$ and $F_{2}(\unicode[STIX]{x1D6FE})$ in figure 2. We see that $F_{1}$ , associated with work performed within the interior of the porous slab, has a maximum at $\unicode[STIX]{x1D6FE}=1$ , whereas $F_{2}$ , associated with the region near the boundary at $y=0$ , is not far from monotonic apart from a small overshoot near $\unicode[STIX]{x1D6FE}=10$ and a small undershoot near $\unicode[STIX]{x1D6FE}=0.4$ . If the porous slab has thickness $L\gg \unicode[STIX]{x1D6FE}^{-1/2}$ the total rate of working of the solid (2.15) is dominated by the term $LF_{1}(\unicode[STIX]{x1D6FE})$ and exhibits a maximum near $\unicode[STIX]{x1D6FE}=1$ . If $L\ll \unicode[STIX]{x1D6FE}^{-1/2}$ we expect the surface effects to play a dominant role. However, if the porous layer is shallow the expansion (2.12) for the mean rate of working when $L\gg \unicode[STIX]{x1D6FE}^{-1/2}$ is no longer valid, and we cannot ignore boundary conditions at $y=-L$ . One could investigate this limit by setting up an alternative geometry e.g. a symmetric problem with a porous slab occupying the region $-H<y<H$ , with fluid in the regions $|y|>H$ , but we shall not pursue this.

Figure 2. The functions $F_{1}(\unicode[STIX]{x1D6FE})$ and $F_{2}(\unicode[STIX]{x1D6FE})$ . Also shown are asymptotes for $F_{2}$ for (c) $\unicode[STIX]{x1D6FE}\ll 1$ (2.17), and (d) $\unicode[STIX]{x1D6FE}\gg 1$ (2.16).

3 Impulsive start-up from rest of a permeable wall

Stokes’ (1851) analysis of fluid motion adjacent to a plane wall that starts to move impulsively in its own plane with a steady velocity $\tilde{U} _{s}H(t)$ (where $H(t)$ is the Heaviside function) is a second textbook example taught to undergraduates (Batchelor 1973). We again investigate the effect of replacing the plane wall at ${\tilde{y}}=0$ by a permeable half-space ${\tilde{y}}<0$ .

The fluid is at rest at time $\tilde{t}=0$ , and subsequently moves with velocity $\tilde{u} (\tilde{t},{\tilde{y}})$ in the $\tilde{x}$ direction. Fluid motion in the region ${\tilde{y}}>0$ obeys the Navier–Stokes equation (2.1); in the region ${\tilde{y}}<0$ it obeys the Brinkman equation (2.4). As in § 2, pressure gradients are zero, so we set $\tilde{p}=0$ .

We scale velocities by $\tilde{U} _{s}$ , and for the moment we scale lengths in the $y$ direction by an arbitrary length scale $\tilde{L}$ . Time is scaled by $\tilde{L}^{2}\unicode[STIX]{x1D70C}/\unicode[STIX]{x1D707}$ , stress by $\unicode[STIX]{x1D707}\tilde{U} _{s}/\tilde{L}$ , force per unit volume by $\unicode[STIX]{x1D707}\tilde{U} _{s}/\tilde{L}^{2}$ and we denote non-dimensional quantities by the absence of a tilde. The governing equations become

(3.1a ) $$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x2202}u}{\unicode[STIX]{x2202}t}=\frac{\unicode[STIX]{x2202}^{2}u}{\unicode[STIX]{x2202}y^{2}},\quad y>0, & \displaystyle\end{eqnarray}$$

(3.1b ) $$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x2202}u}{\unicode[STIX]{x2202}t}=\frac{\unicode[STIX]{x2202}^{2}u}{\unicode[STIX]{x2202}y^{2}}-R(u-u_{s}),\quad y<0, & \displaystyle\end{eqnarray}$$

where $R=\tilde{L}^{2}/k$ and the non-dimensional solid velocity $u_{s}=H(t)$ . We take Laplace transforms, with

(3.2) $$\begin{eqnarray}u^{\ast }(s,y)=\int _{0}^{\infty }u(t,y)\text{e}^{-st}\,\text{d}t.\end{eqnarray}$$

The governing equations become

(3.3a ) $$\begin{eqnarray}\displaystyle & \displaystyle su^{\ast }=\frac{\unicode[STIX]{x2202}^{2}u^{\ast }}{\unicode[STIX]{x2202}y^{2}},\quad y>0, & \displaystyle\end{eqnarray}$$

(3.3b ) $$\begin{eqnarray}\displaystyle & \displaystyle su^{\ast }=\frac{\unicode[STIX]{x2202}^{2}u^{\ast }}{\unicode[STIX]{x2202}y^{2}}-R\left(u^{\ast }-\frac{1}{s}\right),\quad y<0, & \displaystyle\end{eqnarray}$$

with solutions

(3.4a ) $$\begin{eqnarray}\displaystyle & \displaystyle u^{\ast }=A(s)\exp \left(-s^{1/2}y\right),\quad y>0, & \displaystyle\end{eqnarray}$$

(3.4b ) $$\begin{eqnarray}\displaystyle & \displaystyle u^{\ast }=B(s)\exp \left((s+R)^{1/2}y\right)+\frac{R}{s(s+R)},\quad y<0. & \displaystyle\end{eqnarray}$$

Continuity of velocity $u^{\ast }$ and shear stress $\unicode[STIX]{x2202}u^{\ast }/\unicode[STIX]{x2202}y$ at $y=0$ gives

(3.5a,b ) $$\begin{eqnarray}A=\frac{R}{s(s+R)^{1/2}[(s+R)^{1/2}+s^{1/2}]},\quad B=-\frac{R}{s^{1/2}(s+R)[(s+R)^{1/2}+s^{1/2}]}.\end{eqnarray}$$

The fluid velocity in the permeable medium far from the interface ( $y\rightarrow -\infty$ ) depends solely on a balance between inertia and the Darcy resistance to flow, with

(3.6) $$\begin{eqnarray}u^{\ast }\rightarrow u_{\infty }^{\ast }=\frac{R}{s(s+R)}=\frac{1}{s}-\frac{1}{s+R}\quad \text{as }y\rightarrow -\infty ,\end{eqnarray}$$

corresponding to

(3.7) $$\begin{eqnarray}u\rightarrow u_{\infty }=1-\exp (-Rt).\end{eqnarray}$$

The non-dimensional force that the solid exerts on the fluid is $f=R(1-u)$ per unit volume. In an unbounded porous medium this force would be $R(1-u_{\infty })=R\exp (-Rt)$ . The total additional force (per unit length in the $x$ direction) due to the presence of the interface at $y=0$ is therefore

(3.8) $$\begin{eqnarray}F=R\int _{-\infty }^{0}[(1-u)-(1-u_{\infty })]\,\text{d}y,\end{eqnarray}$$

with Laplace transform

(3.9) $$\begin{eqnarray}F^{\ast }=-\frac{RB(s)}{(s+R)^{1/2}}=\frac{R^{2}}{s^{1/2}(s+R)^{3/2}[(s+R)^{1/2}+s^{1/2}]}.\end{eqnarray}$$

In the limit of an impermeable solid ( $k\rightarrow 0$ , $R=\tilde{L}^{2}/k\rightarrow \infty$ ), for fixed $s$ we find $F^{\ast }\sim s^{-1/2}$ corresponding to non-dimensional and dimensional forces

(3.10a,b ) $$\begin{eqnarray}F(t)\sim \frac{1}{(\unicode[STIX]{x03C0}t)^{1/2}},\quad \tilde{F}(t)\sim \frac{(\unicode[STIX]{x1D707}\unicode[STIX]{x1D70C})^{1/2}\tilde{U} _{s}}{(\unicode[STIX]{x03C0}\tilde{t})^{1/2}},\end{eqnarray}$$

in agreement with the classical result for impulsive start-up from rest of an impermeable solid surface.

For more general $R$ , the transform pairs (Abramowitz & Stegun 1972)

(3.11a,b ) $$\begin{eqnarray}f_{1}^{\ast }=\frac{1}{s+R},\quad f_{1}=\exp (-Rt)\end{eqnarray}$$

and

(3.12a,b ) $$\begin{eqnarray}f_{2}^{\ast }=\frac{1}{s^{1/2}(s+R)^{1/2}[(s+R)^{1/2}+s^{1/2}]},\quad f_{2}=\frac{1}{R^{1/2}}\exp (-Rt/2)\text{I}_{1/2}(Rt/2)\end{eqnarray}$$

(where $\text{I}_{1/2}(z)=\sinh (z)(2/\unicode[STIX]{x03C0}z)^{1/2}$ is a modified Bessel function) can be combined to find the inverse transform of $F^{\ast }$ (3.9), with

(3.13) $$\begin{eqnarray}\displaystyle F(t) & = & \displaystyle R^{2}\int _{0}^{t}f_{2}(u)f_{1}(t-u)\,\text{d}u\end{eqnarray}$$

(3.14) $$\begin{eqnarray}\displaystyle & = & \displaystyle R\left(\frac{t}{\unicode[STIX]{x03C0}}\right)^{1/2}\left[\int _{0}^{1}\frac{\exp (-Rtv)}{(1-v)^{1/2}}\,\text{d}v-2\exp (-Rt)\right].\end{eqnarray}$$

When $Rt\ll 1$ we expand the exponential in (3.14) to obtain

(3.15) $$\begin{eqnarray}F\sim \frac{2R^{2}t^{3/2}}{3\unicode[STIX]{x03C0}^{1/2}}-\frac{7R^{3}t^{5/2}}{15\unicode[STIX]{x03C0}^{1/2}}+\cdots \!,\end{eqnarray}$$

which could alternatively have been obtained by inverting term-by-term the expansion of $F^{\ast }$ (3.9) in the limit $s\rightarrow \infty$

(3.16) $$\begin{eqnarray}F^{\ast }\sim \frac{R^{2}}{2s^{5/2}}\left(1-\frac{7R}{4s}+\cdots \,\right)\!.\end{eqnarray}$$

The $t^{-1/2}$ singularity (3.10) when $k=0$ has been removed. When $Rt\gg 1$ we expand $(1-v)^{-1/2}$ in (3.14) as a power series for small $v$ , to obtain

(3.17) $$\begin{eqnarray}F\sim \frac{1}{(\unicode[STIX]{x03C0}t)^{1/2}}+\frac{1}{2R\unicode[STIX]{x03C0}^{1/2}t^{3/2}}+\cdots \!.\end{eqnarray}$$

Figure 3. (a) The additional force $F(t)$ (3.14), for $R=1$ . Also shown are asymptotes for (b) $t\gg 1$ (3.17), (c) $t\ll 1$ (3.15) (leading term only), (d) $t\ll 1$ (3.15) (two terms).

We now pick the length scale $L=k^{1/2}$ , so that $R=1$ . (The only reason for not adopting this natural choice straight away was so that we could consider the limit $k=0$ and thereby obtain (3.10).) Figure 3 shows the force $F$ (3.14), together with the asymptotes for $t\ll 1$ (3.15), and $t\gg 1$ (3.17).

The Laplace transform of the velocity on $y=0$ is $u^{\ast }(s,y=0)=A(s)$ , given by (3.5). To invert this, we note that the Laplace transform pair

(3.18a,b ) $$\begin{eqnarray}f_{3}^{\ast }=\frac{1}{s^{1/2}},\quad f_{3}=\frac{1}{(\unicode[STIX]{x03C0}t)^{1/2}}\end{eqnarray}$$

can be combined with the pair (3.12) to give

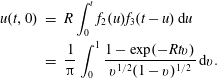(3.19) $$\begin{eqnarray}\displaystyle u(t,0) & = & \displaystyle R\int _{0}^{t}f_{2}(u)f_{3}(t-u)\,\text{d}u\nonumber\\ \displaystyle & = & \displaystyle \frac{1}{\unicode[STIX]{x03C0}}\int _{0}^{1}\frac{1-\exp (-Rtv)}{v^{1/2}(1-v)^{1/2}}\,\text{d}v.\end{eqnarray}$$

When $Rt\ll 1$ , we expand the exponential in (3.19) to obtain

(3.20) $$\begin{eqnarray}u(t,0)=\frac{Rt}{2}-\frac{3R^{2}t^{2}}{16}+\cdots \!,\end{eqnarray}$$

which can be obtained alternatively by inverting term-by-term the expansion of $A(s)$ for $s\gg R$ . We see that non-zero permeability smooths out the step change in fluid velocity that occurs adjacent to an impermeable surface at $t=0$ . When $Rt\gg 1$ ,

(3.21) $$\begin{eqnarray}u(t,0)\sim 1-\frac{1}{(\unicode[STIX]{x03C0}Rt)^{1/2}},\end{eqnarray}$$

and the expansion of (3.4b ) for $s\ll R$ leads to

(3.22) $$\begin{eqnarray}u(t,y)\sim 1-\exp (Rt)-\frac{\exp (R^{1/2}y)}{(\unicode[STIX]{x03C0}Rt)^{1/2}},\quad y<0,Rt\gg 1.\end{eqnarray}$$

4 Concluding remarks

The above Brinkman analysis includes an inertial $\unicode[STIX]{x1D70C}\,\unicode[STIX]{x2202}\boldsymbol{u}/\unicode[STIX]{x2202}t$ term, but studies of high Reynolds number flow inside model porous media have shown that nonlinear $\unicode[STIX]{x1D70C}\,\boldsymbol{u}\boldsymbol{\cdot }\unicode[STIX]{x1D735}\boldsymbol{u}$ inertial contributions due to tortuosity can be important within the porous medium, even when Darcy flow is nominally rectilinear (Mei & Auriault 1991; Koch & Hill 2001; Graham & Higdon 2002) and such nonlinear terms are absent from the Brinkman equation (2.4). A recent study by Lasseux, Valdés-Parada & Bellet (2019) of high Reynolds number unsteady flow in a porous medium (of porosity 0.4) compared direct numerical simulations and an upscaling model against a heuristic model based solely on the fluid inertia $\unicode[STIX]{x1D70C}\,\unicode[STIX]{x2202}\boldsymbol{u}/\unicode[STIX]{x2202}t$ and the steady Darcy permeability. The heuristic model, equivalent to Brinkman’s equation when flow is unidirectional, overestimated the effect of oscillatory pressure gradient fluctuations by a factor ${\approx}2$ . Although Brinkman’s equation should capture the essential physics of the problems discussed in §§ 2 and 3, the quantitative accuracy of its predictions will depend upon the details of the geometry of the porous medium and the flow regime within it.