Published online by Cambridge University Press: 14 August 2015
A new methodology, based on the central limit theorem, is applied to describe the statistical mechanics of two-dimensional point vortex motion in a bounded container 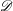 $\mathscr{D}$, as the number of vortices
$\mathscr{D}$, as the number of vortices 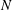 $N$ tends to infinity. The key to the approach is the identification of the normal modes of the system with the eigenfunction solutions of the so-called hydrodynamic eigenvalue problem of the Laplacian in
$N$ tends to infinity. The key to the approach is the identification of the normal modes of the system with the eigenfunction solutions of the so-called hydrodynamic eigenvalue problem of the Laplacian in 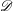 $\mathscr{D}$. The statistics of the projection of the vorticity distribution onto these eigenfunctions (‘vorticity projections’) are then investigated. The statistics are used first to obtain the density-of-states function and caloric curve for the system, generalising previous results to arbitrary (neutral) distributions of vortex circulations. Explicit expressions are then obtained for the microcanonical (i.e. fixed energy) probability density functions of the vorticity projections in a form that can be compared directly with direct numerical simulations of the dynamics. The energy spectra of the resulting flows are predicted analytically. Ensembles of simulations with
$\mathscr{D}$. The statistics of the projection of the vorticity distribution onto these eigenfunctions (‘vorticity projections’) are then investigated. The statistics are used first to obtain the density-of-states function and caloric curve for the system, generalising previous results to arbitrary (neutral) distributions of vortex circulations. Explicit expressions are then obtained for the microcanonical (i.e. fixed energy) probability density functions of the vorticity projections in a form that can be compared directly with direct numerical simulations of the dynamics. The energy spectra of the resulting flows are predicted analytically. Ensembles of simulations with 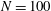 $N=100$, in several conformal domains, are used to make a comprehensive validation of the theory, with good agreement found across a broad range of energies. The probability density function of the leading vorticity projection is of particular interest because it has a unimodal distribution at low energy and a bimodal distribution at high energy. This behaviour is indicative of a phase transition, known as Onsager–Kraichnan condensation in the literature, between low-energy states with no mean flow in the domain and high-energy states with a coherent mean flow. The critical temperature for the phase transition, which depends on the shape but not the size of
$N=100$, in several conformal domains, are used to make a comprehensive validation of the theory, with good agreement found across a broad range of energies. The probability density function of the leading vorticity projection is of particular interest because it has a unimodal distribution at low energy and a bimodal distribution at high energy. This behaviour is indicative of a phase transition, known as Onsager–Kraichnan condensation in the literature, between low-energy states with no mean flow in the domain and high-energy states with a coherent mean flow. The critical temperature for the phase transition, which depends on the shape but not the size of 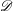 $\mathscr{D}$, and the associated critical energy are found. Finally the accuracy and the extent of the validity of the theory, at finite
$\mathscr{D}$, and the associated critical energy are found. Finally the accuracy and the extent of the validity of the theory, at finite 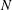 $N$, are explored using a Markov chain phase-space sampling method.
$N$, are explored using a Markov chain phase-space sampling method.