Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-24T21:20:39.893Z Has data issue: false hasContentIssue false
  • English
  • Français

Two-dimensional continuum modelling granular column collapse by non-local peridynamics in a mesh-free method with $\mu(I)$ rheology

Published online by Cambridge University Press:  30 April 2021

Tibing Xu Open the ORCID record for Tibing Xu [Opens in a new window]  and
Yee-Chung Jin Open the ORCID record for Yee-Chung Jin [Opens in a new window]
Tibing Xu*
Affiliation:
School of Civil and Environmental Engineering, Ningbo University, 315211Ningbo, PR China
Yee-Chung Jin
Affiliation:
Faculty of Applied Science and Engineering, University of Regina, ReginaS4S 0A2, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

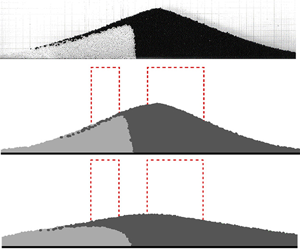
Granular column collapse involves complicated granular flow bridging initial and final solid-like states, with intermediate multi-regime spatial and temporal flow patterns and transitions, which include large free-surface variation. In this study, a non-local mesh-free numerical method is proposed to model these flows and capture the entire process from flowing to arresting. Free surface evolution is tracked by the mesh-free method while the non-local theory of peridynamics is used to capture the arresting for the flow. The constitutive relation to calculate the effective viscosity and stress is based on the μ(I) rheology. The non-local mesh-free method is validated to simulate a granular column collapse in which effects from the peridynamic horizon and particle distance are both examined. Non-local modelling is then used to simulate more types of granular column collapses, by comparison with other numerical results and experimental observations in terms of the free surface and velocity variations in both the fluid-like and solid-like states. The collision between two adjacent collapsing granular columns is also simulated, and the interface variations between material from each collapsing column are compared with experimental observations. The non-local modelling is shown to reflect the internal flow characteristics in the granular flow. Throughout these simulations, the non-local mesh-free method is able to calculate the free surface, velocity and interface variation in the granular flows.

JFM classification

Granular media: Granular media Mathematical Foundations: Computational methods Multiphase and Particle-laden Flows: Particle/fluid flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 917 , 25 June 2021 , A51
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Agwai, A., Guven, I. & Madenci, E. 2011 Predicting crack propagation with Peridynamics: a comparative study. Intl J. Fract. 171, 65–78.CrossRefGoogle Scholar
Balmforth, N.J. & Kerswell, R.R. 2005 Granular collapse in two dimensions. J. Fluid Mech. 538, 399–428.CrossRefGoogle Scholar
Bessa, M.A., Foster, J.T., Belytschko, T. & Liu, W.K. 2014 A meshfree unification: reproducing kernel Peridynamics. Comput. Mech. 53 (6), 1251–1264.CrossRefGoogle Scholar
Bouzid, M., Izzet, A., Trulsson, M., Clément, E., Claudin, P. & Andreotti, B. 2015 Non-local rheology in dense granular flows. Eur. Phys. J. E 38, 125.CrossRefGoogle ScholarPubMed
Bouzid, M., Trulsson, M., Claudin, P., Clément, E. & Andreotti, B. 2013 Nonlocal rheology of granular flows across yield conditions. Phys. Rev. Lett. 111 (23), 238301.CrossRefGoogle ScholarPubMed
Chauchat, J. & Médale, M. 2014 A three-dimensional numerical model for dense granular flows based on the μ(I) rheology. J. Comput. Phys. 256, 696–712.CrossRefGoogle Scholar
Crosta, G.B., Imposimato, S. & Roddeman, D. 2009 Numerical modeling of 2-D granular step collapse on erodible and nonerodible surface. J. Geophys. Res.-Earth 114, F03020.Google Scholar
Da Cruz, F., Emam, S., Prochnow, M., Roux, J.N. & Chevoir, F. 2005 Rheophysics of dense granular materials: discrete simulation of plane shear flows. Phys. Rev. E 72 (2), 021309.CrossRefGoogle ScholarPubMed
Dunatunga, S. & Kamrin, K. 2015 Continuum modelling and simulation of granular flows through their many phases. J. Fluid Mech. 779, 483–513.CrossRefGoogle Scholar
Franci, A. & Cremonesi, M. 2019 3D regularized μ(I)-rheology for granular flows simulation. J. Comput. Phys. 378, 257–277.CrossRefGoogle Scholar
Girolami, L., Hergault, V., Vinay, G. & Wachs, A. 2012 A three-dimensional discrete-grain model for the simulation of dam-break rectangular collapses: comparison between numerical results and experiments. Granul. Matt. 14 (3), 381–392.CrossRefGoogle Scholar
González-Cao, J., Altomare, C., Crespo, A.J.C., Domínguez, J.M., Gómez-Gesteira, M. & Kisacik, D. 2019 On the accuracy of DualSPHysics to assess violent collisions with coastal structures. Comput. Fluids 179, 604–612.CrossRefGoogle Scholar
Gotoh, H. & Khayyer, A. 2016 Current achievements and future perspectives for projection-based particle methods with applications in ocean engineering. J. Ocean Engng Mar. Energy 2 (3), 251–278.CrossRefGoogle Scholar
Gu, X.B. & Wu, Q.H. 2016 The application of nonordinary, state-based Peridynamic theory on the damage process of the rock-like materials. Math. Probl. Engng 2016, 9794605.Google Scholar
Henann, D.L. & Kamrin, K. 2013 A predictive, size-dependent continuum model for dense granular flows. Proc. Natl Acad. Sci. 110 (17), 6730–6735.CrossRefGoogle ScholarPubMed
Ionescu, I.R., Mangeney, A., Bouchut, F. & Roche, O. 2015 Viscoplastic modeling of granular column collapse with pressure-dependent rheology. J. Non-Newtonian Fluid Mech. 219, 1–18.CrossRefGoogle Scholar
Jop, P. 2015 Rheological properties of dense granular flows. C. R. Phys. 16 (1), 62–72.CrossRefGoogle Scholar
Jop, P., Forterre, Y. & Pouliquen, O. 2005 Crucial role of sidewalls in granular surface flows: consequences for the rheology. J. Fluid Mech. 541, 167-192.CrossRefGoogle Scholar
Jop, P., Forterre, Y. & Pouliquen, O. 2006 A constitutive law for dense granular flows. Nature 441, 727–730.CrossRefGoogle ScholarPubMed
Khayyer, A., Gotoh, H. & Shimizu, Y. 2017 Comparative study on accuracy and conservation properties of two particle regularization schemes and proposal of an optimized particle shifting scheme in ISPH context. J. Comput. Phys. 332, 236–256.CrossRefGoogle Scholar
Koshizuka, S., Nobe, A. & Oka, Y. 1998 Numerical analysis of breaking waves using the moving particle semi-implicit method. Intl J. Numer. Meth. Fluids 26 (7), 751–769.3.0.CO;2-C>CrossRefGoogle Scholar
Lacaze, L. & Kerswell, R.R. 2009 Axisymmetric granular collapse: a transient 3D flow test of viscoplasticity. Phys. Rev. Lett. 102 (10), 108305.CrossRefGoogle ScholarPubMed
Lagrée, P.Y., Staron, L. & Popinet, S. 2011 The granular column collapse as a continuum: validity of a two-dimensional Navier–Stokes model with a μ(I)-rheology. J. Fluid Mech. 686, 378–408.CrossRefGoogle Scholar
Lajeunesse, E., Mangeney-Castelnau, A. & Vilotte, J.P. 2004 Spreading of a granular mass on a horizontal plane. Phys. Fluids 16 (7), 2371–2381.CrossRefGoogle Scholar
Lajeunesse, E., Monnier, J.B. & Homsy, G.M. 2005 Granular slumping on a horizontal surface. Phys. Fluids 17 (10), 103302.CrossRefGoogle Scholar
Lee, B.H., Park, J.C., Kim, M.H. & Hwang, S.C. 2011 Step-by-step improvement of MPS method in simulating violent free-surface motions and impact-loads. Comput. Method. Appl. Mech. Engng 200 (9–12), 1113–1125.CrossRefGoogle Scholar
Lube, G., Huppert, H.E., Sparks, R.S.J. & Freundt, A. 2005 Collapses of two-dimensional granular columns. Phys. Rev. E 72 (4), 041301.CrossRefGoogle ScholarPubMed
Lube, G., Huppert, H.E., Sparks, R.S.J. & Freundt, A. 2007 Static and flowing regions in granular collapses down channels. Phys. Fluids 19 (4), 043301.CrossRefGoogle Scholar
Lube, G., Huppert, H.E., Sparks, R.S.J. & Hallworth, M.A. 2004 Axisymmetric collapses of granular columns. J. Fluid Mech. 508, 175–199.CrossRefGoogle Scholar
Mangeney, A., Roche, O., Hungr, O., Mangold, N., Faccanoni, G. & Lucas, A. 2010 Erosion and mobility in granular collapse over sloping beds. J. Geophys. Res.-Earth 115, F03040.Google Scholar
Mast, C.M., Arduino, P., Mackenzie-Helnwein, P. & Miller, G.R. 2015 Simulating granular column collapse using the material point method. Acta Geotech. 10 (1), 101–116.CrossRefGoogle Scholar
Mengesha, T. & Du, Q. 2014 Nonlocal constrained value problems for a linear Peridynamic Navier equation. J. Elasticity 116 (1), 27–51.CrossRefGoogle Scholar
MiDi, G.D.R. 2004 On dense granular flows. Euro. Phys. J. E 14 (4), 341–365.CrossRefGoogle Scholar
Minatti, L. & Paris, E. 2015 A SPH model for the simulation of free surface granular flows in a dense regime. Appl. Math. Model. 39 (1), 363–382.CrossRefGoogle Scholar
Monaghan, J.J. 1994 Simulating free surface flows with SPH. J. Comput. Phys. 110 (2), 399–406.CrossRefGoogle Scholar
Oger, G., Marrone, S., Le Touzé, D. & De Leffe, M. 2016 SPH accuracy improvement through the combination of a quasi-Lagrangian shifting transport velocity and consistent ALE formalisms. J. Comput. Phys. 313, 76–98.CrossRefGoogle Scholar
Peng, C., Guo, X., Wu, W. & Wang, Y. 2016 Unified modelling of granular media with smoothed particle hydrodynamics. Acta Geotech. 11 (6), 1231–1247.CrossRefGoogle Scholar
Pouliquen, O. & Forterre, Y. 2009 A non-local rheology for dense granular flows. Phil. Trans. R. Soc. A 367 (1909), 5091–5107.CrossRefGoogle ScholarPubMed
Ren, B., Fan, H., Bergel, G.L., Regueiro, R.A., Lai, X. & Li, S. 2015 A Peridynamics–SPH coupling approach to simulate soil fragmentation induced by shock waves. Comput. Mech. 55 (2), 287–302.CrossRefGoogle Scholar
Rufai, O., Jin, Y.C. & Tai, Y.C. 2019 Rheometry of dense granular collapse on inclined planes. Granul. Matt. 21 (3), 62.CrossRefGoogle Scholar
Shadloo, M.S., Zainali, A., Yildiz, M. & Suleman, A. 2012 A robust weakly compressible SPH method and its comparison with an incompressible SPH. Intl J. Numer. Meth. Engng 89 (8), 939–956.CrossRefGoogle Scholar
Shakibaeinia, A. & Jin, Y.C. 2010 A weakly compressible MPS method for modeling of open-boundary free-surface flow. Intl J. Numer. Meth. Fluids 63 (10), 1208–1232.Google Scholar
Shakibaeinia, A. & Jin, Y.C. 2012 MPS mesh-free particle method for multiphase flows. Comput. Meth. Appl. Mech. Engng 229, 13–26.CrossRefGoogle Scholar
Siavoshi, S. & Kudrolli, A. 2005 Failure of a granular step. Phys. Rev. E 71 (5), 051302.CrossRefGoogle ScholarPubMed
Siavoshi, S., Orpe, A.V. & Kudrolli, A. 2006 Friction of a slider on a granular layer: nonmonotonic thickness dependence and effect of boundary conditions. Phys. Rev. E 73 (1), 010301.CrossRefGoogle ScholarPubMed
Silling, S.A. & Askari, E. 2005 A meshfree method based on the Peridynamic model of solid mechanics. Comput. Struct. 83 (17–18), 1526–1535.CrossRefGoogle Scholar
Silling, S.A., Epton, M., Weckner, O., Xu, J. & Askari, E. 2007 Peridynamic states and constitutive modeling. J. Elasticity 88 (2), 151–184.CrossRefGoogle Scholar
Silling, S.A. & Lehoucq, R.B. 2008 Convergence of Peridynamics to classical elasticity theory. J. Elasticity 93, 13–37.CrossRefGoogle Scholar
Staron, L. & Hinch, E.J. 2005 Study of the collapse of granular columns using two-dimensional discrete-grain simulation. J. Fluid Mech. 545, 1–27.CrossRefGoogle Scholar
Staron, L., Lagrée, P.Y., Josserand, C. & Lhuillier, D. 2010 Flow and jamming of a two-dimensional granular bed: toward a nonlocal rheology? Phys. Fluids 22 (11), 113303.CrossRefGoogle Scholar
Taylor, M., Gözen, I., Patel, S., Jesorka, A. & Bertoldi, K. 2016 Peridynamic modeling of ruptures in biomembranes. PloS one 11 (11), e0165947.CrossRefGoogle ScholarPubMed
Violeau, D. & Rogers, B.D. 2016 Smoothed particle hydrodynamics (SPH) for free-surface flows: past, present and future. J. Hydraul. Res. 54 (1), 1–26.CrossRefGoogle Scholar
Wang, J. & Zhang, X. 2018 Modified particle method with integral Navier–Stokes formulation for incompressible flows. J. Comput. Phys. 366, 1–13.CrossRefGoogle Scholar
Xu, T. & Jin, Y.C. 2016 a Improvements for accuracy and stability in a weakly-compressible particle method. Comput. Fluids 137, 1–14.CrossRefGoogle Scholar
Xu, T. & Jin, Y.C. 2016 b Modeling free-surface flows of granular column collapses using a mesh-free method. Powder Technol. 291, 20–34.CrossRefGoogle Scholar
Xu, T., Jin, Y.C. & Tai, Y.C. 2019 Granular surface waves interaction across phases modeled by mesh-free method. Powder Technol. 355, 226–241.CrossRefGoogle Scholar
Xu, T., Jin, Y.C., Tai, Y.C. & Lu, C.H. 2017 Simulation of velocity and shear stress distributions in granular column collapses by a mesh-free method. J. Non-Newtonian Fluid Mech. 247, 146–164.CrossRefGoogle Scholar
Ye, T., Pan, D., Huang, C. & Liu, M. 2019 Smoothed particle hydrodynamics (SPH) for complex fluid flows: recent developments in methodology and applications. Phys. Fluids 31 (1), 011301.Google Scholar