Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-12-03T20:28:33.013Z Has data issue: false hasContentIssue false
  • English
  • Français

Two-degree-of-freedom flow-induced vibrations of a rotating cylinder

Published online by Cambridge University Press:  18 June 2020

Rémi Bourguet Open the ORCID record for Rémi Bourguet [Opens in a new window]
Rémi Bourguet*
Affiliation:
Institut de Mécanique des Fluides de Toulouse, Université de Toulouse and CNRS, Toulouse, 31400, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

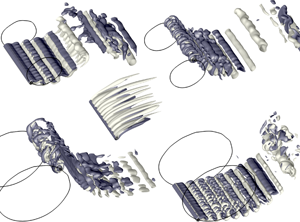
The flow-induced vibrations of an elastically mounted circular cylinder, free to oscillate in the streamwise and cross-flow directions, and forced to rotate about its axis, are investigated via two- and three-dimensional simulations. The Reynolds number based on the body diameter and inflow velocity is equal to $100$. The impact of the imposed rotation on the flow–structure system behaviour is explored over wide ranges of values of the rotation rate (ratio between the cylinder surface and inflow velocities, $\unicode[STIX]{x1D6FC}\in [0,5.5]$) and of the reduced velocity (inverse of the oscillator natural frequency non-dimensionalized by the inflow velocity and body diameter, $U^{\star }\in [1,25]$). Flow-induced vibrations are found to develop over the entire range of $\unicode[STIX]{x1D6FC}$, including in the intervals where the imposed rotation cancels flow unsteadiness when the body is rigidly mounted (i.e. not allowed to translate). The responses of the two-degree-of-freedom oscillator substantially depart from their one-degree-of-freedom counterparts. Up to a rotation rate close to $2$, the body exhibits oscillations comparable to the vortex-induced vibrations usually reported for a non-rotating circular cylinder: they develop under flow–body synchronization and their amplitudes present bell-shaped evolutions as functions of $U^{\star }$. They are, however, enhanced by the rotation as they can reach $1$ body diameter in each direction, which represents twice the peak amplitude of cross-flow response for $\unicode[STIX]{x1D6FC}=0$. The symmetry breaking due to the rotation results in deviations from the typical figure-eight orbits. The flow remains close to that observed in the rigidly mounted body case, i.e. two-dimensional with two spanwise vortices shed per cycle. Beyond $\unicode[STIX]{x1D6FC}=2$, the structural responses resemble the galloping oscillations generally encountered for non-axisymmetric bodies, with amplitudes growing unboundedly with $U^{\star }$. The response growth rate increases with $\unicode[STIX]{x1D6FC}$ and amplitudes larger than $20$ diameters are observed. The cylinder describes, at low frequencies, elliptical orbits oriented in the opposite sense compared to the imposed rotation. The emergence of subharmonic components of body displacements, leading to period doubling or quadrupling, induces slight variations about this canonical shape. These responses are not predicted by a quasi-steady modelling of fluid forcing, i.e. based on the evolution of the mean flow at each step of body motion; this suggests that the interaction with flow unsteadiness cannot be neglected. It is shown that flow–body synchronization persists, which is not expected for galloping oscillations. Within this region of the parameter space, the flow undergoes a major reconfiguration. A myriad of novel spatio-temporal structures arise with up to $20$ vortices formed per cycle. The flow three-dimensional transition occurs down to $\unicode[STIX]{x1D6FC}\approx 2$, versus $3.7$ for the rigidly mounted body. It is, however, shown that it has only a limited influence on the system behaviour.

JFM classification

Wakes/Jets: Vortex streets Wakes/Jets: Wakes
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 897 , 25 August 2020 , A31
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdel-Rohman, M. 1992 Galloping of tall prismatic structures: a two-dimensional analysis. J. Sound Vib. 153, 97–111.Google Scholar
Aljure, D. E., Rodríguez, I., Lehmkuhl, O., Pérez-Segarra, C. D. & Oliva, A. 2015 Influence of rotation on the flow over a cylinder at Re = 5000. Intl J. Heat Fluid Flow 55, 76–90.CrossRefGoogle Scholar
Badr, H. M., Coutanceau, M., Dennis, S. C. R. & Ménard, C. 1990 Unsteady flow past a rotating circular cylinder at Reynolds numbers 103 and 104. J. Fluid Mech. 220, 459–484.CrossRefGoogle Scholar
Bearman, P. W. 1984 Vortex shedding from oscillating bluff bodies. Annu. Rev. Fluid Mech. 16, 195–222.CrossRefGoogle Scholar
Bearman, P. W. 2011 Circular cylinder wakes and vortex-induced vibrations. J. Fluids Struct. 27, 648–658.CrossRefGoogle Scholar
Bearman, P. W., Gartshore, I. S., Maull, D. J. & Parkinson, G. V. 1987 Experiments on flow-induced vibration of a square-section cylinder. J. Fluids Struct. 1, 19–34.CrossRefGoogle Scholar
Blackburn, H. M., Govardhan, R. & Williamson, C. H. K. 2000 A complementary numerical and physical investigation of vortex-induced vibration. J. Fluids Struct. 15, 481–488.CrossRefGoogle Scholar
Blevins, R. D. 1990 Flow-Induced Vibration. Van Nostrand Reinhold.Google Scholar
Bourguet, R. 2019 Flow-induced vibrations of a rotating cylinder in an arbitrary direction. J. Fluid Mech. 860, 739–766.CrossRefGoogle Scholar
Bourguet, R., Karniadakis, G. E. & Triantafyllou, M. S. 2013 Distributed lock-in drives broadband vortex-induced vibrations of a long flexible cylinder in shear flow. J. Fluid Mech. 717, 361–375.CrossRefGoogle Scholar
Bourguet, R. & Lo Jacono, D. 2014 Flow-induced vibrations of a rotating cylinder. J. Fluid Mech. 740, 342–380.CrossRefGoogle Scholar
Bourguet, R. & Lo Jacono, D. 2015 In-line flow-induced vibrations of a rotating cylinder. J. Fluid Mech. 781, 127–165.CrossRefGoogle Scholar
Bourguet, R. & Triantafyllou, M. S. 2016 The onset of vortex-induced vibrations of a flexible cylinder at large inclination angle. J. Fluid Mech. 809, 111–134.CrossRefGoogle Scholar
Cagney, N. & Balabani, S. 2013 Wake modes of a cylinder undergoing free streamwise vortex-induced vibrations. J. Fluids Struct. 38, 127–145.CrossRefGoogle Scholar
Cagney, N. & Balabani, S. 2014 Streamwise vortex-induced vibrations of cylinders with one and two degrees of freedom. J. Fluid Mech. 758, 702–727.CrossRefGoogle Scholar
Chew, Y. T., Cheng, M. & Luo, S. C. 1995 A numerical study of flow past a rotating circular cylinder using a hybrid vortex scheme. J. Fluid Mech. 299, 35–71.CrossRefGoogle Scholar
Corless, R. M. & Parkinson, G. V. 1988 A model of the combined effects of vortex-induced oscillation and galloping. J. Fluids Struct. 2, 203–220.CrossRefGoogle Scholar
Dahl, J. M., Hover, F. S., Triantafyllou, M. S. & Oakley, O. H. 2010 Dual resonance in vortex-induced vibrations at subcritical and supercritical Reynolds numbers. J. Fluid Mech. 643, 395–424.CrossRefGoogle Scholar
Den Hartog, J. P. 1932 Transmission line vibration due to sleet. Trans. Am. Inst. Electr. Engrs 51, 1074–1076.CrossRefGoogle Scholar
Díaz, F., Gavaldà, J., Kawall, J. G., Keffer, J. F. & Giralt, F. 1983 Vortex shedding from a spinning cylinder. Phys. Fluids 26, 3454.CrossRefGoogle Scholar
Feng, C. C.1968 The measurement of vortex-induced effects in flow past stationary and oscillating circular and D-section cylinders. Master’s thesis, University of British Columbia.Google Scholar
Gsell, S., Bourguet, R. & Braza, M. 2016 Two-degree-of-freedom vortex-induced vibrations of a circular cylinder at Re = 3900. J. Fluids Struct. 67, 156–172.CrossRefGoogle Scholar
Gsell, S., Bourguet, R. & Braza, M. 2019 One- versus two-degree-of-freedom vortex-induced vibrations of a circular cylinder at Re = 3900. J. Fluids Struct. 85, 165–180.CrossRefGoogle Scholar
Gurian, T. D., Currier, T. & Modarres-Sadeghi, Y. 2019 Flow force measurements and the wake transition in purely inline vortex-induced vibration of a circular cylinder. Phys. Rev. F 4, 034701.Google Scholar
Hémon, P., Amandolese, X. & Andrianne, T. 2017 Energy harvesting from galloping of prisms: A wind tunnel experiment. J. Fluids Struct. 70, 390–402.CrossRefGoogle Scholar
Hémon, P. & Santi, F. 2002 On the aeroelastic behaviour of rectangular cylinders in cross-flow. J. Fluids Struct. 16, 855–889.CrossRefGoogle Scholar
Hover, F. S., Techet, A. H. & Triantafyllou, M. S. 1998 Forces on oscillating uniform and tapered cylinders in crossflow. J. Fluid Mech. 363, 97–114.CrossRefGoogle Scholar
Jauvtis, N. & Williamson, C. H. K. 2004 The effect of two degrees of freedom on vortex-induced vibration at low mass and damping. J. Fluid Mech. 509, 23–62.CrossRefGoogle Scholar
Jones, K. F. 1992 Coupled vertical and horizontal galloping. J. Engng Mech. 118, 92–107.Google Scholar
Kang, S., Choi, H. & Lee, S. 1999 Laminar flow past a rotating circular cylinder. Phys. Fluids 11, 3312.CrossRefGoogle Scholar
Karniadakis, G. E. & Sherwin, S. 1999 Spectral/hp Element Methods for CFD (first edition). Oxford University Press.Google Scholar
Khalak, A. & Williamson, C. H. K. 1999 Motions, forces and mode transitions in vortex-induced vibrations at low mass-damping. J. Fluids Struct. 13, 813–851.CrossRefGoogle Scholar
Klamo, J. T., Leonard, A. & Roshko, A. 2006 The effects of damping on the amplitude and frequency response of a freely vibrating cylinder in cross-flow. J. Fluids Struct. 22, 845–856.CrossRefGoogle Scholar
Konstantinidis, E. 2014 On the response and wake modes of a cylinder undergoing streamwise vortex-induced vibration. J. Fluids Struct. 45, 256–262.CrossRefGoogle Scholar
Leontini, J. S., Stewart, B. E., Thompson, M. C. & Hourigan, K. 2006 Wake state and energy transitions of an oscillating cylinder at low Reynolds number. Phys. Fluids 18, 067101.CrossRefGoogle Scholar
Lo Jacono, D., Bourguet, R., Thompson, M. C. & Leontini, J. S. 2018 Three-dimensional mode selection of the flow past a rotating and inline oscillating cylinder. J. Fluid Mech. 855, R3.CrossRefGoogle Scholar
Lucor, D., Foo, J. & Karniadakis, G. E. 2005 Vortex mode selection of a rigid cylinder subject to VIV at low mass-damping. J. Fluids Struct. 20, 483–503.CrossRefGoogle Scholar
Mannini, C., Marra, A. M. & Bartoli, G. 2016 Interference of vortex-induced vibration and transverse galloping for a rectangular cylinder. J. Fluids Struct. 66, 403–423.CrossRefGoogle Scholar
Mittal, S. & Kumar, B. 2003 Flow past a rotating cylinder. J. Fluid Mech. 476, 303–334.CrossRefGoogle Scholar
Mittal, S. & Tezduyar, T. E. 1992 A finite element study of incompressible flows past oscillating cylinders and aerofoils. Intl J. Numer. Meth. Fluids 15, 1073–1118.CrossRefGoogle Scholar
Mukhopadhyay, V. & Dugundji, J. 1976 Wind excited vibration of a square section cantilever beam in smooth flow. J. Sound Vib. 45, 329–339.Google Scholar
Nakamura, Y. & Tomonari, Y. 1977 Galloping of rectangular prisms in a smooth and a turbulent flow. J. Sound Vib. 52, 233–241.Google Scholar
Naudascher, E. 1987 Flow-induced streamwise vibrations of structures. J. Fluids Struct. 1, 265–298.CrossRefGoogle Scholar
Naudascher, E. & Rockwell, D. 1994 Flow-induced Vibrations: an Engineering Guide. Dover Publications.Google Scholar
Navrose, Meena, J. & Mittal, S. 2015 Three-dimensional flow past a rotating cylinder. J. Fluid Mech. 766, 28–53.CrossRefGoogle Scholar
Navrose & Mittal, S. 2016 Lock-in in vortex-induced vibration. J. Fluid Mech. 794, 565–594.CrossRefGoogle Scholar
Nemes, A., Zhao, J., Lo Jacono, D. & Sheridan, J. 2012 The interaction between flow-induced vibration mechanisms of a square cylinder with varying angles of attack. J. Fluid Mech. 710, 102–130.CrossRefGoogle Scholar
Okajima, A., Kosugi, T. & Nakamura, A. 2002 Flow-induced in-line oscillation of a circular cylinder in a water tunnel. J. Pressure Vessel Technol. 124, 89–96.CrossRefGoogle Scholar
Païdoussis, M. P., Price, S. J. & de Langre, E. 2010 Fluid-Structure Interactions: Cross-Flow-Induced Instabilities. Cambridge University Press.CrossRefGoogle Scholar
Parkinson, G. V. & Smith, J. D. 1964 The square prism as an aeroelastic nonlinear oscillator. Q. J. Mech. Appl. Maths 17, 225–239.CrossRefGoogle Scholar
Pralits, J. O., Brandt, L. & Giannetti, F. 2010 Instability and sensitivity of the flow around a rotating circular cylinder. J. Fluid Mech. 650, 513–536.CrossRefGoogle Scholar
Pralits, J. O., Giannetti, F. & Brandt, L. 2013 Three-dimensional instability of the flow around a rotating circular cylinder. J. Fluid Mech. 730, 5–18.CrossRefGoogle Scholar
Radi, A., Thompson, M. C., Rao, A., Hourigan, K. & Sheridan, J. 2013 Experimental evidence of new three-dimensional modes in the wake of a rotating cylinder. J. Fluid Mech. 734, 567–594.CrossRefGoogle Scholar
Rao, A., Leontini, J. S., Thompson, M. C. & Hourigan, K. 2013 Three-dimensionality in the wake of a rapidly rotating cylinder in uniform flow. J. Fluid Mech. 730, 379–391.CrossRefGoogle Scholar
Riches, G. & Morton, C. 2018 One degree-of-freedom vortex-induced vibrations at constant Reynolds number and mass-damping. Exp. Fluids 59, 157.CrossRefGoogle Scholar
Sarpkaya, T. 2004 A critical review of the intrinsic nature of vortex-induced vibrations. J. Fluids Struct. 19, 389–447.CrossRefGoogle Scholar
Seyed-Aghazadeh, B., Carlson, D. W. & Modarres-Sadeghi, Y. 2017 Vortex-induced vibration and galloping of prisms with triangular cross-sections. J. Fluid Mech. 817, 590–618.CrossRefGoogle Scholar
Seyed-Aghazadeh, B. & Modarres-Sadeghi, Y. 2015 An experimental investigation of vortex-induced vibration of a rotating circular cylinder in the crossflow direction. Phys. Fluids 27, 067101.CrossRefGoogle Scholar
Shiels, D., Leonard, A. & Roshko, A. 2001 Flow-induced vibration of a circular cylinder at limiting structural parameters. J. Fluids Struct. 15, 3–21.CrossRefGoogle Scholar
Stansby, P. K. & Rainey, R. C. T. 2001 On the orbital response of a rotating cylinder in a current. J. Fluid Mech. 439, 87–108.CrossRefGoogle Scholar
Stojković, D., Breuer, M. & Durst, F. 2002 Effect of high rotation rates on the laminar flow around a circular cylinder. Phys. Fluids 14, 3160.CrossRefGoogle Scholar
Tamura, T. 1999 Reliability on CFD estimation for wind-structure interaction problems. J. Wind Engng Ind. Aerodyn. 81, 117–143.CrossRefGoogle Scholar
Williamson, C. H. K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–538.CrossRefGoogle Scholar
Williamson, C. H. K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.CrossRefGoogle Scholar
Williamson, C. H. K. & Roshko, A. 1988 Vortex formation in the wake of an oscillating cylinder. J. Fluids Struct. 2, 355–381.CrossRefGoogle Scholar
Wong, K. W. L., Zhao, J., Lo Jacono, D., Thompson, M. C. & Sheridan, J. 2017 Experimental investigation of flow-induced vibration of a rotating circular cylinder. J. Fluid Mech. 829, 486–511.CrossRefGoogle Scholar
Yao, W. & Jaiman, R. K. 2017 Model reduction and mechanism for the vortex-induced vibrations of bluff bodies. J. Fluid Mech. 827, 357–393.CrossRefGoogle Scholar
Yogeswaran, V. & Mittal, S. 2011 Vortex-induced and galloping response of a rotating circular cylinder. In IUTAM Symposium on Bluff Body Flows, IIT-Kanpur, India (ed. Mittal, S. & Biswas, G.), pp. 153–156.Google Scholar
Zhao, J., Hourigan, K. & Thompson, M. C. 2019 An experimental investigation of flow-induced vibration of high-side-ratio rectangular cylinders. J. Fluids Struct. 91, 91025.CrossRefGoogle Scholar
Zhao, J., Leontini, J. S., Lo Jacono, D. & Sheridan, J. 2014a Fluid-structure interaction of a square cylinder at different angles of attack. J. Fluid Mech. 747, 688–721.CrossRefGoogle Scholar
Zhao, J., Lo Jacono, D., Sheridan, J., Hourigan, K. & Thompson, M. C. 2018 Experimental investigation of in-line flow-induced vibration of a rotating circular cylinder. J. Fluid Mech. 847, 664–699.CrossRefGoogle Scholar
Zhao, M., Cheng, L. & Lu, L. 2014b Vortex induced vibrations of a rotating circular cylinder at low Reynolds number. Phys. Fluids 26, 073602.Google Scholar