Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-12-01T04:36:54.176Z Has data issue: false hasContentIssue false
  • English
  • Français

The two classes of primary modal instability in laminar separation bubbles

Published online by Cambridge University Press:  10 October 2013

Daniel Rodríguez ,
Elmer M. Gennaro  and
Matthew P. Juniper
Daniel Rodríguez*
Affiliation:
School of Aeronautics, Universidad Politécnica de Madrid, Pza. Cardenal Cisneros 3, E-28040 Madrid, Spain Department of Aeronautics, São Carlos School of Engineering, Universidade de São Paulo, Rua Jõao Dagnone 1100, 13563-120, São Carlos, Brazil
Elmer M. Gennaro
Affiliation:
Department of Aeronautics, São Carlos School of Engineering, Universidade de São Paulo, Rua Jõao Dagnone 1100, 13563-120, São Carlos, Brazil
Matthew P. Juniper
Affiliation:
Department of Engineering, University of Cambridge, Trumpington Street, Cambridge CB2 1PZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The self-excited global instability mechanisms existing in flat-plate laminar separation bubbles are studied here, in order to shed light on the causes of unsteadiness and three-dimensionality of unforced, nominally two-dimensional separated flows. The presence of two known linear global mechanisms, namely an oscillator behaviour driven by local regions of absolute inflectional instability and a centrifugal instability giving rise to a steady three-dimensionalization of the bubble, is studied in a series of model separation bubbles. 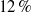These results indicate that absolute instability, and consequently a global oscillator behaviour, does not exist for two-dimensional bubbles with a peak reversed-flow velocity below $12\hspace{0.167em} \% $ of the free-stream velocity. However, the three-dimensional instability becomes active for recirculation levels as low as 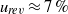${u}_{rev} \approx 7\hspace{0.167em} \% $. These findings suggest a route to the three-dimensionality and unsteadiness observed in experiments and simulations substantially different from that usually found in the literature of laminar separation bubbles, in which two-dimensional vortex shedding is followed by three-dimensionalization.

JFM classification

Instability: Absolute/convective instability Boundary Layers: Boundary layer separation
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 734 , 10 November 2013 , R4
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alam, M. & Sandham, N. D. 2000 Direct numerical simulation of ‘short’ laminar separation bubbles with turbulent reattachment. J. Fluid Mech. 410, 1–28.CrossRefGoogle Scholar
Allen, T. & Riley, N. 1995 Absolute and convective instabilities in separation bubbles. Aeronaut. J. 99, 439–448.CrossRefGoogle Scholar
Amestoy, P. R., Duff, I. S., L’Excellent, J.-Y. & Koster, J. 2001 A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Applics. 23 (1), 15–41.CrossRefGoogle Scholar
Barkley, D., Gomes, M. G. M. & Henderson, R. D. 2002 Three-dimensional instability in a flow over a backward-facing step. J. Fluid Mech. 473, 167–190.CrossRefGoogle Scholar
Beaudoin, J., Cadot, O., Aider, J. & Wesfreid, J. 2004 Three-dimensional stationary flow over a backward-facing step. Eur. J. Mech. (B/Fluids) 23, 147–155.CrossRefGoogle Scholar
Carter, J. 1975 Inverse solutions for laminar boundary-layer flows with separation and reattachment. NASA Tech. Rep. TR R-447.CrossRefGoogle Scholar
Cebeci, T. & Cousteix, J. 2005 Modelling and Computation of Boundary-Layer Flows – Solutions Manual and Computer Programs. Springer.Google Scholar
Dallmann, U. & Schewe, G. 1987 On topological changes of separating flow structures at transition Reynolds numbers. In 16th Fluid Dynamics, Plasma Dynamics and Lasers Conference, Honolulu, HI. AIAA Paper 87-1266.Google Scholar
Diwan, S. S. 2009 Dynamics of early stages of transition in a laminar separation bubble. PhD thesis, Indian Institute of Science, Bangalore, India.Google Scholar
Diwan, S., Chetan, S. & Ramesh, O. 2006 On the bursting criterion for laminar separation bubbles. In Sixth IUTAM Symposium on Laminar–Turbulent Transition (ed. Govindarajan, R.), pp. 401–407. Springer.CrossRefGoogle Scholar
Diwan, S. & Ramesh, O. 2009 On the origin of the inflectional instability of a laminar separation bubble. J. Fluid Mech. 629, 263–298.CrossRefGoogle Scholar
Diwan, S. & Ramesh, O. 2012 Relevance of local parallel theory to the linear stability of laminar separation bubbles. J. Fluid Mech. 698, 468–478.CrossRefGoogle Scholar
Dovgal, A., Kozlov, V. & Michalke, A. 1994 Laminar boundary layer separation: instability and associated phenomena. Prog. Aero. Sci. 3, 61–94.CrossRefGoogle Scholar
Fasel, H. F. & Postl, D. 2004 Interaction of separation and transition of three-dimensional development in boundary layer transition. In Sixth IUTAM Symposium on Laminar-Turbulent Transition (ed. Govindarajan, R.), pp. 71–88. Springer.Google Scholar
Gallaire, F., Marquillie, M. & Ehrenstein, U. 2007 Three-dimensional transverse instabilities in detached boundary layers. J. Fluid Mech. 571, 221–233.CrossRefGoogle Scholar
Gaster, M. 1967 The structure and behaviour of separation bubbles. NPL R & M 3595.Google Scholar
Gaster, M. 2004 Laminar separation bubbles. In Sixth IUTAM Symposium on Laminar-Turbulent Transition (ed. Govindarajan), R.), pp. 1–13. Springer.Google Scholar
Gault, D. 1949 Boundary-layer and stalling characteristics of the NACA 63-009 airfoil section. NACA TN 1894.Google Scholar
Gennaro, E. M., Rodríguez, D., Medeiros, M. A. F. & Theofilis, V. 2013 Sparse techniques in global flow instability with application to compressible leading-edge flow. AIAA J. 51 (9), 2295–2303.CrossRefGoogle Scholar
Gruber, K., Bestek, H. & Fasel, H. 1987 Interaction between a Tollmien–Schlichting wave and a laminar separation bubble. AIAA Paper 87-1256.CrossRefGoogle Scholar
Hammond, D. & Redekopp, L. 1998 Local and global instability properties of separation bubbles. Eur. J. Mech. (B/Fluids) 17, 145–164.CrossRefGoogle Scholar
Huerre, P. & Monkewitz, P. 1985 Absolute and convective instabilities in free shear layers. J. Fluid Mech. 159, 151–168.CrossRefGoogle Scholar
Huerre, P. & Monkewitz, P. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.CrossRefGoogle Scholar
Juniper, M. P., Tammisola, O. & Lundell, F. 2011 The local and global stability of confined planar wakes at intermediate Reynolds number. J. Fluid Mech. 686, 218–238.CrossRefGoogle Scholar
Kitsios, V., Rodríguez, D., Theofilis, V., Ooi, A. & Soria, J. 2009 Biglobal stability analysis in curvilinear coordinates of massively separated lifting bodies. J. Comput. Phys. 228, 7181–7196.CrossRefGoogle Scholar
Marquet, O., Sipp, D., Chomaz, J. & Jacquin, L. 2008 Amplifier and resonator dynamics of a low-Reynolds-number recirculation bubble in a global framework. J. Fluid Mech. 605, 429–443.CrossRefGoogle Scholar
Marxen, O. & Henningson, D. S. 2011 The effect of small-amplitude convective disturbances on the size and bursting of a laminar separation bubble. J. Fluid Mech. 671, 1–33.CrossRefGoogle Scholar
Marxen, O., Lang, M. & Rist, U. 2012 Discrete linear local eigenmodes in a separating laminar boundary layer. J. Fluid Mech. 711, 1–26.CrossRefGoogle Scholar
McCullough, G. & Gault, D. 1951 Examples of three representative types of airfoil-section stall at low speed. NACA TN 2502.Google Scholar
Monkewitz, P., Huerre, P. & Chomaz, J. M. 1993 Global linear stability analysis of weakly non-parallel shear flows. J. Fluid Mech. 251, 1–20.CrossRefGoogle Scholar
Passaggia, P.-Y., Leweke, T. & Ehrenstein, U. 2012 Transverse instability and low-frequency flapping in incompressible separated boundary layer flows: an experimental study. J. Fluid Mech. 703, 363–373.CrossRefGoogle Scholar
Pauley, L., Moin, P. & Reynolds, W. 1990 The structure of two-dimensional separation. J. Fluid Mech. 220, 397–411.CrossRefGoogle Scholar
Rist, U. & Maucher, U. 1994 Direct numerical simulation of 2-d and 3-d instability waves in a laminar separation bubble. In AGARD-CP-551 Application of Direct and Large Eddy Simulation to Transition and Turbulence (ed. Cantwell, B.), pp. 34-1–34-7.Google Scholar
Rist, U. & Maucher, U. 2002 Investigations of time-growing instabilities in laminar separation bubbles. Eur. J. Mech. (B/Fluids) 21, 495–509.CrossRefGoogle Scholar
Rodríguez, D. 2010 Global stability of laminar separation bubbles. PhD thesis, Universidad Politecnica de Madrid.Google Scholar
Rodríguez, D. & Theofilis, V. 2009 Massively parallel numerical solution of the biglobal linear instability eigenvalue problem using dense linear algebra. AIAA J. 47 (10), 2449–2459.CrossRefGoogle Scholar
Rodríguez, D. & Theofilis, V. 2010a On the birth of stall cells on airfoils. Theor. Comput. Fluid Dyn. 25, 105–117.CrossRefGoogle Scholar
Rodríguez, D. & Theofilis, V. 2010b Structural changes of laminar separation bubbles induced by global linear instability. J. Fluid Mech. 655, 280–305.CrossRefGoogle Scholar
Schlichting, H. 1979 Boundary Layer Theory, 7th edn. McGraw-Hill.Google Scholar
Theofilis, V. 2003 Advances in global linear instability analysis of nonparallel and three-dimensional flows. Prog. Aero. Sci. 39, 249–315.CrossRefGoogle Scholar
Theofilis, V. 2011 Global linear stability. Annu. Rev. Fluid Mech. 43, 319–352.CrossRefGoogle Scholar
Theofilis, V., Hein, S. & Dallmann, U. 2000 On the origins of unsteadiness and three-dimensionality in a laminar separation bubble. Phil. Trans. R. Soc. Lond. A 358, 3229–3246.CrossRefGoogle Scholar
Watmuff, J. H. 1999 Evolution of a wave packet into vortex loops in a laminar separation bubble. J. Fluid Mech. 397, 119–169.CrossRefGoogle Scholar
Yon, S. & Katz, J. 1998 Study of the unsteady flow features on a stalled wing. AIAA J. 36 (3), 305–312.CrossRefGoogle Scholar
Zaman, K., McKinzie, D. & Rumsey, C. 1989 A natural low-frequency oscillation of the flow over an airfoil near stalling conditions. J. Fluid Mech. 202, 403–442.CrossRefGoogle Scholar