Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-02-10T16:13:29.708Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent wake past a three-dimensional blunt body. Part 1. Global modes and bi-stability

Published online by Cambridge University Press:  28 March 2013

M. Grandemange ,
M. Gohlke  and
O. Cadot
M. Grandemange*
Affiliation:
Unité de Mécanique, Ecole Nationale Supérieure de Techniques Avancées, ParisTech, Chemin de la Hunière, 91761 Palaiseau CEDEX, France PSA Peugeot Citroën, Centre Technique de Velizy, Route de Gisy, 78943 Vélizy-Villacoublay CEDEX, France
M. Gohlke
Affiliation:
PSA Peugeot Citroën, Centre Technique de Velizy, Route de Gisy, 78943 Vélizy-Villacoublay CEDEX, France
O. Cadot
Affiliation:
Unité de Mécanique, Ecole Nationale Supérieure de Techniques Avancées, ParisTech, Chemin de la Hunière, 91761 Palaiseau CEDEX, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

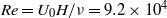The flow around the three-dimensional blunt geometry presented in the work of Ahmed, Ramm & Faitin (Tech. Rep., 1984) is investigated experimentally at $\mathit{Re}= {U}_{0} H/ \nu = 9. 2\times 1{0}^{4} $ (where 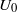${U}_{0} $ is free-stream velocity, 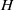$H$ the height of the body and $\nu $ viscosity). The very large recirculation on the base responsible for the dominant part of the drag is characterized. The analyses of the coherent dynamics of the wake reveal the presence of two very distinctive time scales. At long time scales ${T}_{l} \sim 1{0}^{3} H/ {U}_{0} $, the recirculation region shifts between two preferred reflectional-symmetry-breaking positions leading to a statistically symmetric wake; the sequence of these asymmetric states is random. This bi-stable behaviour is independent of the Reynolds number but occurs only above a critical value of ground clearance. At short time scales 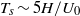${T}_{s} \sim 5H/ {U}_{0} $, the wake presents weak coherent oscillations in the vertical and lateral directions. They are respectively associated with the interaction of the top/bottom and lateral shear layers; when normalized by the height and width of the body, the Strouhal numbers are close to 0.17. These results suggest an alternate shedding associated with the vertical oscillation and a one-sided vortex shedding in the lateral direction with an orientation linked to the current asymmetric position. Finally, the impact of these coherent wake motions on the base pressure is discussed to motivate further drag reduction strategies.

JFM classification

Wakes/Jets: Separated flows Wakes/Jets: Shear layers Wakes/Jets: Wakes/Jets
Type
Papers
Information
Journal of Fluid Mechanics , Volume 722 , 10 May 2013 , pp. 51 - 84
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahmed, S. R., Ramm, G. & Faitin, G. 1984 Some salient features of the time-averaged ground vehicle wake. SAE Tech. Rep. No. 840300, Society of Automotive Engineers, Inc., Warrendale, PA, doi:10.4271/840300.CrossRefGoogle Scholar
Balachandar, S., Mittal, R. & Najjar, F. M. 1997 Properties of the mean recirculation region in the wakes of two-dimensional bluff bodies. J. Fluid Mech. 351, 167–199.CrossRefGoogle Scholar
Bayraktar, I., Landman, D. & Baysal, O. 2001 Experimental and computational investigation of Ahmed body for ground vehicle aerodynamics, SAE paper no. 2001-01-2742.CrossRefGoogle Scholar
Beaudoin, J. F. & Aider, J. L. 2008 Drag and lift reduction of a 3D bluff body using flaps. Exp. Fluids 44 (4), 491–501.CrossRefGoogle Scholar
Beaudoin, J. F., Cadot, O., Aider, J. L., Gosse, K., Paranthoën, P., Hamelin, B., Tissier, M., Allano, D., Mutabazi, I. & Gonzales, M. et al. 2004 Cavitation as a complementary tool for automotive aerodynamics. Exp. Fluids 37 (5), 763–768.CrossRefGoogle Scholar
Berger, E., Scholz, D. & Schumm, M. 1990 Coherent vortex structures in the wake of a sphere and a circular disk at rest and under forced vibrations. J. Fluids Struct. 4 (3), 231–257.CrossRefGoogle Scholar
Bruneau, C. H., Creuse, E., Depeyras, D. & Gillieron, P. 2011 Active procedures to control the flow past the Ahmed body with a 25°rear window. Intl J. Aerodyn. 1 (3), 299–317.CrossRefGoogle Scholar
Bruneau, C. H., Creusé, E., Depeyras, D., Gilliéron, P. & Mortazavi, I. 2010 Coupling active and passive techniques to control the flow past the square back Ahmed body. Comput. Fluids 39 (10), 1875–1892.CrossRefGoogle Scholar
Cadot, O., Thiria, B. & Beaudoin, J. F. 2009 Passive drag control of a turbulent wake by local disturbances. IUTAM Symposium on Unsteady Separated Flows and their Control, Corfu, Greece, June 18–22, 2007. IUTAM Bookseries, vol. 14, pp. 529–537.Google Scholar
Cantwell, B. & Coles, D. 1983 An experimental study of entrainment and transport in the turbulent near wake of a circular cylinder. J. Fluid Mech. 136, 321–374.CrossRefGoogle Scholar
Duell, E. G. & George, A. R. 1993 Measurements in the unsteady near wakes of ground vehicle bodies. SAE Tech. Rep. No. 930298, Society of Automotive Engineers, 400 Commonwealth Dr, Warrendale, PA, 15096, USA.CrossRefGoogle Scholar
Duell, E. G. & George, A. R. 1999 Experimental study of a ground vehicle body unsteady near wake. SAE Trans. 108 (6; Part 1), 1589–1602.Google Scholar
Fourrié, G., Keirsbulck, L., Labraga, L. & Gilliéron, P. 2011 Bluff-body drag reduction using a deflector. Exp. Fluids 50 (2), 385–395.CrossRefGoogle Scholar
Franck, G., Nigro, N., Storti, M. & Delıa, J. 2009 Numerical simulation of the Ahmed vehicle model near-wake. Latin Am. Appl. Res. 39 (4), 295–306.Google Scholar
Gilliéron, P. & Kourta, A. 2010 Aerodynamic drag reduction by vertical splitter plates. Exp. Fluids 48 (1), 1–16.CrossRefGoogle Scholar
Grandemange, M., Cadot, O. & Gohlke, M. 2012a Reflectional symmetry breaking of the separated flow over three-dimensional bluff bodies. Phys. Rev. E 86, 035302.CrossRefGoogle ScholarPubMed
Grandemange, M., Parezanović, V., Gohlke, M. & Cadot, O. 2012b On experimental sensitivity analysis of the turbulent wake from an axisymmetric blunt trailing edge. Phys. Fluids 24, 035106.CrossRefGoogle Scholar
Greenblatt, D. & Wygnanski, I. J. 2000 The control of flow separation by periodic excitation. Prog. Aeronaut. Sci. 36 (7), 487–545.CrossRefGoogle Scholar
Herry, B. B., Keirsbulck, L. & Paquet, J. B. 2011 Flow bistability downstream of three-dimensional double backward facing steps at zero-degree slideslip. Trans. ASME: J. Fluids Engng 133, 1–4.Google Scholar
Hucho, W. H. 1998 Aerodynamics of Road Vehicles. SAE.Google Scholar
Khalighi, B., Chen, K. H. & Laccarino, G. 2012 Unsteady aerodynamic flow investigation around a simplified square-back road vehicle with drag reduction devices. Trans. ASME: J. Fluids Engng 134 (6) 061101 (16 pages).Google Scholar
Khalighi, B., Zhang, S., Koromilas, C., Balkanyi, S. R., Bernal, L. P., Iaccarino, G. & Moin, P. 2001 Experimental and computational study of unsteady wake flow behind a bluff body with a drag reduction device. SAE Paper 2001-01-1042.CrossRefGoogle Scholar
Kiya, M. & Abe, Y. 1999 Turbulent elliptic wakes. J. Fluids Struct. 13 (7–8), 1041–1067.CrossRefGoogle Scholar
Krajnović, S. & Davidson, L. 2005 Flow around a simplified car, part 2: understanding the flow. Trans. ASME: J. Fluids Engng 127, 919–928.Google Scholar
Lawson, N. J., Garry, K. P. & Faucompret, N. 2007 An investigation of the flow characteristics in the bootdeck region of a scale model notchback saloon vehicle. Proc. Inst. Mech. Engrs D: J. Automobile Engng 221 (D6), 739–754.CrossRefGoogle Scholar
Lienhart, H. & Becker, S. 2003 Flow and turbulence structure in the wake of a simplified car model. SAE Trans. 112 (6), 785–796.Google Scholar
Littlewood, R. & Passmore, M. 2010 The optimization of roof trailing edge geometry of a simple square-back. SAE Paper 2010-01-0510, pp. 151–160.Google Scholar
Pao, H. P. & Kao, T. W. 1977 Vortex structure in the wake of a sphere. Phys. Fluids 20, 187–191.CrossRefGoogle Scholar
Parezanović, V. & Cadot, O. 2012 Experimental sensitivity analysis of the global properties of a 2D turbulent wake. J. Fluid Mech. 693, 115–149.CrossRefGoogle Scholar
Pujals, G., Depardon, S. & Cossu, C. 2010 Drag reduction of a 3d bluff body using coherent streamwise streaks. Exp. Fluids 49 (5), 1085–1094.CrossRefGoogle Scholar
Roshko, A. 1993 Perspectives on bluff body aerodynamics. J. Wind Engng Ind. Aerodyn. 49 (1–3), 79–100.CrossRefGoogle Scholar
Rouméas, M., Gilliéron, P. & Kourta, A. 2009 Analysis and control of the near-wake flow over a square-back geometry. Comput. Fluids 38 (1), 60–70.CrossRefGoogle Scholar
Ruiz, T., Sicot, C., Brizzi, L. E., Laumonier, J., Borée, J. & Gervais, Y. 2009 Unsteady near wake of a flat disk normal to a wall. Exp. Fluids 47 (4), 637–653.CrossRefGoogle Scholar
Sakamoto, H. & Haniu, H. 1990 A study on vortex shedding from spheres in a uniform flow. Trans. ASME: J. Fluids Engng 112, 386–392.Google Scholar
Spohn, A. & Gilliéron, P. 2002 Flow separations generated by a simplified geometry of an automotive vehicle. In Congress IUTAM Symposium on Unsteady Separated Flows (Toulouse, France, 8–12 April 2002).Google Scholar
Taneda, S. 1978 Visual observations of the flow past a sphere at Reynolds numbers between 104 and 106. J. Fluid Mech. 85 (1), 187–192.CrossRefGoogle Scholar
Wassen, E., Eichinger, S. & Thiele, F. 2010 Simulation of active drag reduction for a square-back vehicle. Active Flow Control II 241–255.CrossRefGoogle Scholar
Waudby-Smith, P., Bender, T. & Vigneron, R. 2004 The GIE S2A full-scale aero-acoustic wind tunnel. SAE Trans. 113 (6), 449–461.Google Scholar
Yun, G., Kim, D. & Choi, H. 2006 Vortical structures behind a sphere at subcritical Reynolds numbers. Phys. Fluids 18, 015102.CrossRefGoogle Scholar