Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-12-02T22:13:22.526Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulence modifications induced by the bed mobility in intense sediment-laden flows

Published online by Cambridge University Press:  02 November 2016

T. Revil-Baudard ,
J. Chauchat ,
D. Hurther  and
O. Eiff
T. Revil-Baudard
Affiliation:
Institut fur Hydromechanik, Kaiserstr.12, D-76131 Karlsruhe, Germany LEGI, Grenoble University, Domaine Universitaire, BP 53, 38041 Grenoble CEDEX 9, France
J. Chauchat*
Affiliation:
LEGI, Grenoble University, Domaine Universitaire, BP 53, 38041 Grenoble CEDEX 9, France
D. Hurther
Affiliation:
LEGI, Grenoble University, Domaine Universitaire, BP 53, 38041 Grenoble CEDEX 9, France
O. Eiff
Affiliation:
Institut fur Hydromechanik, Kaiserstr.12, D-76131 Karlsruhe, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

An experimental dataset of high-resolution velocity and concentration measurements is obtained under intense sediment transport regimes to provide new insights into the modification of turbulence induced by the presence of a mobile sediment bed. The physical interpretation of the zero-plane level in the law of the wall is linked to the bed-level variability induced by large-scale turbulent flow structures. 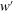The comparison between intrinsic and superficial Reynolds shear stresses shows that the observed strong bed-level variability results in an increased covariance between wall-normal ($w^{\prime }$) and streamwise (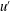$u^{\prime }$) velocity fluctuations. This appears as an additional Reynolds shear stress in the near-wall region. It is also observed that the mobile sediment bed induces an increase of turbulence kinetic energy (TKE) across the boundary layer. However, the increased contribution of interaction events ($u^{\prime }w^{\prime }>0$, i.e. quadrants I and III in the ($u^{\prime },w^{\prime }$) plane) induces a decrease of the turbulent momentum diffusion and an increase of the turbulent concentration diffusion in the suspension region. This result provides an explanation for the modification of the von Kármán parameter and the turbulent Schmidt number observed in the literature for intense sediment transport.

JFM classification

Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows Geophysical and Geological Flows: Sediment transport Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 808 , 10 December 2016 , pp. 469 - 484
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bagnold, R. A. 1956 The flow of cohesionless grains in fluids. Phil. Trans. R. Soc. Lond. 249, 235–297.Google Scholar
Best, J., Bennett, S., Bridge, J. & Leeder, M. 1997 Turbulence modulation and particle velocities over flat sand beds at low transport rates. ASCE J. Hydraul. Engng 123 (12), 1118–1129.CrossRefGoogle Scholar
Camenen, B., Bayram, A. & Larson, M. 2006 Equivalent roughness height for plane bed under steady flow. ASCE J. Hydraul. Engng 132 (11), 1146–1158.CrossRefGoogle Scholar
Capart, H. & Fraccarollo, L. 2011 Transport layer structure in intense bed-load. Geophys. Res. Lett. 38 (20), L20402.Google Scholar
Castro-Orgaz, O., Giráldez, J. V., Mateos, L. & Dey, S. 2012 Is the von Kármán constant affected by sediment suspension? J. Geophys. Res.: Earth Surface 117, F04002.CrossRefGoogle Scholar
Derksen, J. J. 2015 Simulations of granular bed erosion due to a mildly turbulent shear flow. J. Hydraul. Res. 53 (5), 622–632.Google Scholar
Gao, P. & Abrahams, A. D. 2004 Bedload transport resistance in rough open-channel flows. Earth Surf. Process. Landf. 29 (4), 423–435.CrossRefGoogle Scholar
Garbini, J. L., Forster, F. K. & Jorgensen, J. E. 1982 Measurement of fluid turbulence based on pulsed ultrasound techniques. Part 2. Experimental investigation. J. Fluid Mech. 118, 471–505.Google Scholar
Gaudio, R., Miglio, A. & Dey, S. 2010 Non-universality of von Kármán’s 𝜅 in fluvial streams. J. Hydraul. Res. 48 (5), 658–663.CrossRefGoogle Scholar
Greimann, B. P., Muste, M. & Holly, F. M. Jr. 1999 Two-phase formulation of suspended sediment transport. J. Hydraul. Res. 37, 479–500.Google Scholar
Hanes, D. M. & Bowen, A. J. 1985 A granular-fluid model for steady intense bed-load transport. J. Geophys. Res. 90, 9149–9158.CrossRefGoogle Scholar
Hsu, T. J., Jenkins, J. T. & Liu, L. F. 2003 On two-phase sediment transport: dilute flow. J. Geophys. Res. 108 (C3), 3057.Google Scholar
Hsu, T. J., Jenkins, J. T. & Liu, P. L.-F. 2004 On two-phase sediment transport: sheet flow of massive particles. Proc. R. Soc. Lond. A 460 (2048), 2223–2250.CrossRefGoogle Scholar
Hurther, D., Lemmin, U. & Terray, E. A. 2007 Turbulent transport in the outer region of rough-wall open-channel flows: the contribution of large coherent shear stress structures (LC3S). J. Fluid Mech. 574, 465–493.Google Scholar
Hurther, D. & Thorne, P. D. 2011 Suspension and near-bed load sediment transport processes above a migrating, sand-rippled bed under shoaling waves. J. Geophys. Res.: Oceans 116, C07001.CrossRefGoogle Scholar
Jackson, P. S. 1981 On the displacement height in the logarithmic velocity profile. J. Fluid Mech. 111, 15–25.CrossRefGoogle Scholar
Jenkins, J. T. & Hanes, D. M. 1998 Collisional sheet flows of sediment driven by a turbulent fluid. J. Fluid Mech. 370, 29–52.CrossRefGoogle Scholar
Jiménez, J. 2004 Turbulent flows over rough walls. Annu. Rev. Fluid Mech. 36 (1), 173–196.CrossRefGoogle Scholar
Kaftori, D., Hestroni, G. & Banerjee, S. 1996 Particle behavior in the turbulent boundary layer. Velocity and Distribution profiles. Phys. Fluids 7, 1107–1121.Google Scholar
Kidanemariam, A. G., Chan-Braun, C., Doychev, T. & Uhlmann, M. 2013 Direct numerical simulation of horizontal open channel flow with finite-size, heavy particles at low solid volume fraction. New J. Phys. 15 (2), 025031.Google Scholar
Lu, S. S. & Willmarth, W. W. 1973 Measurements of the structure of the Reynolds stress in a turbulent boundary layer. J. Fluid Mech. 60, 481–511.CrossRefGoogle Scholar
Manes, C., Poggi, D. & Ridolfi, L. 2011 Turbulent boundary layers over permeable walls: scaling and near-wall structure. J. Fluid Mech. 687, 141–170.Google Scholar
Mignot, E., Barthélémy, E. & Hurther, D. 2009a Double-averaging analysis and local flow characterization of near-bed turbulence in gravel-bed channel flows. J. Fluid Mech. 618, 279–303.CrossRefGoogle Scholar
Mignot, E., Hurther, D. & Barthélémy, E. 2009b On the structure of shear stress and turbulent kinetic energy flux across the roughness layer of a gravel-bed channel flow. J. Fluid Mech. 638, 423–452.Google Scholar
Nakagawa, H. & Nezu, I. 1977 Prediction of the contributions to the Reynolds stress from bursting events in open-channel flows. J. Fluid Mech. 80, 99–128.Google Scholar
Nezu, I. & Nakagava, H. 1993 Turbulence in Open-channel Flows. IAHR.Google Scholar
Niño, Y. & Garcia, M. H. 1996 Experiments on particle-turbulence interactions in the near-wall region of an open channel flow: implications for sediment transport. J. Fluid Mech. 326, 285–319.CrossRefGoogle Scholar
Nielsen, P. & Teakle, I. A. L. 2004 Turbulent diffusion of momentum and suspended particles: A finite-mixing-length theory. Phys. Fluids 16 (7), 2342–2348.Google Scholar
Nikora, V., Ballio, F., Coleman, S. & Pokrajac, D. 2013 Spatially averaged flows over mobile rough beds: definitions, averaging theorems, and conservation equations. ASCE J. Hydraul. Engng 139 (8), 803–811.CrossRefGoogle Scholar
Nikora, V., Goring, D., Mcewan, I. & Griffiths, G. 2001 Spatially averaged open-channel flow over rough bed. ASCE J. Hydraul. Engng 127 (2), 123–133.Google Scholar
Nikora, V., Koll, K., Mclean, S., Dittrich, A. & Aberle, J. 2002 Zero-plane displacement for rough-bed open-channel flows. In Proceedings of the International Conference on Fluvial Hydraulics River Flow, pp. 83–92.Google Scholar
Nnadi, F. N. & Wilson, K. C. 1992 Motion of contact-load particles at high shear stress. ASCE J. Hydraul. Engng 118 (12), 1670–1684.Google Scholar
Pokrajac, D., Mcewan, I. & Nikora, V. 2008 Spatially averaged turbulent stress and its partitioning. Exp. Fluids 45 (1), 73–83.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Radice, A. & Ballio, F. 2008 Double-average characteristics of sediment motion in one-dimensional bed load. Acta Geophys. 56 (3), 654–668.Google Scholar
Raupach, M. R. 1981 Conditional statistics of Reynolds stress in rough-wall and smooth-wall turbulent boundary layers. J. Fluid Mech. 108, 363–382.CrossRefGoogle Scholar
Recking, A. 2010 A comparison between flume and field bed load transport data and consequences for surface-based bed load transport prediction. Water Resour. Res. 46 (3), W03518.Google Scholar
Recking, A., Frey, P., Paquier, A., Belleudy, P. & Champagne, J. Y. 2008 Feedback between bed load transport and flow resistance in gravel and cobble bed rivers. Water Resour. Res. 44, W03518.Google Scholar
Revil-Baudard, T. & Chauchat, J. 2013 A two-phase model for sheet flow regime based on dense granular flow rheology. J. Geophys. Res.: Oceans 118 (2), 619–634.CrossRefGoogle Scholar
Revil-Baudard, T., Chauchat, J., Hurther, D. & Barraud, P.-A. 2015 Investigation of sheet-flow processes based on novel acoustic high-resolution velocity and concentration measurements. J. Fluid Mech. 767, 1–30.CrossRefGoogle Scholar
Rouse, H. 1937 Modern conceptions of the mechanics of turbulence. Trans. Am. Soc. Civ. Eng. 102, 463–505.CrossRefGoogle Scholar
Shao, X., Wu, T. & Yu, Z. 2012 Fully resolved numerical simulation of particle-laden turbulent flow in a horizontal channel at a low Reynolds number. J. Fluid Mech. 693, 319–344.Google Scholar
Sumer, B. M., Kozakiewicz, A., Fredsøe, J. & Deigaard, R. 1996 Velocity and concentration profiles in sheet-flow layer of movable bed. ASCE J. Hydraul. Engng 122 (10), 549–558.CrossRefGoogle Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Vanoni, V. A. E. J. 1946 Transportation of suspended sediment by running water. Trans. Am. Soc. Civ. Eng. 111, 67–133.Google Scholar
Wilson, K. C. 1989 Mobile-bed friction at high shear stress. ASCE J. Hydraul. Engng 115 (6), 825–830.Google Scholar