Topology of interacting coiled vortex rings

Abstract

Pairs of nested vortex rings, one with coils, are evolved numerically to compare their topological numbers to those of recent experiments reported in Scheeler et al. (Science, vol. 357, 2017, pp. 487–491). Included are the twist 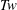$Tw$, writhe 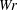$Wr$ and self-linking 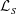${\mathcal{L}}_{S}$ numbers, plus centreline helicities 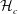${\mathcal{H}}_{c}$. The questions are: can the experimental numbers be validated and do these numbers have roles in the dynamics of the global helicities 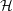${\mathcal{H}}$ and enstrophies $Z$ with respect to cascades? Topological analysis of the experiments $t=0$ analytic centreline vortex trajectories validates only the writhe measurements, not their values of 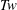$Tw$ and 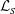${\mathcal{L}}_{S}$, which obey $Tw\lesssim {\mathcal{L}}_{S}=m\gg Wr$ for 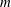$m$-coil rings. Not $Tw\ll Wr$. To suggest why the large twists do not contribute to ${\mathcal{H}}$, it is noted that the mapping of the coiled rings onto the mesh is to a first approximation a single pair of Clebsch potentials, whose self-helicity 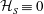${\mathcal{H}}_{S}\equiv 0$. Numerical rings with circulations $\unicode[STIX]{x1D6E4}$, including some single rings, show small initial helicities with 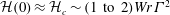${\mathcal{H}}(0)\approx {\mathcal{H}}_{c}\sim (\text{1 to 2})Wr\unicode[STIX]{x1D6E4}^{2}$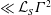$\ll {\mathcal{L}}_{S}\unicode[STIX]{x1D6E4}^{2}$. For time and velocity scales that are consistent with the experiments, as the coils evolve, their 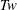$Tw$, $Wr$, ${\mathcal{L}}_{S}$ numbers and their helicities are nearly static until reconnection. Nonetheless, 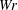$Wr$ and 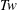$Tw$ retain important complementary roles in the dynamics of the global helicity 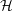${\mathcal{H}}$ and enstrophy 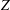$Z$, with the evolution of the torsion 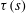$\unicode[STIX]{x1D70F}(s)$ profile showing the beginnings of a cascade to small scales.