Article contents
Tip-vortex instability and turbulent mixing in wind-turbine wakes
Published online by Cambridge University Press: 23 September 2015
Abstract
Kinetic-energy transport and turbulence production within the shear layer of a horizontal-axis wind-turbine wake are investigated with respect to their influence on the tip-vortex pairwise instability, the so-called leapfrogging instability. The study quantifies the effect of near-wake instability and tip-vortex breakdown on the process of mean-flow kinetic-energy transport within the far wake of the wind turbine, in turn affecting the wake re-energising process. Experiments are conducted in an open-jet wind tunnel with a wind-turbine model of 60 cm diameter at a diameter-based Reynolds number range 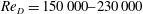 $\mathit{Re}_{D}=150\,000{-}230\,000$. The velocity fields in meridian planes encompassing a large portion of the wake past the rotor are measured both in the unconditioned and the phase-locked mode by means of stereoscopic particle image velocimetry. The detailed topology and development of the tip-vortex interactions are discussed prior to a statistical analysis based on the triple decomposition of the turbulent flow fields. The study emphasises the role of the pairing instability as a precursor to the onset of three-dimensional vortex distortion and breakdown, leading to increased turbulent mixing and kinetic-energy transport across the shear layer. Quadrant analysis further elucidates the role of sweep and ejection events within the two identified mixing regimes. Prior to the onset of the instability, vortices shed from the blade appear to inhibit turbulent mixing of the expanding wake. The second region is dominated by the leapfrogging instability, with a sudden increase of the net entrainment of kinetic energy. Downstream of the latter, random turbulent motion characterises the flow, with a significant increase of turbulent kinetic-energy production. In this scenario, the leapfrogging mechanism is recognised as the triggering event that accelerates the onset of efficient turbulent mixing followed by the beginning of the wake re-energising process.
$\mathit{Re}_{D}=150\,000{-}230\,000$. The velocity fields in meridian planes encompassing a large portion of the wake past the rotor are measured both in the unconditioned and the phase-locked mode by means of stereoscopic particle image velocimetry. The detailed topology and development of the tip-vortex interactions are discussed prior to a statistical analysis based on the triple decomposition of the turbulent flow fields. The study emphasises the role of the pairing instability as a precursor to the onset of three-dimensional vortex distortion and breakdown, leading to increased turbulent mixing and kinetic-energy transport across the shear layer. Quadrant analysis further elucidates the role of sweep and ejection events within the two identified mixing regimes. Prior to the onset of the instability, vortices shed from the blade appear to inhibit turbulent mixing of the expanding wake. The second region is dominated by the leapfrogging instability, with a sudden increase of the net entrainment of kinetic energy. Downstream of the latter, random turbulent motion characterises the flow, with a significant increase of turbulent kinetic-energy production. In this scenario, the leapfrogging mechanism is recognised as the triggering event that accelerates the onset of efficient turbulent mixing followed by the beginning of the wake re-energising process.
JFM classification
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
References
- 115
- Cited by



