Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-24T18:44:22.698Z Has data issue: false hasContentIssue false
  • English
  • Français

Three-dimensional wave packet in a Mach 6 boundary layer on a flared cone

Published online by Cambridge University Press:  27 December 2019

Christoph Hader Open the ORCID record for Christoph Hader [Opens in a new window]  and
Hermann F. Fasel
Christoph Hader*
Affiliation:
Department of Aerospace and Mechanical Engineering, University of Arizona, Tucson, AZ85721, USA
Hermann F. Fasel
Affiliation:
Department of Aerospace and Mechanical Engineering, University of Arizona, Tucson, AZ85721, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

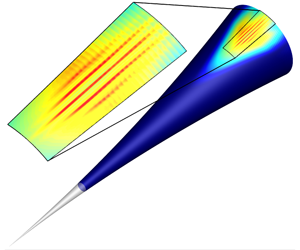
High-resolution direct numerical simulations (DNS) were carried out to investigate the nonlinear breakdown process of a three-dimensional wave packet initiated by a short-duration pulse in a flared cone boundary layer at Mach 6 and zero angle of attack. For these simulations the cone geometry of the flared cone experiments conducted in the Boeing/AFOSR Mach 6 Quiet Tunnel (BAM6QT) at Purdue University was considered. The computational domain covered a large extent of the cone in the azimuthal direction to allow for a wide range of azimuthal wavenumbers ($k_{c}$) and to include shallow instability waves with small azimuthal wavenumbers. The simulation results indicated that the wave packet development was dominated by axisymmetric and shallow (small $k_{c}$) second-mode waves for a large downstream extent. Towards the downstream end of the computational domain a rapid broadening of the disturbance amplitude spectra was observed, which is an indication that the wave packet reached the strongly nonlinear stages. The disturbance spectra of the nonlinear regime, and the downstream amplitude development of the dominant disturbance wave components, provided conclusive evidence that the so-called fundamental breakdown was the dominant nonlinear mechanism. Furthermore, contours of the time-averaged Stanton number exhibited ‘hot’ streaks within the wave packet on the surface of the cone. Hot streaks have also been observed in the Purdue flared cone experiments using temperature sensitive paint (TSP) and in numerical investigations using DNS. The azimuthal streak spacing obtained from the wave packet simulation agrees well with that observed in the Purdue quiet tunnel experiments.

JFM classification

Aerodynamics: High-speed flow Boundary Layers: Boundary layer stability Instability: Transition to turbulence
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 885 , 25 February 2020 , R3
Copyright
© The Author(s), 2019. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bushnell, D. 1990 Notes on initial disturbance fields for the transition problem. In Instability and Transition, pp. 217–232. Springer.Google Scholar
Casper, K. M., Beresh, S. J., Henfling, J., Spillers, R. & Pruett, B. 2013 High-speed schlieren imaging of disturbances in a transitional hypersonic boundary layer. In 51st AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, AIAA Paper 2013-0376.Google Scholar
Casper, K. M., Beresh, S. J. & Schneider, S. P. 2014 Pressure fluctuations beneath instability wavepackets and turbulent spots in a hypersonic boundary layer. J. Fluid Mech. 756, 1058–1091.CrossRefGoogle Scholar
Chuvakhov, P. V., Fedorov, A. V. & Obraz, A. O. 2018 Numerical simulation of turbulent spots generated by unstable wave packets in a hypersonic boundary layer. Comput. Fluids 162, 26–38.CrossRefGoogle Scholar
Chuvakhov, P. V., Fedorov, A. V. & Obraz, A. O. 2019 Numerical modelling of supersonic boundary-layer receptivity to solid particulates. J. Fluid Mech. 869, 949–971.CrossRefGoogle Scholar
Chynoweth, B.2018 Measurements of transition dominated by the second-mode instability at Mach 6. PhD thesis, Purdue University, West Lafayette, IN.Google Scholar
Chynoweth, B. C., Schneider, S. P., Hader, C., Fasel, H. F., Batista, A., Kuehl, J., Juliano, T. J. & Wheaton, B. M. 2019 History and progress of boundary-layer transition on a Mach-6 flared cone. J. Spacecr. Rockets 56 (2), 333–346.CrossRefGoogle Scholar
Fedorov, A. V. 2013 Receptivity of a supersonic boundary layer to solid particulates. J. Fluid Mech. 737, 105–131.CrossRefGoogle Scholar
Gross, A. & Fasel, H. F. 2008 High-order accurate numerical method for complex flows. AIAA J. 46 (1), 204–214.CrossRefGoogle Scholar
Hader, C. & Fasel, H. F. 2018 Towards simulating natural transition in hypersonic boundary layers via random inflow disturbances. J. Fluid Mech. 847, R3.CrossRefGoogle Scholar
Hader, C. & Fasel, H. F. 2019 Direct numerical simulations of hypersonic boundary-layer transition for a flared cone: fundamental breakdown. J. Fluid Mech. 869, 341–384.CrossRefGoogle Scholar
Jewell, J. S., Leyva, I. A. & Shepherd, J. E. 2017 Turbulent spots in hypervelocity flow. Exp. Fluids 58 (4), 32.CrossRefGoogle Scholar
Jewell, J. S., Parziale, N. J., Leyva, I. A. & Shepherd, J. E. 2016 Effects of shock-tube cleanliness on hypersonic boundary layer transition at high enthalpy. AIAA J. 55 (1), 332–338.CrossRefGoogle Scholar
Jocksch, A. & Kleiser, L. 2008 Growth of turbulent spots in high-speed boundary layers on a flat plate. Intl J. Heat Fluid Flow 29 (6), 1543–1557.CrossRefGoogle Scholar
Krishnan, L. & Sandham, N. D. 2006a Effect of Mach number on the structure of turbulent spots. J. Fluid Mech. 566, 225–234.CrossRefGoogle Scholar
Krishnan, L. & Sandham, N. D. 2006b Turbulent spots in a compressible boundary-layer flow. In IUTAM Symposium on Laminar-Turbulent Transition, pp. 329–334. Springer.CrossRefGoogle Scholar
Krishnan, L. & Sandham, N. D. 2006c On the merging of turbulent spots in a supersonic boundary-layer flow. Intl J. Heat Fluid Flow 27 (4), 542–550.CrossRefGoogle Scholar
Laible, A. & Fasel, H. F.2011 Numerical investigation of hypersonic transition for a flared and a straight cone at Mach 6. AIAA Paper 2011-3565.CrossRefGoogle Scholar
Laible, A. C.2011 Numerical investigation of boundary layer transition for cones at Mach 3.5 and 6.0. PhD thesis, The University of Arizona, Tucson, AZ.Google Scholar
Marineau, E. C. 2016 Prediction methodology for second-mode-dominated boundary-layer transition in wind tunnels. AIAA J. 55 (2), 484–499.CrossRefGoogle Scholar
Marineau, E. C., Grossir, G., Wagner, A., Leinemann, M., Radespiel, R., Tanno, H., Wadhams, T. P., Chynoweth, B. C., Schneider, S. P., Wagnild, R. W. et al. 2018 Compilation and analysis of second-mode amplitudes on sharp cones in hypersonic wind tunnels. In 2018 AIAA Aerospace Sciences Meeting, AIAA Paper 2018-0349.Google Scholar
Mayer, C. S. J., Laible, A. C. & Fasel, H. F.2009 Numerical investigation of transition initiated by a wave packet on a cone at Mach 3.5. AIAA Paper 2009-3809.CrossRefGoogle Scholar
Mayer, C. S. J., Laible, A. C. & Fasel, H. F. 2011a Numerical investigation of wave packets in a Mach 3.5 cone boundary layer. AIAA J. 49 (1), 67–86.CrossRefGoogle Scholar
Mayer, C. S. J., Von Terzi, D. A. & Fasel, H. F. 2011b Direct numerical simulation of complete transition to turbulence via oblique breakdown at Mach 3. J. Fluid Mech. 674, 5–42.CrossRefGoogle Scholar
Meitz, H. L.1996 Numerical investigation of suction in a transitional flat-plate boundary layer. PhD thesis, The University of Arizona, Tucson, AZ.Google Scholar
Redford, J. A., Sandham, N. D. & Roberts, G. T. 2012 Numerical simulations of turbulent spots in supersonic boundary layers: effects of Mach number and wall temperature. Prog. Aerosp. Sci. 52, 67–79.CrossRefGoogle Scholar
Salemi, L., Fasel, H. F., Wernz, S. H. & Marquart, E. 2014 Numerical investigation of wavepackets in a hypersonic high-enthalpy boundary layer on a 5deg sharp cone. In 7th AIAA Theoretical Fluid Mechanics Conference, AIAA Paper 2014-2775.Google Scholar
Salemi, L. & Fasel, H. F. 2015 Numerical investigation of nonlinear wave-packets in a hypersonic high-enthalpy boundary-layer on a 5° sharp cone. In 45th AIAA Thermo-Physics Conference, AIAA Paper 2015-2318.Google Scholar
Sivasubramanian, J. & Fasel, H. F.2012 Growth and breakdown of a wave packet into a turbulent spot in a cone boundary layer at Mach 6. AIAA Paper 2012-0085.CrossRefGoogle Scholar
Sivasubramanian, J. & Fasel, H. F. 2014 Numerical investigation of the development of three-dimensional wavepackets in a sharp cone boundary layer at Mach 6. J. Fluid Mech. 756, 600–649.CrossRefGoogle Scholar
Sivasubramanian, J. & Fasel, H. F. 2015 Direct numerical simulation of transition in a sharp cone boundary layer at Mach 6: fundamental breakdown. J. Fluid Mech. 768, 175–218.CrossRefGoogle Scholar
White, F. M. 2006 Viscous Fluid Flow, intl edn. McGraw–Hill.Google Scholar

Hader and Fasel supplementary movie

Derivative of the temperature with respect to y for the instantaneous flow field (right half of the cone) and of the time-averaged flow field (left half of the cone).

Download Hader and Fasel supplementary movie(Video)
Video 3.3 MB