Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-24T19:37:23.776Z Has data issue: false hasContentIssue false
  • English
  • Français

Three-dimensional organization and dynamics of vortices in multichannel swirling jets

Published online by Cambridge University Press:  21 March 2018

Andrea Ianiro Open the ORCID record for Andrea Ianiro [Opens in a new window] ,
Kyle P. Lynch ,
Daniele Violato ,
Gennaro Cardone  and
Fulvio Scarano
Andrea Ianiro*
Affiliation:
Aerospace Engineering Group, Universidad Carlos III de Madrid, Av. de la Universidad 30, 28911 Leganés, Spain
Kyle P. Lynch
Affiliation:
Aerospace Engineering Department, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
Daniele Violato
Affiliation:
Aerospace Engineering Department, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
Gennaro Cardone
Affiliation:
Dipartimento di Ingegneria Industriale – Sezione Aerospaziale, University of Naples ‘Federico II’, Naples 80125, Italy
Fulvio Scarano
Affiliation:
Aerospace Engineering Department, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The unsteady three-dimensional flow organization of jets issued from a duct with swirl vanes at Reynolds number equal to 1000 and swirl number 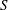$S$ ranging between 0 and 0.8 is investigated. Time-resolved tomographic particle image velocimetry returns the instantaneous flow structure and its evolution by visualization of velocity and vortical features. The most relevant coherent motions are identified and characterized with the aid of dynamic mode decomposition. The time-averaged flow topology indicates that the vanes used to impart the swirling motion have a significant impact on the azimuthal modulation of momentum, with the jet exhibiting four sectors separated by a thin cross-like wake resulting from the boundary layer developed along the vane walls. The flow field is thus characterized by inner and outer shear regions. An increase in swirl, up to moderate levels (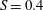$S=0.4$), causes larger jet spreading angles. Further increase of the swirl number is accompanied by the appearance of a central recirculation zone due to vortex breakdown at $S=0.6$ which increases in size and is triggered upstream for increasing 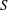$S$. Although no shear layer instability development is observed at 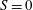$S=0$, already at $S=0.2$ the swirling motion promotes the growth of helical vortices appearing as Kelvin–Helmholtz waves that deform the outer axial shear layer. The downstream evolution features successive pairing, which is observed for all the considered swirl numbers. The initial development of the instability is independent for each vane, whereas a mutual interaction between the vanes occurs after the vortex pairing. The reconnection from the four sectors vortices induces a significant increase of azimuthal vorticity, which affects the dynamical behaviour of the precessing vortex core. The latter is visualized by a low-order spatio-temporal reconstruction based on few dynamical modes. At a higher swirl number ($S\geqslant 0.6$), the axial vorticity component dominates the flow field; it interacts with the azimuthal vorticity, which penetrates inward through the meanders of the vane wakes and forces the vortex core precession and breakdown.

JFM classification

Wakes/Jets: Jets Vortex Flows: Vortex breakdown Vortex Flows: Vortex interactions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 843 , 25 May 2018 , pp. 180 - 210
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: Currently a postdoctoral appointee at Sandia National Laboratories, Albuquerque, NM, USA 87185.

§

Present address: Currently employed by the Innovation and Networks Executive Agency of the European Commission, Chaussée de Wavre 910, 1040 Etterbeek, Brussels, Belgium.

References

Beavers, G. S. & Wilson, T. A. 1970 Vortex growth in jets. J. Fluid Mech. 44 (1), 97–112.Google Scholar
Billant, P., Chomaz, J. M. & Huerre, P. 1998 Experimental study of vortex breakdown in swirling jets. J. Fluid Mech. 376, 183–219.10.1017/S0022112098002870Google Scholar
Brown, G. L. & Lopez, J. M. 1990 Axisymmetric vortex breakdown. Part 2. Physical mechanisms. J. Fluid Mech. 221, 553–576.Google Scholar
Cala, C. E., Fernandes, E., Heitor, M. V. & Shtork, S. I. 2006 Coherent structures in unsteady swirling jet flow. Exp. Fluids 40 (2), 267–276.10.1007/s00348-005-0066-9Google Scholar
Candel, S., Durox, D., Schuller, T., Bourgouin, J. F. & Moeck, J. P. 2014 Dynamics of swirling flames. Annu. Rev. Fluid Mech. 46, 147–173.10.1146/annurev-fluid-010313-141300Google Scholar
Ceglia, G., Discetti, S., Ianiro, A., Michaelis, D., Astarita, T. & Cardone, G. 2014 Three-dimensional organization of the flow structure in a non-reactive model aero engine lean burn injection system. Exp. Therm. Fluid Sci. 52, 164–173.Google Scholar
Chanaud, R. C. 1965 Observations of oscillatory motion in certain swirling flows. J. Fluid Mech. 21 (1), 111–127.Google Scholar
Chigier, N. A. & Chervinsky, A. 1967 Aerodynamic study of turbulent burning free jets with swirl. In Symposium (International) on Combustion, vol. 11, no. 1, pp. 489–499. Elsevier.Google Scholar
Childs, P. R. 2010 Rotating Flow. Elsevier.Google Scholar
Davidson, P. 2015 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Elkins, C. J., Markl, M., Pelc, N. & Eaton, J. K. 2003 4D Magnetic resonance velocimetry for mean velocity measurements in complex turbulent flows. Exp. Fluids 34 (4), 494–503.Google Scholar
Elsinga, G. E., Scarano, F., Wieneke, B. & van Oudheusden, B. W. 2006 Tomographic particle image velocimetry. Exp. Fluids 41 (6), 933–947.10.1007/s00348-006-0212-zGoogle Scholar
Elsinga, G. E., Westerweel, J., Scarano, F. & Novara, M. 2011 On the velocity of ghost particles and the bias errors in Tomographic-PIV. Exp. Fluids 50 (4), 825–838.Google Scholar
Gallaire, F. & Chomaz, J. M. 2003 Instability mechanisms in swirling flows. Phys. Fluids 15 (9), 2622–2639.Google Scholar
Grundmann, S., Wassermann, F., Lorenz, R., Jung, B. & Tropea, C. 2012 Experimental investigation of helical structures in swirling flows. Intl J. Heat Fluid Flow 37, 51–63.10.1016/j.ijheatfluidflow.2012.05.003Google Scholar
Gupta, A. K., Lilley, D. G. & Syred, N. 1984 Swirl Flows. Abacus Press.Google Scholar
Harvey, J. K. 1962 Some observations of the vortex breakdown phenomenon. J. Fluid Mech. 14 (4), 585–592.10.1017/S0022112062001470Google Scholar
Herman, G. T. & Lent, A. 1976 Iterative reconstruction algorithms. Comput. Biol. Med. 6 (4), 273–294.Google Scholar
Holmes, P., Lumley, J. L., Berkooz, G. & Rowley, C. W. 2012 Turbulence, Coherent Structures, Dynamical Systems and Symmetry, 2nd edn. Cambridge University Press.Google Scholar
Hunt, J. C., Wray, A. A. & Moin, P.1988 Eddies, streams, and convergence zones in turbulent flows.Google Scholar
Ianiro, A. & Cardone, G. 2012 Heat transfer rate and uniformity in multichannel swirling impinging jets. Appl. Therm. Engng 49, 89–98.10.1016/j.applthermaleng.2011.10.018Google Scholar
Jakirlic, S., Hanjalic, K. & Tropea, C. 2002 Modeling rotating and swirling turbulent flows: a perpetual challenge. AIAA J. 40 (10), 1984–1996.10.2514/2.1560Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Jovanović, M. R., Schmid, P. J. & Nichols, J. W. 2014 Sparsity-promoting dynamic mode decomposition. Phys. Fluids 26 (2), 024103.Google Scholar
Kähler, C. J., Astarita, T., Vlachos, P. P., Sakakibara, J., Hain, R., Discetti, S., La Foy, R. & Cierpka, C. 2016 Main results of the 4th International PIV Challenge. Exp. Fluids 57, 97.Google Scholar
Kelvin, W. 1871 Hydrokinetic solutions and observations. Phil. Mag. 42, 362–377.Google Scholar
Kurosaka, M., Cain, C. B., Srigrarom, S., Wimer, J. D., Dabiri, D., Johnson, W. F., Hatcher, J. C., Thompson, B. R., Kikuchi, M., Hirano, K., Yugé, T. & Honda, T. 2006 Azimuthal vorticity gradient in the formative stages of vortex breakdown. J. Fluid Mech. 569, 1–28.10.1017/S0022112006001911Google Scholar
Lambourne, N. & Bryer, D. W. 1962 The bursting of leading-edge vortices: some observations and discussion of the phenomenon. Aero. Res. Counc. R&M 36, 3282.Google Scholar
Leibovich, S. 1984 Vortex stability and breakdown: survey and extension. AIAA J. 22 (9), 1192–1206.Google Scholar
Liang, H. & Maxworthy, T. 2005 An experimental investigation of swirling jets. J. Fluid Mech. 525, 115–159.10.1017/S0022112004002629Google Scholar
Lilley, D. G. 1977 Swirl flows in combustion: a review. AIAA J. 15 (8), 1063–1078.Google Scholar
Lucca-Negro, O. & O’Doherty, T. 2001 Vortex breakdown: a review. Prog. Energy Combust. Sci. 27 (4), 431–481.10.1016/S0360-1285(00)00022-8Google Scholar
Lynch, K. & Scarano, F. 2013 A high-order time-accurate interrogation method for time-resolved PIV. Meas. Sci. Technol. 24 (3), 035305.Google Scholar
Lynch, K. P. & Scarano, F. 2014 Experimental determination of tomographic PIV accuracy by a 12-camera system. Meas. Sci. Technol. 25 (8), 084003.10.1088/0957-0233/25/8/084003Google Scholar
Lynch, K. P. & Scarano, F. 2015 An efficient and accurate approach to MTE-MART for time-resolved tomographic PIV. Exp. Fluids 56 (3), 1–16.10.1007/s00348-015-1934-6Google Scholar
Majda, A. J. & Bertozzi, A. L. 2002 Vorticity and Incompressible Flow, vol. 27. Cambridge University Press.Google Scholar
Markovich, D. M., Abdurakipov, S. S., Chikishev, L. M., Dulin, V. M. & Hanjalić, K. 2014 Comparative analysis of low- and high-swirl confined flames and jets by proper orthogonal and dynamic mode decompositions. Phys. Fluids 26 (6), 065109.10.1063/1.4884915Google Scholar
Martinelli, F., Olivani, A. & Coghe, A. 2007 Experimental analysis of the precessing vortex core in a free swirling jet. Exp. Fluids 42 (6), 827–839.10.1007/s00348-006-0230-xGoogle Scholar
Moffat, R. J. 1988 Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1 (1), 3–17.Google Scholar
Novara, M., Batenburg, K. J. & Scarano, F. 2010 Motion tracking-enhanced MART for tomographic PIV. Meas. Sci. Technol. 21 (3), 035401.Google Scholar
Örlü, R. & Alfredsson, P. H. 2008 An experimental study of the near-field mixing characteristics of a swirling jet. Flow Turbul. Combust. 80 (3), 323–350.10.1007/s10494-007-9126-yGoogle Scholar
Oberleithner, K., Sieber, M., Nayeri, C. N., Paschereit, C. O., Petz, C., Hege, H. C. & Wygnanski, I. 2011 Three-dimensional coherent structures in a swirling jet undergoing vortex breakdown: stability analysis and empirical mode construction. J. Fluid Mech. 679, 383–414.Google Scholar
Panda, J. & McLaughlin, D. K. 1994 Experiments on the instabilities of a swirling jet. Phys. Fluids 6 (1), 263–276.Google Scholar
Park, S. H. & Shin, H. D. 1993 Measurements of entrainment characteristics of swirling jets. Intl J. Heat Mass Transfer 36 (16), 4009–4018.10.1016/0017-9310(93)90151-UGoogle Scholar
Rajaratnam, N. 1976 Turbulent Jets, vol. 5. Elsevier.Google Scholar
Rayleigh, Lord 1917 On the dynamics of revolving fluids. Proc. R. Soc. Lond. A 93 (648), 148–154.Google Scholar
Riley, N. & Stevens, D. P. 1993 A note on leapfrogging vortex rings. Fluid Dyn. Res. 11 (5), 235–244.Google Scholar
Rose, W. G. 1962 A swirling round turbulent jet. Part 1. Mean-flow measurements. J. Appl. Mech. 29 (4), 615–625.Google Scholar
Rotunno, R. 2013 The fluid dynamics of tornadoes. Annu. Rev. Fluid Mech. 45 (1), 59.Google Scholar
Sarpkaya, T. 1971 On stationary and travelling vortex breakdowns. J. Fluid Mech. 45 (3), 545–559.Google Scholar
Scarano, F. 2012 Tomographic PIV: principles and practice. Meas. Sci. Technol. 24 (1), 012001.Google Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.10.1017/S0022112010001217Google Scholar
Schmid, P. J., Violato, D. & Scarano, F. 2012 Decomposition of time-resolved tomographic PIV. Exp. Fluids 52 (6), 1567–1579.10.1007/s00348-012-1266-8Google Scholar
Shanbhogue, S. J., Husain, S. & Lieuwen, T. 2009 Lean blowoff of bluff body stabilized flames: scaling and dynamics. Prog. Energy Combust. Sci. 35 (1), 98–120.Google Scholar
Syred, N. & Beér, J. M. 1974 Combustion in swirling flows: a review. Combust. Flame 23 (2), 143–201.Google Scholar
Syred, N. 2006 A review of oscillation mechanisms and the role of the precessing vortex core (PVC) in swirl combustion systems. Prog. Energy Combust. Sci. 32 (2), 93–161.Google Scholar
Toh, K., Honnery, D. & Soria, J. 2010 Axial plus tangential entry swirling jet. Exp. Fluids 48 (2), 309–325.10.1007/s00348-009-0734-2Google Scholar
Torricelli, E.(1643) De motu gravium naturaliter accelerato. Florence.Google Scholar
Tu, J. H., Rowley, C. W., Luchtenburg, D. M., Brunton, S. L. & Kutz, J. N. 2014 On dynamic mode decomposition: theory and applications. J. Comput. Dyn. 1 (2), 391–421.10.3934/jcd.2014.1.391Google Scholar
Vanierschot, M. & Van den Bulck, E. 2008 Influence of swirl on the initial merging zone of a turbulent annular jet. Phys. Fluids 20 (10), 105104.Google Scholar
Violato, D. & Scarano, F. 2011 Three-dimensional evolution of flow structures in transitional circular and chevron jets. Phys. Fluids 23 (12), 124104.Google Scholar
Violato, D., Ianiro, A., Cardone, G. & Scarano, F. 2012 Three-dimensional vortex dynamics and convective heat transfer in circular and chevron impinging jets. Intl J. Heat Fluid Flow 37, 22–36.Google Scholar
Wieneke, B. 2008 Volume self-calibration for 3D particle image velocimetry. Exp. Fluids 45 (4), 549–556.10.1007/s00348-008-0521-5Google Scholar
Winant, C. D. & Browand, F. K. 1974 Vortex pairing: the mechanism of turbulent mixing-layer growth at moderate Reynolds number. J. Fluid Mech. 63 (2), 237–255.10.1017/S0022112074001121Google Scholar
Yazdabadi, P. A., Griffiths, A. J. & Syred, N. 1994 Characterization of the PVC phenomena in the exhaust of a cyclone dust separator. Exp. Fluids 17 (1–2), 84–95.Google Scholar
Yule, A. J. 1978 Large-scale structure in the mixing layer of a round jet. J. Fluid Mech. 89 (3), 413–432.Google Scholar
Zhang, J., Tao, B. & Katz, J. 1997 Turbulent flow measurement in a square duct with hybrid holographic PIV. Exp. Fluids 23 (5), 373–381.Google Scholar

Ianiro et al. suplementary movie 1

Animation of the S=0.2 jet with vortex visualization

Download Ianiro et al. suplementary movie 1(Video)
Video 9.7 MB

Ianiro et al. suplementary movie 2

Animation of the S=0.4 jet with vortex visualization

Download Ianiro et al. suplementary movie 2(Video)
Video 9.2 MB

Ianiro et al. suplementary movie 3

Animation of the S=0.6 jet with vortex visualization

Download Ianiro et al. suplementary movie 3(Video)
Video 10.4 MB