Article contents
Theoretical and numerical studies on dissolution of horizontal salt body and pattern formation incorporated with a dynamic moving interface
Published online by Cambridge University Press: 30 March 2023
Abstract
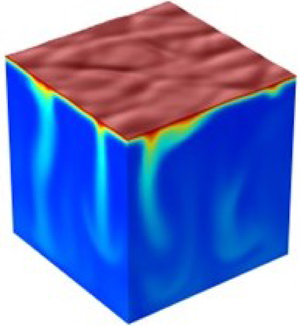
The dissolution of a horizontal salt body is theoretically and numerically investigated by considering the coupled dynamics of the dissolution reaction and the diffusive and convective mass transfer at the salt surface. To take into account the nature of the recessing salt surface and the dissolution-driven convection, a dynamic moving boundary condition coupled with a dissolution reaction are employed By employing a newly derived moving interface condition and parameters, a theoretical analysis predicts the onset of gravitational instability, suggesting scaling relationships between parameters to describe the onset of instability (in transport-dominant (Damkholer number  $Da \to \infty $ and Rayleigh number
$Da \to \infty $ and Rayleigh number  $Ra > {10^5}$), onset time
$Ra > {10^5}$), onset time  ${\tau _d}\sim R{a^{ - 2/3}}$ and
${\tau _d}\sim R{a^{ - 2/3}}$ and  ${\tau _d}\sim (RaDa)^{1/4}$ for reaction-dominant regimes
${\tau _d}\sim (RaDa)^{1/4}$ for reaction-dominant regimes  $(Da \to 0)$). The scaling relationships on dissolution (the average height change,
$(Da \to 0)$). The scaling relationships on dissolution (the average height change,  $\Delta {h_{avg}}\sim \sqrt \tau $ for the diffusion-dominant regime and
$\Delta {h_{avg}}\sim \sqrt \tau $ for the diffusion-dominant regime and  $\Delta {h_{avg}}\sim R{a^{0.79/3}}{\tau ^{0.86}}$ for the convection-dominant regime) are also suggested to explain the pattern formation. Under a convective mass transfer condition, the concentration gradient of the solute developed during the recession of the salt surface results in an adverse density gradient, which causes gravitational instability motion and finally determines the formation of a dissolution pattern. In addition, two-dimensional and three-dimensional numerical simulations visualize dissolution-driven convective flow fields, the recession of the liquid-solid interface and pattern formation on the dissolving interface. Theoretical and numerical analyses of the dissolution process suggest that the control of gravitational instability is important to prevent or enhance dissolution pattern formation. This systematic parameter study provides a deep understanding of the effect of gravitational instability on convection-driven dissolution in a horizontal geometry.
$\Delta {h_{avg}}\sim R{a^{0.79/3}}{\tau ^{0.86}}$ for the convection-dominant regime) are also suggested to explain the pattern formation. Under a convective mass transfer condition, the concentration gradient of the solute developed during the recession of the salt surface results in an adverse density gradient, which causes gravitational instability motion and finally determines the formation of a dissolution pattern. In addition, two-dimensional and three-dimensional numerical simulations visualize dissolution-driven convective flow fields, the recession of the liquid-solid interface and pattern formation on the dissolving interface. Theoretical and numerical analyses of the dissolution process suggest that the control of gravitational instability is important to prevent or enhance dissolution pattern formation. This systematic parameter study provides a deep understanding of the effect of gravitational instability on convection-driven dissolution in a horizontal geometry.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 4
- Cited by



