Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-30T19:30:12.507Z Has data issue: false hasContentIssue false
  • English
  • Français

Surface pressure fluctuations on steps immersed in turbulent boundary layers

Published online by Cambridge University Press:  01 October 2012

Minsuk Ji  and
Meng Wang
Minsuk Ji*
Affiliation:
Institute for Flow Physics and Control, Department of Aerospace and Mechanical Engineering, University of Notre Dame, Notre Dame, IN 46556, USA
Meng Wang
Affiliation:
Institute for Flow Physics and Control, Department of Aerospace and Mechanical Engineering, University of Notre Dame, Notre Dame, IN 46556, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Surface pressure fluctuations induced by turbulent boundary-layer flow at 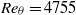${\mathit{Re}}_{\theta } = 4755$ over small backward- and forward-facing steps are studied with large-eddy simulation. Four step heights that are 53, 13, 3.3 and 0.83 % of the boundary-layer thickness are considered to investigate the effects of step height on surface pressure characteristics and pressure-source mechanisms. The extent to which turbulent velocity fluctuations in the boundary layer and the separated shear layer contribute to the surface pressure fluctuations is examined with scaling of various pressure statistics and two-point correlations. For larger steps, vortical structures develop in the shear layer and the associated intense velocity fluctuations are the dominant source. Downstream of slightly less than one reattachment length from the step, the root-mean-square pressure is found to scale with the local maximum cross-stream Reynolds normal stress 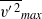${ \overline{{v}^{\ensuremath{\prime} \hspace{0.167em} 2} } }_{\mathit{max}} $. The pressure frequency spectrum at the maximum 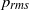${p}_{\mathit{rms}} $ location consists of an energy-containing range that scales with the mean reattachment length 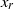${x}_{r} $ and a higher frequency range that rolls off with a slope close to 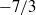$\ensuremath{-} 7/ 3$. As the step height decreases, the boundary-layer turbulent fluctuations become the dominant source, the 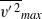${ \overline{{v}^{\ensuremath{\prime} \hspace{0.167em} 2} } }_{\mathit{max}} $ scaling of 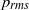${p}_{\mathit{rms}} $ is no longer valid and the roll-off slope of the frequency spectrum becomes steeper. The downstream recovery of a step-perturbed boundary layer towards an equilibrium boundary layer is investigated from the point of view of surface pressure fluctuations. For steps with a strong separated shear layer, pressure fluctuations are found to decay rapidly for up to three reattachment lengths downstream of the step, within which approximately 60 % of the peak 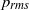${p}_{\mathit{rms}} $ is dissipated. Farther downstream, recovery is much slower. The pressure-recovery distances estimated for the largest backward and forward steps are 175 and 295 step heights, respectively.

JFM classification

Acoustics: Hydrodynamic noise Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 712 , 10 December 2012 , pp. 471 - 504
Copyright
©2012 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alving, A. E. & Fernholz, H. H. 1996 Turbulence measurements around a mild separation bubble and downstream of reattachment. J. Fluid Mech. 322, 297–328.CrossRefGoogle Scholar
Awasthi, M., Forest, J. B., Morton, M. A., Devenport, W. & Glegg, S. A. L. 2011 The disturbance of a high Reynolds number turbulent boundary layer by small forward steps. AIAA Paper 2011-2777.Google Scholar
Bendat, J. S. & Piersol, A. G. 2000 Random Data: Analysis and Measurement Procedures, 3rd edn. Wiley-Interscience.Google Scholar
Bradshaw, P. & Wong, F. Y. F. 1972 The reattachment and relaxation of a turbulent shear layer. J. Fluid Mech. 52, 113–135.CrossRefGoogle Scholar
Brungart, T. A., Lauchle, G. C., Deutsch, S. & Riggs, E. T. 2002 Wall pressure fluctuations induced by separated/reattached channel flow. J. Sound Vib. 251, 558–577.Google Scholar
Camussi, R., Felli, M., Pereira, F., Aloisio, G. & Di Marco, A. 2008 Statistical properties of wall pressure fluctuations over a forward-facing step. Phys. Fluids 20, 075113.Google Scholar
Camussi, R., Guj, G. & Ragni, A. 2006 Wall pressure fluctuations induced by turbulent boundary layers over surface discontinuities. J. Sound Vib. 294, 177–204.Google Scholar
Castro, I. P. & Epik, E. 1998 Boundary layer development after a separated region. J. Fluid Mech. 374, 91–116.Google Scholar
Chun, S., Liu, Y. Z. & Sung, H. J. 2004 Wall pressure fluctuations of a turbulent separated and reattaching flow affected by an unsteady wake. Exp. Fluids 37, 531–546.Google Scholar
Dandois, J., Garnier, E. & Sagaut, P. 2007 Numerical simulation of active separation control by a synthetic jet. J. Fluid Mech. 574, 25–58.Google Scholar
Eaton, J. K. & Johnston, J. P. 1981 A review of research on subsonic turbulent flow reattachment. AIAA J. 19, 1093–1100.Google Scholar
Efimtsov, B. M., Kozlov, N. M., Kravchenko, S. V. & Andersson, A. O. 1999 Wall pressure fluctuation spectra at small forward facing steps. AIAA Paper 99-1964.Google Scholar
Efimtsov, B. M., Kozlov, N. M., Kravchenko, S. V. & Andersson, A. O. 2000 Wall pressure fluctuation spectra at small backward facing steps. AIAA Paper 2000-2053.Google Scholar
Farabee, T. M. & Casarella, M. J. 1984 Effects of surface irregularity on turbulent boundary layer wall pressure fluctuations. Trans. ASME: J. Vib., Acoust, Stress, Reliab. Design 106, 343–350.Google Scholar
Farabee, T. M. & Casarella, M. J. 1986 Measurements of fluctuating wall pressure for separated/reattached boundary layer flows. Trans. ASME: J. Vib., Acoust, Stress, Reliab. Design 108, 301–307.Google Scholar
Fricke, F. R. 1971 Pressure fluctuations in separated flows. J. Sound Vib. 17, 113–123.Google Scholar
George, W. K., Beuther, P. D. & Arndt, R. E. A. 1984 Pressure spectra in turbulent free shear flows. J. Fluid Mech. 148, 155–191.Google Scholar
Heenan, A. F. & Morrison, J. F. 1998 Passive control of pressure fluctuations generated by separated flow. AIAA J. 36, 1014–1022.CrossRefGoogle Scholar
Hudy, L. M., Naguib, A. M. & Humphreys, W. M. 2003 Wall-pressure-array measurements beneath a separating/reattaching flow region. Phys. Fluids 15, 706–717.Google Scholar
Ji, M. & Wang, M. 2010 Sound generation by turbulent boundary-layer flow over small steps. J. Fluid Mech. 654, 161–193.Google Scholar
Kiya, M. & Sasaki, K. 1983 Structure of a turbulent separation bubble. J. Fluid Mech. 137, 83–113.Google Scholar
Kraichnan, R. H. 1956 Pressure fluctuations in turbulent flow over a flat plate. J. Acoust. Soc. Am. 28, 378–390.Google Scholar
Largeau, J. F. & Moriniere, V. 2007 Wall pressure fluctuations and topology in separated flows over a forward-facing step. Exp. Fluids 42, 21–40.Google Scholar
Lauchle, G. C. & Kargus, W. A. IV 2000 Scaling of turbulent wall pressure fluctuations downstream of a rearward facing step. J. Acoust. Soc. Am. 107, L1–L6.CrossRefGoogle ScholarPubMed
Leclercq, D. J. J., Jacob, M. C., Louisot, A. & Talotte, C. 2001 Forward–backward facing step pair: aerodynamic flow, wall pressure and acoustic characterization. AIAA Paper 2001-2249.Google Scholar
Lee, I. & Sung, H. J. 2001 Characteristics of wall pressure fluctuations in separated and reattaching flows over a backward-facing step. Part I. Time-mean statistics and cross-spectral analyses. Exp. Fluids 30, 262–272.CrossRefGoogle Scholar
Lee, I. & Sung, H. J. 2002 Multiple-arrayed pressure measurement for investigation of the unsteady flow structure of a reattaching shear layer. J. Fluid Mech. 463, 377–402.Google Scholar
Lund, T. S., Wu, X. & Squires, K. D. 1998 Generation of turbulent inflow data for spatially-developing boundary layer simulations. J. Comput. Phys. 140, 233–258.Google Scholar
Mabey, D. G. 1972 Analysis and correlation of data on pressure fluctuations in separated flow. J. Aircraft 9, 642–645.CrossRefGoogle Scholar
Mabey, D. G. 1982 Comment on ‘A review of research on subsonic turbulent flow attachment’. AIAA J. 20, 1632.Google Scholar
Mohsen, A. M. 1968 Experimental investigation of the wall pressure fluctuations in subsonic separated flows. Tech. Rep. D6-17094. Boeing Company.CrossRefGoogle Scholar
Moss, W. D. & Baker, S. 1980 Re-circulating flows associated with two-dimensional steps. Aeronaut. Q. 31, 151–172.Google Scholar
Na, Y. & Moin, P. 1998a Direct numerical simulation of a separated turbulent boundary layer. J. Fluid Mech. 374, 379–405.Google Scholar
Na, Y. & Moin, P. 1998b The structure of wall-pressure fluctuations in turbulent boundary layers with adverse pressure gradient and separation. J. Fluid Mech. 377, 347–373.CrossRefGoogle Scholar
Panton, R. L. & Linebarger, J. H. 1974 Wall pressure spectra calculations for equilibrium boundary layers. J. Fluid Mech. 65, 261–287.Google Scholar
Sigurdson, L. W. 1995 The structure and control of a turbulent reattaching flow. J. Fluid Mech. 298, 139–165.CrossRefGoogle Scholar
Simpson, R. L. 1989 Turbulent boundary-layer separation. Annu. Rev. Fluid Mech. 21, 205–234.Google Scholar
Simpson, R. L., Chew, Y.-T. & Shivaprasad, B. G. 1981 The structure of a separating turbulent boundary layer. Part 1. Mean flow and Reynolds stresses. J. Fluid Mech. 113, 23–51.Google Scholar
Simpson, R. L., Ghodbane, M. & McGrath, B. E. 1987 Surface pressure fluctuations in a separating turbulent boundary layer. J. Fluid Mech. 177, 167–186.CrossRefGoogle Scholar
Song, S., DeGraaff, D. B. & Eaton, J. K. 2000 Experimental study of a separating, reattaching, and redeveloping flow over a smoothly contoured ramp. Intl J. Heat Fluid Flow 21, 512–519.CrossRefGoogle Scholar
Tachie, M. F., Balachandar, R. & Bergstrom, D. J. 2001 Open channel boundary layer relaxation behind a forward facing step at low Reynolds numbers. Trans. ASME: J. Fluids Engng 123, 539–544.Google Scholar
You, D., Mittal, R., Wang, M. & Moin, P. 2004 Computational methodology for large-eddy simulation of tip-clearance flows. AIAA J. 42, 271–279.Google Scholar